How Much Marsh Restoration Is Enough to Deliver Wave Attenuation Coastal Protection Benefits?

As coastal communities grow more vulnerable to sea-level rise and increased storminess, communities have turned to nature-based solutions to bolster coastal resilience and protection. Marshes have significant wave attenuation properties and can play an important role in coastal protection for many communities. Many restoration projects seek to maximize this ecosystem service but how much marsh restoration is enough to deliver measurable coastal protection benefits is still unknown. This question is critical to guiding assessments of cost effectiveness and for funding, implementation, and optimizing of marsh restoration for risk reduction projects. This study uses SWAN model simulations to determine empirical relationships between wave attenuation and marsh vegetation. The model runs consider several different common marsh morphologies (including systems with channels, ponds, and fringing mudflats), vegetation placement, and simulated storm intensity. Up to a 95% reduction in wave energy is seen at as low as 50% vegetation cover. Although these empirical relationships between vegetative cover and wave attenuation provide essential insight for marsh restoration, it is also important to factor in lifespan estimates of restored marshes when making overall restoration decisions. The results of this study are important for coastal practitioners and managers seeking performance goals and metrics for marsh restoration, enhancement, and creation.

Introduction

Communities have turned to nature-based solutions to bolster coastal resilience and protection in the face of rising sea levels and increasing storminess. Marshes have significant wave attenuation properties and can play an important role in coastal protection for many communities (Gedan et al., 2011; Temmerman et al., 2013). Previous field, lab, and modeling research has documented the ability of marsh vegetation to reduce the energy and height of storm waves. Despite both making landfall in southern Louisiana in 2005 as category 3 hurricanes, Hurricane Rita was less deadly than Hurricane Katrina, largely because Rita traveled over at least 30 km of wetland before reaching a major populated center (Day et al., 2007). A flume experiment by Möller et al. (2014) using natural marsh found that up to 60% of observed wave height reduction was due to the presence of vegetation. This builds on the meta-analysis of Shepard et al. (2011), which found that marsh vegetation attenuates smaller, more frequent waves and plays a significant role in shoreline stabilization. Wamsley et al. (2009) modeled Hurricanes Katrina and Rita on southern Louisiana marshes and found that restoration decreased storm wave heights, though the amount of attenuation was variable among different marshes.

Many restoration projects seek to maximize the ecosystem service of wave attenuation, but how much marsh restoration is enough to deliver benefits remains unclear. Salt marsh restoration projects often employ a combination of techniques, including increasing tidal flow through removal of existing restrictions (Burdick and Roman, 2012), increasing marsh elevation (through natural sedimentation associated with increased flow or augmented sedimentation such as thin-layer deposition; U.S. Army Corps of Engineers, 2022), and increasing vegetation cover through physical planting of appropriate native species (Sparks et al., 2013). While wave attenuation is often cited as an important benefit to marsh restoration, and studies have quantified wave attenuation in the field (Möller and Spencer, 2002; Lightbody and Nepf, 2006; Jadhav et al., 2013), lab (Möller et al., 2014; Rupprecht et al., 2017), and in model settings (Loder et al., 2009; Wu et al., 2016; Marsooli et al., 2017), wave energy reduction as a function of marsh restoration is less studied.

Reduction in wave height and subsequent wave energy has cost-effective implications for coastal resilience; reduction of wave energy, in turn, reduces wave-driven flooding. Salt marshes and mangrove systems have been found to be two to five times less expensive than submerged breakwaters for wave heights up to 0.5 m (Narayan et al., 2016). Narayan et al. (2017) found that during Hurricane Sandy in 2012, wetlands in the northeastern United States avoided more than $600 million in direct flood damage. Management decisions, however, consider effort and cost trade-offs in marsh restoration scenarios. Sparks et al. (2013) found that small-scale experimental Juncus roemerianus plots planted at half density were largely more or equally cost-effective for both planting cost and effort when compared to plots planted at full density. Smith et al. (2018) analyzed the resistance of various types of shorelines in North Carolina, United States, to Hurricane Matthew (2016), and found that constructed/planted salt marshes with an offshore rock sill were more resistant to the hurricane’s impacts than both traditional hardened shorelines and natural marshes. Given this context, research into wave attenuation as a function of marsh vegetation and, indirectly, marsh restoration, provides valuable insight critical to guiding efforts to optimize funding, effort, and overall cost effectiveness of restoration projects.

This study uses a series of model simulations to determine empirical relationships among wave attenuation, percent vegetated, and marsh unvegetated-vegetated ratio (UVVR). The empirical relationships presented in this study span a variety of characteristics (including vegetated/unvegetated ratio, vegetation distribution, storm intensity, and general marsh morphology) and can be applied by restoration practitioners to determine appropriate restoration planting targets to maximize wave attenuation.

Materials and Methods

Model Setup

This study uses a third-generation directional spectral wave model, SWAN (Simulating Waves Nearshore; Booij et al., 1999), to simulate wave attenuation by salt marsh vegetation across a series of different idealized morphologies (Figure 1 and Supplementary Table 1), generalized and based on common field analogs (Supplementary Figure 1). Wave characteristics were chosen to be representative of low-, medium-, and high-intensity storm events (Table 1). Waves approached the 600 m-by-600 m, 1-m resolution model grid directly from the south and assumed a JONSWAP (JOint NOrth Sea WAve Project) spectral shape (Hasselmann et al., 1973). Model bathymetry spanned from -10 m (offshore) to +2 m, with vegetation beginning at 0 m, approximately ∼160 m from the north edge of the domain (Figure 1J). Model water depth was defined at 2.5 m above 0 m bathymetry, such that the entire marsh surface was inundated, though at depths dependent on bathymetry, representative of a major storm surge or high tide event (Figure 1J). Vegetated cells were defined (Table 1) using values consistent with Bendoni et al. (2019) using the default Dalrymple vegetation module (Dalrymple et al., 1984). The vegetation drag coefficient was chosen to be representative of the synthesis of both Anderson and Smith (2014) and Vuik et al. (2016).

FIGURE 1

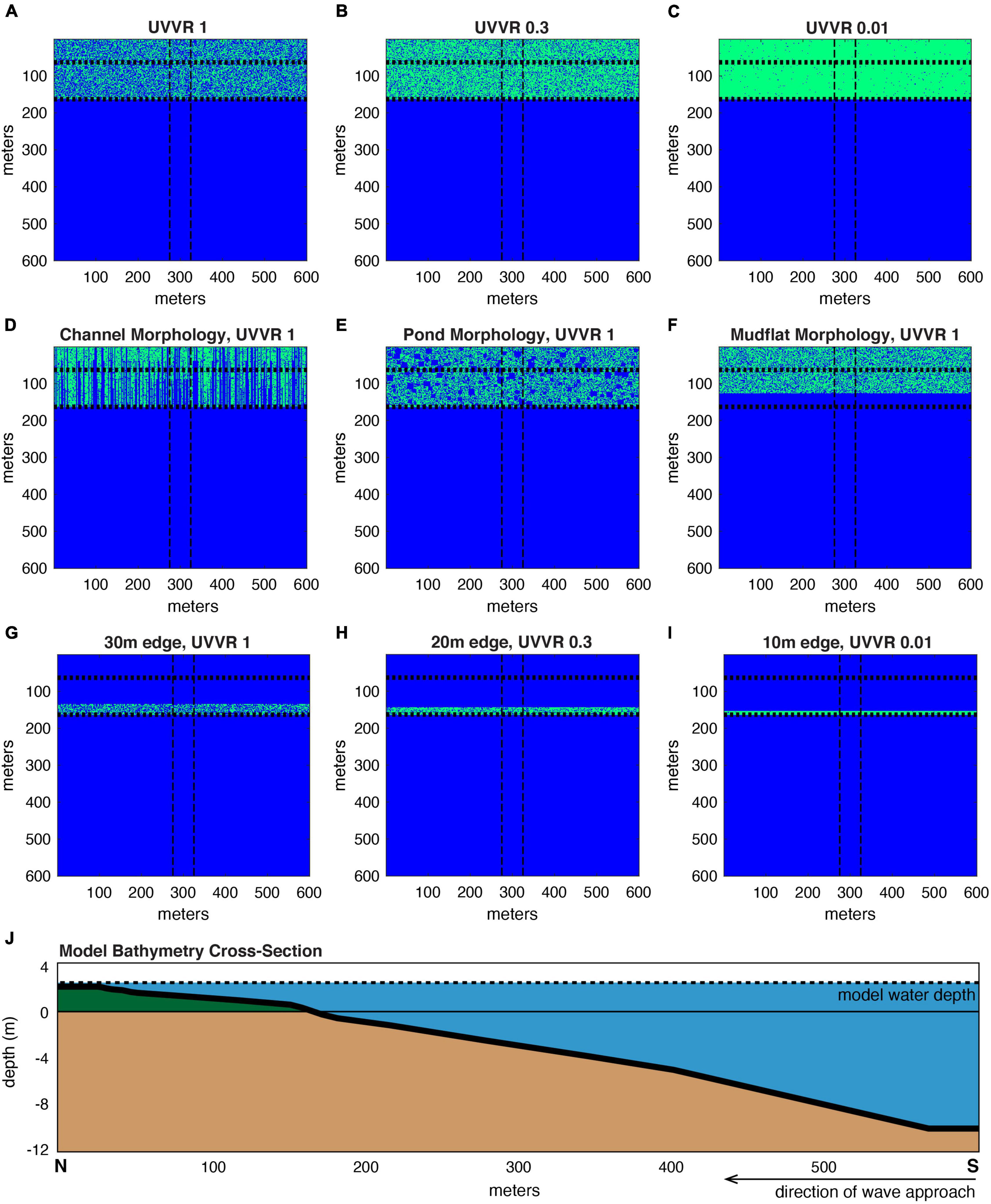
Figure 1. Example 600 by 600 m, 1-m resolution model domains for the following cases: (A–C) comparing randomized vegetation percentages; (D–F) comparing different significant marsh morphologies (channel, pond, and mudflat), and (G–I) varying the width and density of a vegetated leading edge. Vegetated cells are indicated with green. Waves approach directly from the south. Wave energy is averaged horizontally over the middle 50 m, indicated with vertical dashed lines. Percent change in wave energy is determined between the horizontal dotted lines. (J) Cross-section of model input bathymetry, with model water depth indicated by the dotted line. Vegetation for all cases began at 0 m depth; indicated by green.

TABLE 1

Table 1. Model constants.

Here, we present three study cases that explore varying vegetation, morphology, and the presence of a vegetated leading edge. Wave energy was determined from wave height using the equation:

$E=\frac{1}{8}\mathrm{\rho }g{H}^2$

where E represents wave energy in J/m², ρ is the density of water (1024 kg/m³ at 20°C), g is the acceleration of gravity (9.81 m/s²), and H is the wave height. Wave energy was horizontally averaged in the middle 50 m of the domain, and percent change in wave energy was determined over the first 100 m from the model shore. Reduction in wave energy is a function of both vegetation and marsh elevation; model runs with no vegetation indicate a percent reduction in wave energy over the first 100 m of marsh of 52, 81, and 84% for low, medium, and high intensity wave scenarios, respectively.

Vegetation distribution was based on unvegetated/vegetated ratio (UVVR) values. UVVR is a pixel-based indicator of marsh vulnerability related to sediment budget and derived from aerial imagery (Ganju et al., 2016). UVVR is distinct from percent vegetated (a metric often used in marsh literature to represent individual transect or quadrat measurement) in that it can be translated directly to modeling frameworks over a wide variety of spatial scales (Supplementary Figure 1). UVVR has also been used to identify key tipping points in marsh stability (Ganju et al., 2016). Integrating stability (D’Alpaos, 2011; Ganju, 2019; Wasson et al., 2019) into our understanding of marsh restoration is imperative to develop and implement projects that are successful over long timescales. UVVR values are available for entire contiguous United States coastline through the U.S. Geological Survey (Couvillion et al., 2021), but it is important to consider that practitioners can use percent vegetation cover measurements to estimate UVVR, particularly in areas where marsh-restoration projects are rapidly changing. As such, this study employs both vegetation measurement conventions.

Vegetation

To determine a relationship between marsh UVVR, percent vegetation cover, and wave attenuation, marshes were first modeled with randomized vegetated cells throughout the entire domain. Desired percent vegetated cover and subsequent UVVR value was reached through randomly seeding vegetated cells in the model domain where the bathymetry is >0 m (Figures 1A–C). UVVR values ranged from 0.01 (∼99% vegetated) to 2 (33% vegetated). Each 1-m² cell where the bathymetery is >0 m (indicated with green in Figure 1J) was randomly assigned a value of 0 or 1; cells with a value of 1 were vegetated using the vegetation characteristics in Table 1. The UVVR 0.01–2 range functionally represents a marsh, since UVVR values of >2 (equivalent to 67% unvegetated) effectively function as an intertidal flat or estuarine embayment rather than a marsh, as discussed by Ganju et al. (2020). Three randomized configurations were generated per desired UVVR condition. Percent change in wave energy was determined and averaged across each vegetation configuration for each storm intensity scenario.

Morphology

Three idealized marsh morphologies were used in this study to determine the wave attenuation capability of common marsh presentations: channel-dominated, pond-dominated, and fringing-mudflat-adjacent (as seen in Supplementary Figures 1C–E).

For channel morphologies, channels of varying widths (1–3 m) and lengths (25–160 m) were randomly seeded throughout the vegetated model domain (Figure 1D). Channels were given a uniform depth of 2 m, consistent with observed values (Iwasaki et al., 2013). The number of channels and vegetated cells were randomly varied to reach the desired UVVR, and each UVVR condition, ranging from 0.1 to 2, was run in three randomized configurations.

For pond morphologies, ponds of varying areas, ranging from 1 to 225 m² with a uniform depth of 0.3 m (consistent with observations by Spivak et al., 2017), were randomly seeded throughout the vegetated model domain (Figure 1E). As with the channel morphology, the number of ponds and vegetated cells were randomly varied to reach the desired UVVR, and each UVVR condition was run in three randomized configurations.

Mudflats were represented as unvegetated areas at the model shore. Mudflats were held at a constant width, and vegetation was randomly seeded behind the mudflat to reach the desired UVVR (Figure 1F). As with the other morphologies, each UVVR condition was run in three randomized configurations.

Vegetated Leading Edge

To test specific restoration scenarios, the study also explored the influence of a vegetated leading edge on wave attenuation (Supplementary Figures 1D,F). Leading edges of varying widths (10–50 m) were vegetated at varying UVVRs (0.01–2, equivalent to 99–33% vegetated, respectively) at the model shore (where bathymetry > 0 m; Figures 1G–I). The area behind the leading edge was not vegetated. Percent change in wave energy was determined, as with the previous cases, over the first 100 m from model shore.

Lifespan Estimates

Following the technique of Ganju et al. (2016), lifespan of the restored model marsh was estimated using established relationships among UVVR, sediment budget, marsh area, and marsh elevation. The sediment-based lifespan of a marsh system, L_sed, is a function of the total available sediment mass (M_sed) in a vegetated marsh plain, net sediment budget of the marsh system (Q_b), and the total area of the marsh unit (A). M_sed is approximated as the product of the mean elevation above mean sea level (E_m), the vegetated area within a marsh unit (A_veg), and the representative mean dry bulk density of the sediment stored within the marsh plain (ρ_mean; 373 kg m^–3 per Ganju et al., 2016 and Morris et al., 2016). Using approximations of the relationship between Q_b and UVVR per Ganju et al. (2016):

$L{}_{sed}=-(E{}_m\times A{}_{veg}\times \mathrm{\rho }{}_{mean})/(-0.42logUVVR-1.08\times A)$

Following Ganju et al. (2020), present-day marsh unit lifespans can also be estimated under future global mean sea-level (Sweet et al., 2017) through recomputing lifespan to incorporate the excess sediment deficit from three sea-level rise (SLR) scenarios: 0.3, 0.5, and 1.0 m rise by 2100:

$\begin{array}{c}\hfill L{}_{sed,future}=-(E{}_m\times A{}_{veg}\times \mathrm{\rho }{}_{mean})/(-0.42logUVVR-1.08\\ \hfill \times A)-[(SLR{}_{future}-SLR{}_{background})\times \mathrm{\rho }{}_{future}]\times A\end{array}$

where ρ_future represents the dry bulk density of future deposited sediment (159 kg m^–3 per Morris et al., 2016). SLR_future and SLR_background represent downscaled SLR values from Ganju et al. (2020) for several United States marshes (Chincoteague Bay, Great South Bay, Cape Cod, and Plum Island Estuary) were averaged to apply to model space. Background and 0.3, 0.5, and 1.0 m SLR by 2100 scenarios averaged 0.001, 0.005, 0.006, and 0.013 m/year, respectively.

Results

Vegetation

For randomized vegetation cases, percent change in wave energy over the first 100 m of marsh followed a linear regression (Figure 2A). Wave energy decreased with UVVR value. The percent decrease in wave energy across all wave scenarios was substantial; there was a 95% decrease in wave energy over the first 100 m of the marsh for all wave scenarios by UVVR 1.2 (45% vegetated). Slope, intercept, and statistical values for the linear regressions can be found in Table 2.

FIGURE 2

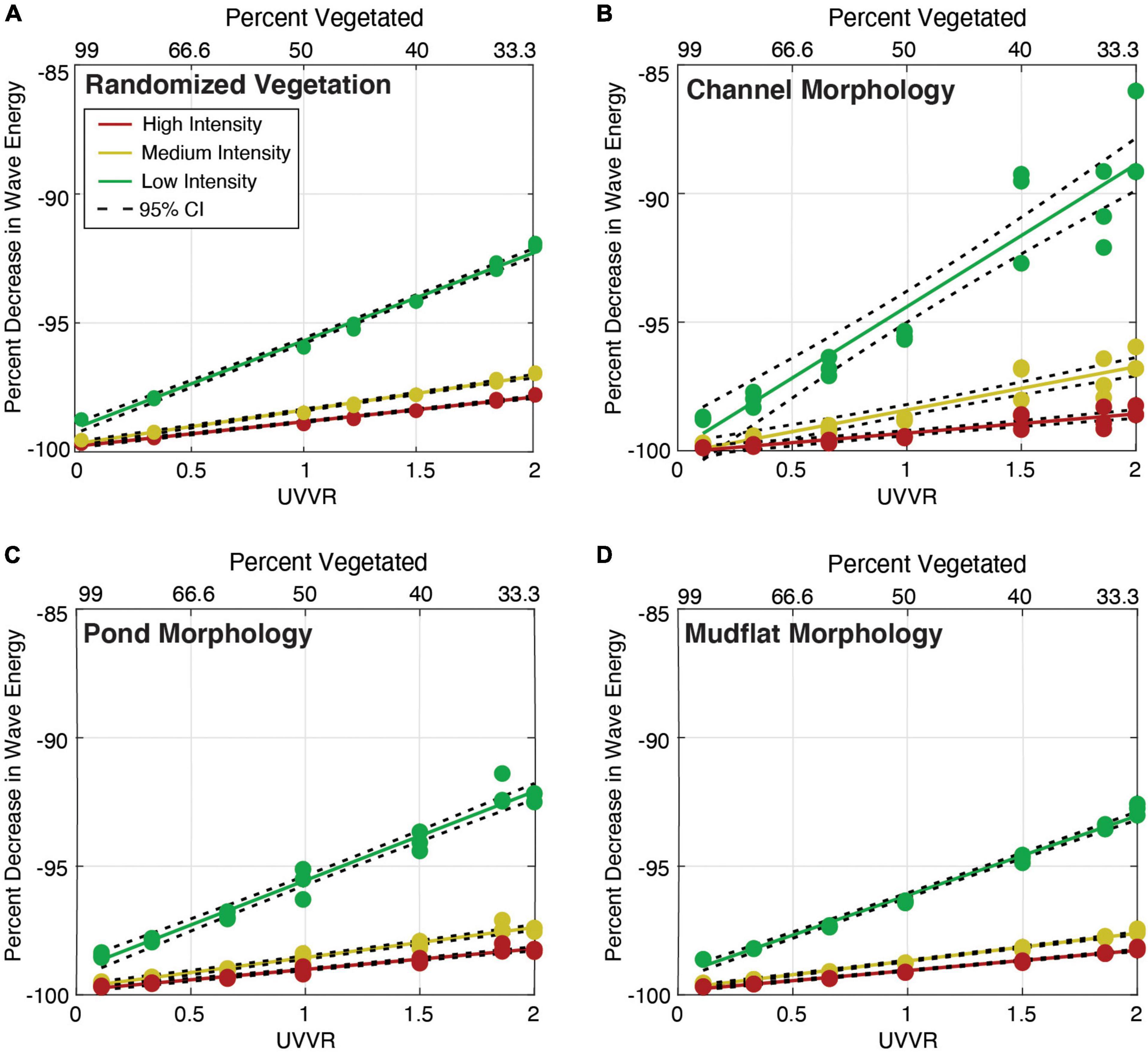
Figure 2. Percent change in wave energy over the first 100 m of marsh for (A) randomized vegetation, (B) channel morphology, (C) pond morphology, and (D) mudflat morphology. Wave intensity scenario is indicated by color, with high-intensity waves (5 m,12 s) in red, medium-intensity waves (2 m, 8 s) in yellow, and low-intensity waves (1 m, 6 s) in green. Model runs with no vegetation and no topography change indicate a percent reduction in wave energy over the first 100 m of marsh of 52, 81, and 84% for low, medium, and high intensity wave scenarios, respectively.

TABLE 2

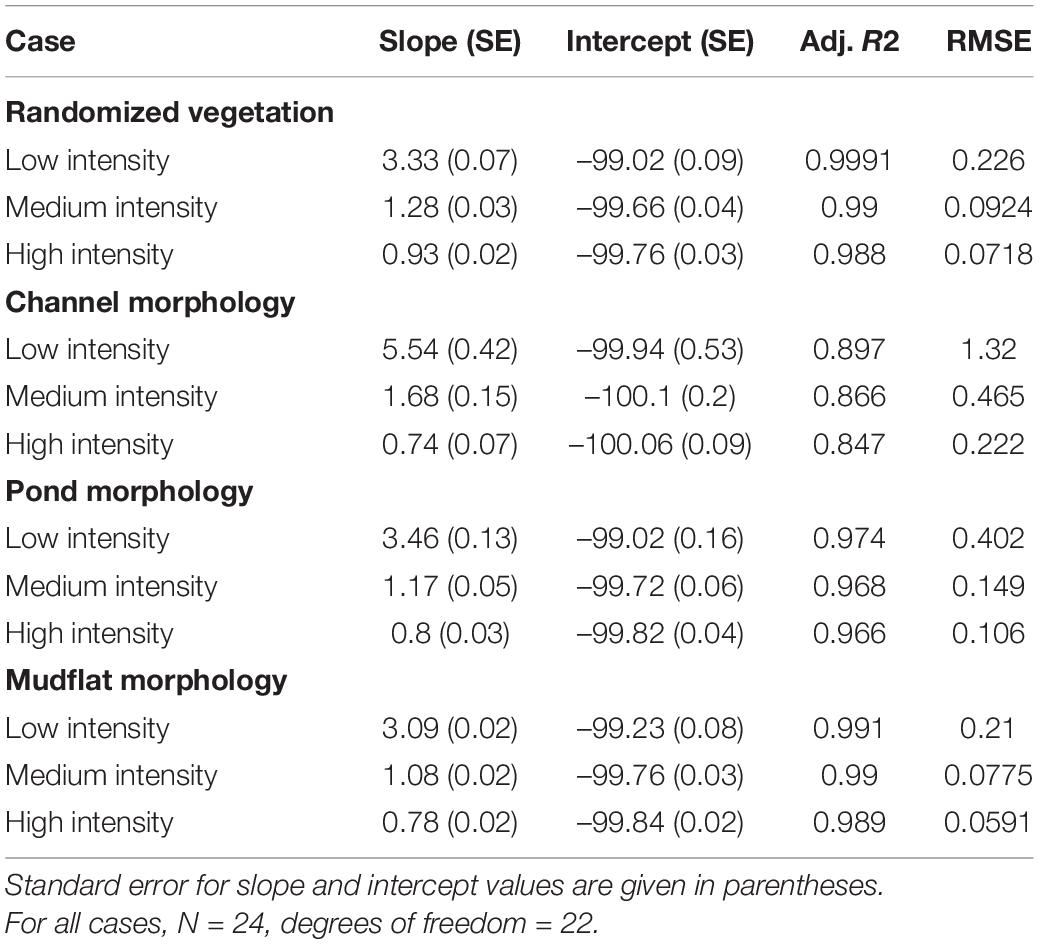
Table 2. Values for linear regressions in the form of y = mx + b, where y represents percent change in wave energy and x represents unvegetated/vegetated ratio (UVVR).

Percent decrease in wave energy was largely influenced by wave intensity (Figure 2A). For low-intensity wave conditions, average percent decrease in wave energy ranged from 91.9% at UVVR 2 (33% vegetated) to 98.7% at UVVR 0.1 (90% vegetated). For medium-intensity wave conditions, average percent decrease in wave energy ranged from 96.9% at UVVR 2 to 99.5% at UVVR 0.1. For high-intensity wave conditions, average percent decrease in wave energy ranged from 97.8% at UVVR 2 to 99.7% at UVVR 0.1.

Wave energy also decreased with changes in elevation and associated wave breaking; model runs with no vegetation and no topography change indicate a percent reduction in wave energy over the first 100 m of marsh of 52, 81, and 84% for low, medium, and high intensity wave scenarios, respectively, suggesting that vegetation alone is responsible for the additional 16–48% reduction in wave energy in high-vegetation scenarios.

Morphology

Percent change in wave energy over the first 100 m of marsh also followed a pattern of linear regression for all morphology types and wave-intensity scenarios (Figures 2B–D); equation constants can be found in Table 2. As with the vegetation simulations, there was a 95% decrease in wave energy over the first 100 m of the marsh for all morphology types and wave scenarios by UVVR 1 (50% vegetated). Channel morphology had the most variation between model runs, particularly under low-intensity wave scenarios; a 95% reduction in wave energy was reached by UVVR 1 in the low-intensity scenario, whereas 96.7 and 98.6% reductions were reached by UVVR 2 under medium- and high-intensity conditions, respectively (Figure 2B). For pond and mudflat morphologies, a 95% reduction in wave energy was reached by UVVR 1, >2 and >2 under low-, medium-, and high-intensity wave conditions, respectively (Figures 2C,D). For context, at UVVR 1 under the low-intensity wave scenario, initial wave heights in the model of 0.9 m are reduced to less than 0.2 m within the first 100 m of marsh. In the medium-intensity wave scenario, 1.6 m model wave heights are reduced to ∼0.3 m, and in the high-intensity wave scenario, 1.9 m model wave heights are reduced to ∼0.4 m.

Vegetated Leading Edge

Percent wave energy reduction increased as leading-edge width increased and UVVR decreased. Under low-intensity wave conditions, a 40-m leading edge with UVVR 0.2 (∼85% vegetated) can reduce wave energy by at least 80% (Figure 3A). Under medium-intensity wave conditions, a 30-m leading edge at UVVR 1 (50% vegetated) can provide the same percent reduction (Figure 3B). Under high-intensity wave conditions, a 30-m leading edge at UVVR 1 (50% vegetated) can provide more than 85% reduction (Figure 3C). Under higher-intensity wave conditions, even thinner, leading edges at higher UVVRs can provide more than 75% reduction in wave energy. A 10 m vegetated edge, perhaps more accessible to homeowners, will still provide >50% reduction in wave energy at low-intensity conditions and >80% reduction at high-intensity conditions, though additional reduction from increased vegetation is more apparent at vegetated leading edges 20 m and wider.

FIGURE 3

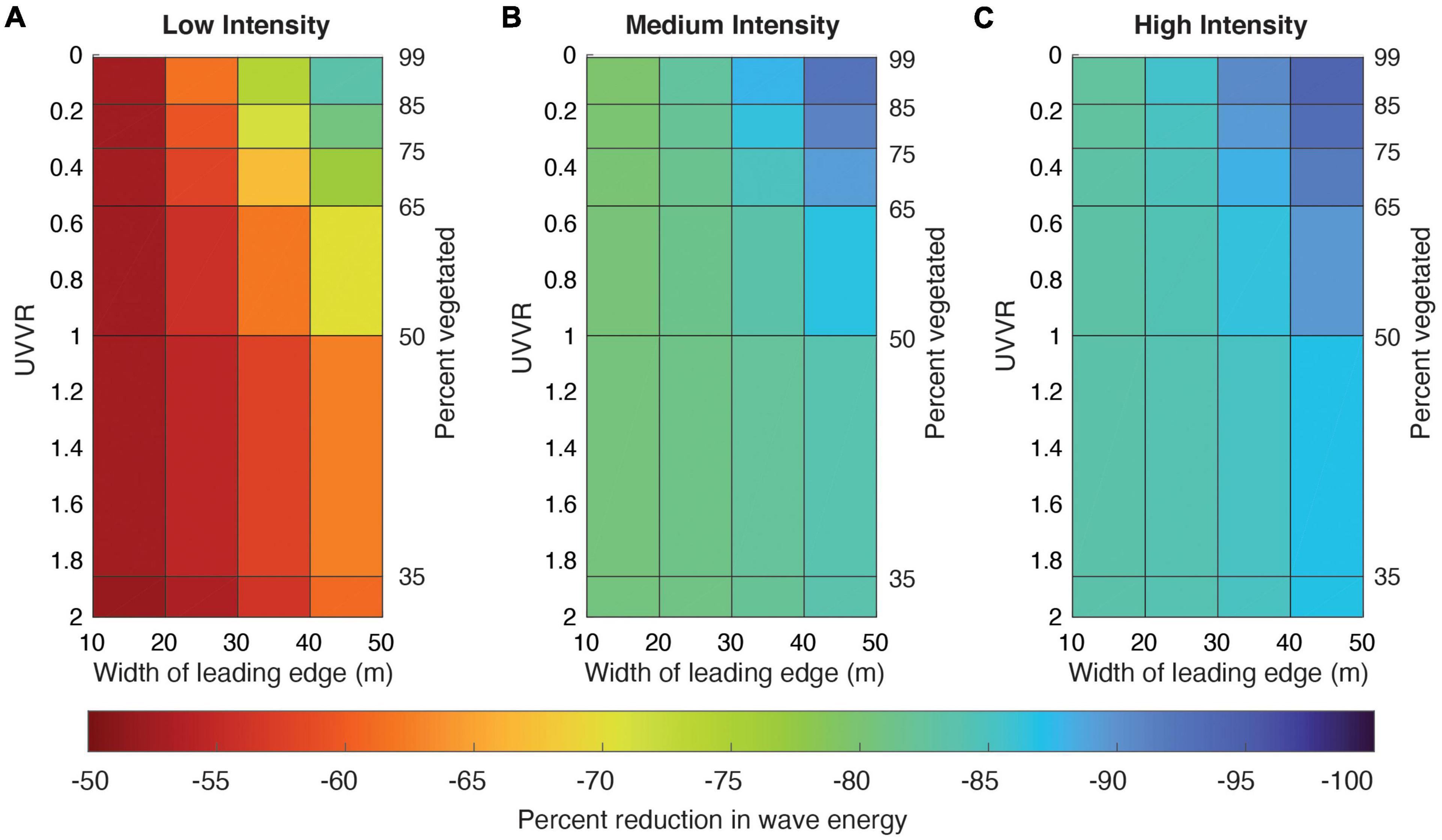
Figure 3. Relationship among the width of a vegetated leading edge, UVVR, and percent reduction in wave energy over the first 100 m of marsh for (A) low-, (B) medium-, and (C) high-intensity wave scenarios.

Lifespan Estimates Under Sea-Level Rise Scenarios

To assess the relationship between UVVR and marsh lifespan, lifespans under future global mean sea-level scenarios were calculated using qualities of the model domain and averaged downscaled SLR values for several United States marshes (Figure 4). Under background rates of SLR (∼1 mm/year per Sweet et al., 2017), our study marsh (with an initial average elevation of 1.2 m) restored to UVVR 1 has a lifespan of 208 years. For 0.3, 0.5, and 1.0 m SLR rise by 2100, lifespan decreased to 130, 120, and 75 years, respectively. A marsh restored to UVVR 0.1, identified as a key tipping point in marsh stability (Ganju et al., 2016), has a lifespan of 3,477, 540, 446, and 200 years, for background, 0.3, 0.5, and 1.0 m SLR rise scenarios, respectively.

FIGURE 4

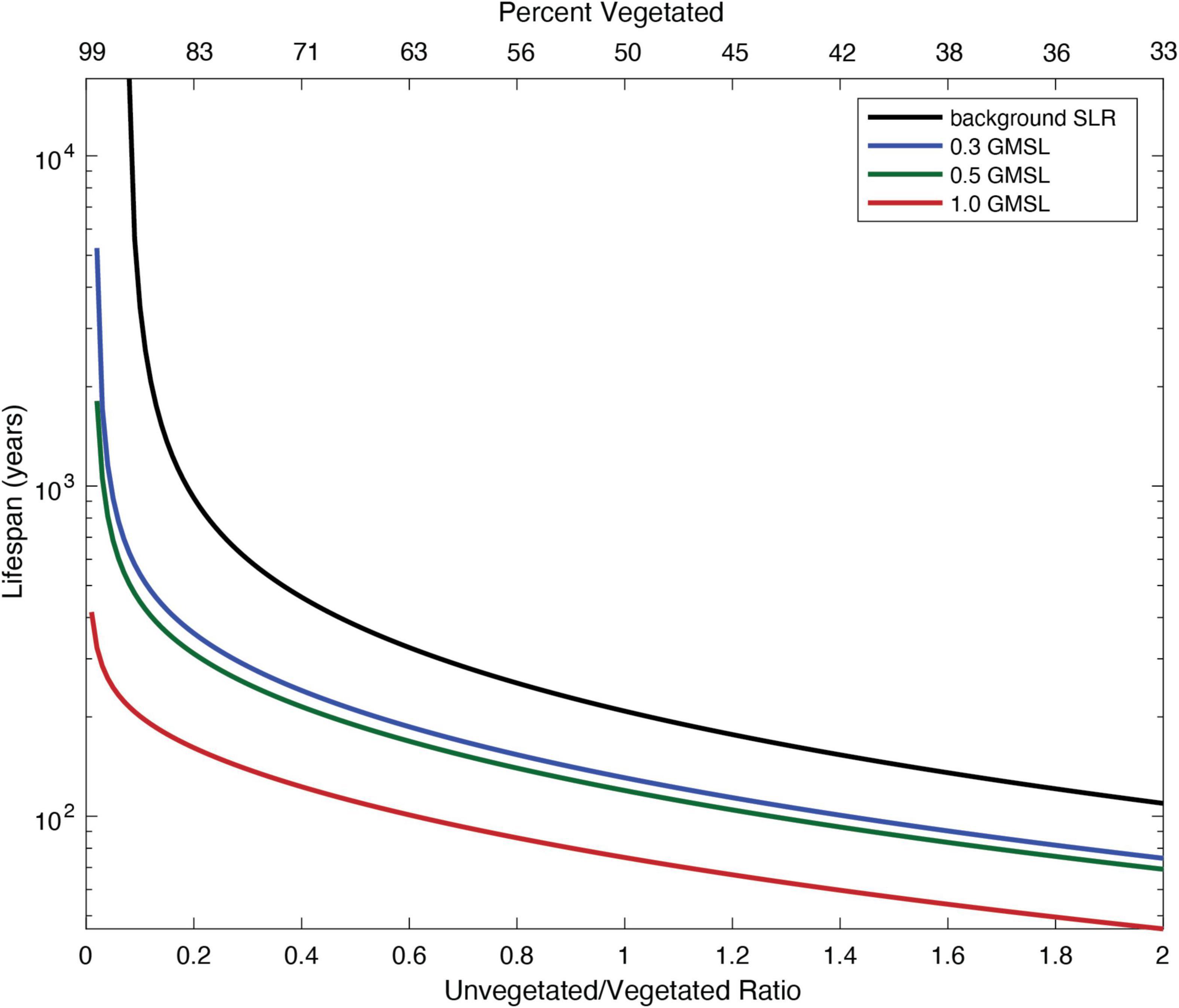
Figure 4. Estimated lifespan (per Ganju et al., 2020) for varying UVVR values of the idealized model marsh in the study under background and 0.3, 0.5, and 1.0 m sea-level rise (SLR) by 2100 scenarios.

Discussion

Studies have quantified wave attenuation by marsh vegetation in field (Möller and Spencer, 2002; Lightbody and Nepf, 2006; Jadhav et al., 2013) and lab (Möller et al., 2014; Rupprecht et al., 2017) settings. Highly sophisticated and often computationally expensive models have been used to examine wave attenuation across specific locations (Loder et al., 2009; Marsooli et al., 2017) or within relatively small spatial constraints (Wu et al., 2016). Framing wave energy reduction as a function of marsh restoration, however, has been less studied. This study was specifically designed with a management perspective in mind, using generalized and representative model input to broadly look at relationships between marsh vegetation distribution and wave attenuation in a functional restoration context. Practitioners may be more familiar with and better able to implement percent cover measurements as part of an intensive monitoring program. UVVR measurements, particularly the datasets available for entire United States coastline through the U.S. Geological Survey (Couvillion et al., 2021), may be more appropriate for identification of vulnerable areas suitable for restoration. As such, this study has been framed in terms of both UVVR and percent vegetative cover to maximize its utility in multiple applications.

The amount of vegetation cover in a marsh, in tandem with its elevation capital, influences its ability to reduce wave energy. Regardless of marsh morphology, a marsh with 50% vegetated (UVVR 1) is likely able to reduce storm wave energy by 95% within the first 100 m; which represents a strong benchmark value for hazard mitigation. Marshes with ponding morphology are better able to reduce wave energy compared to marshes with channel morphology, especially at lower wave intensity and reduced vegetation scenarios. This is likely due to a focusing effect of the channels, which can funnel larger waves further than if they were traveling over a smoother, more vegetated topography. Marshes with a leading mudflat performed better than channeled and ponded marshes and nearly as well as the fully vegetated scenarios. This is likely due to a combination of consistent vegetation, uninterrupted by major topographic features, and the general ability of the system to reduce wave heights and subsequent wave energy through increasing platform elevation and decreasing water depth. Though model runs with no vegetation indicate that wave energy is reduced substantially by the reduction in water depth and its associated wave breaking, a 95% reduction in wave energy when including vegetation is of critical importance. In 50% vegetated (UVVR 1) scenarios, for example, wave heights fall from almost 2 m to approximately 40 cm in the high-intensity wave scenario and from 0.9 m to less than 20 cm in the low-intensity wave scenario. While this study is limited by specific vegetation configurations and storm conditions by design, the 50% vegetated (UVVR 1) benchmark is consistent across a wide range of wave intensities and marsh morphologies.

Given this benchmark, a marsh restoration project designed with at least 50% vegetated cover (UVVR ≤ 1) could maximize the ecosystem service of wave attenuation. This success metric can be incorporated by practitioners when determining overall planting density and distribution. While planting distribution and density requirements may vary from project to project, maintaining at least 50% vegetation (UVVR ≤ 1) in a marsh at least 100 m in width could provide substantial coastal protection from storm waves. The 50% vegetation benchmark is supported in the literature; small-scale experimental plots of Juncus roemerianus planted at half density (roughly equivalent to UVVR∼1) in the Grand Bay National Estuarine Research Reserve in Mississippi, United States, were similarly or more cost effective in regards to both planting cost and effort than planting the plots at full density (roughly equivalent to UVVR ≤ 0.1), particularly because total vegetated coverage was similar between both the full and half density plots approximately 2 years after planting (Sparks et al., 2013). While our study randomly placed vegetation throughout the model domain, specific planting patterns (for instance, grouping plants in clusters or clumps instead of planting the plugs in a systematic grid) may also substantially increase future plant yield and survival (Silliman et al., 2015). These decisions will become even more important as the knowledge and practice of nature-based solutions continues to expand (Castagno et al., 2021).

Considering trade-offs is important for maximization of restoration success and cost-effectiveness. In situations where only a smaller leading edge can be planted, maintaining at least 75% vegetated cover (UVVR ≤ 0.33) in a 50-m-wide area will provide an 80–90% reduction in wave energy. In the case of homeowners, who may only be able to restore a smaller stretch of marsh in front of their properties, a 10 m vegetated edge under low-energy conditions will provide >50% reduction in wave energy and >80% reduction under high-intensity conditions. Practitioners, planners, and managers can use the relationships presented in Figure 4 to decide trade-offs, e.g., planting a 30-m leading edge at 50% vegetated cover (UVVR 1) may be sufficient if restoration emphasis is placed primarily on wave energy reduction of high-intensity storms.

It is important to consider, however, that a restored marsh’s lifespan is largely dependent on sediment supply (Ganju, 2019), so a sparsely vegetated, restored system that lacks sufficient sediment input will have a limited lifespan. Marsh lifespan calculations for the model domain indicate that 50% vegetated cover (UVVR∼1) corresponds to a lifespan of ∼200 years under background scenarios. With 1.0 m SLR by 2100, the maximum scenario considered in this study, marsh lifespan decreases to 75 years. Alternatively, 90% vegetated cover (UVVR ∼ 0.1) corresponds to a model marsh lifespan of ∼3,500 years under background SLR conditions and ∼200 years for 1.0 m SLR. It is important to consider that this is a conservative case; average elevation for the model marsh at 1.2 m is already relatively high, and marshes with lower elevation capital will have even shorter lifespans.

Desired lifespan of the restoration project should also be considered when determining vegetation density. For example, assuming moderate SLR projections (0.5 m SLR by 2100) and a baseline price of $1.25 per 5-cm plug of Spartina alterniflora (2021 bulk-purchase value from New England Wetland Plants, a common distributor for New England restoration projects) with 10 plugs per square meter, planting the model domain at 50% cover (UVVR 1) would cost ∼$614 thousand for a marsh with a lifespan of 120 years, whereas planting the model domain to 90% (UVVR 0.1) would cost less than twice as much (∼$1.1) million for a lifespan almost four times that of the reduced density scenario. Planting the model domain to 90% instead of 50% would also provide increased wave attenuation benefits, with percent reduction in wave energy increasing to ∼99% under medium- and high-intensity wave scenarios.

The relationships between UVVR and wave attenuation can also be applied to existing marshes to determine their utility for coastal protection in attenuation of storm waves. UVVR and percent cover are directly related, but UVVR is determined through aerial imagery and less labor intensive than boots-on-the-ground field studies (Ganju et al., 2016). As previously mentioned, UVVR values are available for entire contiguous United States coastline through the U.S. Geological Survey (Couvillion et al., 2021) and can be generated through analysis of existing infrared aerial photography (Ganju et al., 2016). A marsh area of ≥100 m with a UVVR value of ≤1 (≥50%) has the capacity to reduce wave energy by ∼95%. Spatial analysis of UVVR across multiple marshes or within individual marshes can identify areas that are particularly capable of reducing storm wave energy (highly vegetated) or that may benefit from restoration (UVVR ≥ 1). While more resource-intensive, surveys incorporating aerial imagery for UVVR analysis have great utility in assessing the resilience of larger areas of marsh as part of an initial baseline assessment of a site or a long-term monitoring program.

Focusing marsh protection and restoration efforts specifically on flood risk reduction has the potential to significantly bolster the resilience of vulnerable coastal communities. Analysis of coastal protection ecosystem services for hazard-mitigation valuation and insurance practices has already begun for coral reef (Reguero et al., 2020) and mangrove systems (Menéndez et al., 2020). Incorporating this understanding of how much restoration is necessary to provide significant wave energy benefits into Federal Emergency Management Agency Benefit-Cost Analyses for hazard mitigation or future flood risk models strengthens the argument for nature-based solutions to coastal protection and shoreline stabilization. This is of particular importance in areas where marshes are the primary form of natural coastal protection (e.g., the northeastern United States, where the climate is prohibitive for mangrove or coral reef protection, among other locations). Lifespan estimates are an important consideration when selecting vegetation density, since marshes with greater elevation capital and/or more vegetation have longer lifespans and tend to be more resilient to sea-level rise. Since the relationship between UVVR and wave attenuation is roughly linear within UVVR’s functional limits for wetlands, the straightforward equations presented in this study can be applied easily in a wide variety of contexts. Future research, including the application of more sophisticated or detailed models, analysis of model sensitivities to a spectrum of vegetation and wave characteristics, and field-based ground truthing could enhance the ability to refine these relationships and our understanding of how marsh restoration can be leveraged to maximize coastal protection benefits.

Conclusion

This study found a substantial reduction in storm wave energy when the first 100 m of a marsh is at least 50% vegetated, though lifespan estimates, including both marsh elevation and desired UVVR, for restored marshes are important factors when considering overall restoration investment and return decisions. As coastal communities continue to grow more vulnerable to sea-level rise and increased storminess, it becomes even more important to maximize coastal protection benefits through nature-based solutions, including marsh restoration. The benchmarks and empirical relationships presented in this study have significant implications for coastal practitioners and managers seeking timely performance goals and metrics for marsh restoration, enhancement, and creation.

Author’s Note

Any use of trade, firm, or product names is for descriptive purposes only and does not imply endorsement by the U.S. Government.

Data Availability Statement

The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.

Author Contributions

KC and NG conceived the manuscript. KC performed the model runs, analyzed the results, and wrote the first draft of the manuscript. NG, MB, AB, and SS offered critical insight and edits to the manuscript. All authors contributed to the article and approved the submitted version.

Conflict of Interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Publisher’s Note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

Supplementary Material

The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fmars.2021.756670/full#supplementary-material

References

Anderson, M. E., and Smith, J. M. (2014). Wave attenuation by flexible, idealized salt marsh vegetation. Coast. Eng. 83, 82–92. doi: 10.1016/j.coastaleng.2013.10.004

CrossRef Full Text | Google Scholar

Bendoni, M., Georgiou, I. Y., Roelvink, D., and Oumeraci, H. (2019). Numerical modelling of the erosion of marsh boundaries due to wave impact. Coast. Eng. 152:103514. doi: 10.1016/j.coastaleng.2019.103514

CrossRef Full Text | Google Scholar

Booij, N., Ris, R. C., and Holthuijsen, L. H. (1999). A third-generation wave model for coastal regions: 1. Model description and validation. J. Geophys. Res. 104, 7649–7666. doi: 10.1029/98JC02622

CrossRef Full Text | Google Scholar

Burdick, D. M., and Roman, C. T. (2012). “Salt marsh responses to tidal restriction and restoration,” in Tidal Marsh Restoration. The Science and Practice of Ecological Restoration, eds C. T. Roman and D. M. Burdick (Washington, DC: Island Press). doi: 10.5822/978-1-61091-229-7_22

CrossRef Full Text | Google Scholar

Castagno, K. A., Burns, S. E., Bowden, A. A., Roberts, E. J., Harlan, S. L., Senier, L., et al. (2021). Conservation practice insights from a comparative case study of two shoreline stabilization projects in Boston. Conserv. Sci. Pract. 3:e465. doi: 10.1111/csp2.465

CrossRef Full Text | Google Scholar

Couvillion, B. R., Ganju, N. K., and Defne, Z. (2021). An Unvegetated to Vegetated Ratio (UVVR) for Coastal Wetlands of the Conterminous United States (2014-2018). Reston, VA: U.S. Geological Survey data release. doi: 10.5066/P97DQXZP

PubMed Abstract | CrossRef Full Text | Google Scholar

D’Alpaos, A. (2011). The mutual influence of biotic and abiotic components on the long-term ecomorphodynamic evolution of salt-marsh ecosystems. Geomorphology 126, 269–278. doi: 10.1016/j.geomorph.2010.04.027

CrossRef Full Text | Google Scholar

Dalrymple, R. A., Kirby, J. T., and Hwang, P. A. (1984). Wave diffraction due to areas of energy dissipation. J. Waterw. Port Coast. Ocean Eng. 110, 67–79. doi: 10.1061/(ASCE)0733-950X(1984)110:1(67)

CrossRef Full Text | Google Scholar

Day, J. W., Boesch, D. F., Clairain, E. J., Kemp, G. P., Laska, S. B., Mitsch, W. J., et al. (2007). Restoration of the Mississippi Delta: lessons from hurricanes Katrina and Rita. Science 315, 1679–1684. doi: 10.1126/science.1137030

PubMed Abstract | CrossRef Full Text | Google Scholar

Ganju, N. K. (2019). Marshes are the new beaches: integrating sediment transport into restoration planning. Estuaries Coasts 42, 917–926. doi: 10.1007/s12237-019-00531-3

CrossRef Full Text | Google Scholar

Ganju, N. K., Defne, Z., and Fagherazzi, S. (2020). Are elevation and open-water conversion of salt marshes connected? Geophys. Res. Lett. 47:e2019GL086703. doi: 10.1029/2019GL086703

CrossRef Full Text | Google Scholar

Ganju, N. K., Defne, Z., Kirwan, M. L., Fagherazzi, S., and Carniello, L. (2016). Spatially integrative metrics reveal hidden vulnerability of microtidal salt marshes. Nat. Commun. 8:14156. doi: 10.1038/ncomms14156

PubMed Abstract | CrossRef Full Text | Google Scholar

Gedan, K. B., Kirwan, M. L., Wolanski, E., Barbier, E. B., and Silliman, B. R. (2011). The present and future role of coastal wetland vegetation in protecting shorelines: answering recent challenges to the paradigm. Clim. Chang. 106, 7–29. doi: 10.1007/s10584-010-0003-7

CrossRef Full Text | Google Scholar

Hasselmann, K., Barnett, T., Bouws, E., Carlson, H., Cartwright, D., Enke, K., et al. (1973). Measurements of wind-wave growth and swell decay during the Joint North Sea wave project (JONSWAP). Ergänzungsheft Dtsch. Hydrogr. Z. A 8:95.

Google Scholar

Iwasaki, T., Shimizu, Y., and Kimura, I. (2013). Modelling of the initiation and development of tidal creek networks. Proc. Inst. Civil Eng. Marit. Eng. 166, 76–88. doi: 10.1680/maen.2012.12

PubMed Abstract | CrossRef Full Text | Google Scholar

Jadhav, R. S., Chen, Q., and Smith, J. M. (2013). Spectral distribution of wave energy dissipation by salt marsh vegetation. Coast. Eng. 77, 99–107. doi: 10.1016/j.coastaleng.2013.02.013

CrossRef Full Text | Google Scholar

Lightbody, A. F., and Nepf, H. M. (2006). Prediction of velocity profiles and longitudinal dispersion in emergent salt marsh vegetation. Limnol. Oceanogr. 51, 218–228. doi: 10.4319/lo.2006.51.1.0218

CrossRef Full Text | Google Scholar

Loder, N. M., Irish, J. L., Cialone, M. A., and Wamsley, T. V. (2009). Sensitivity of hurricane surge to morphological parameters of coastal wetlands. Estuar. Coast. Shelf Sci. 84, 625–636. doi: 10.1016/j.ecss.2009.07.036

CrossRef Full Text | Google Scholar

Marsooli, R., Orton, P. M., and Mellor, G. (2017). Modeling wave attenuation by salt marshes in Jamaica Bay, New York, using a new rapid wave model. J. Geophys. Res. Ocean. 122, 5689–5707. doi: 10.1002/2016JC012546

CrossRef Full Text | Google Scholar

Menéndez, P., Losada, I. J., Torres-Ortega, S., Narayan, S., and Beck, M. W. (2020). The global flood protection benefits of mangroves. Sci. Rep. 10:4404. doi: 10.1038/s41598-020-61136-6

PubMed Abstract | CrossRef Full Text | Google Scholar

Möller, I., Kudella, M., Rupprecht, F., Spencer, T., Paul, M., van Wesenbeeck, B. K., et al. (2014). Wave attenuation over coastal salt marshes under storm surge conditions. Nat. Geosci. 7, 727–731. doi: 10.1038/ngeo2251

CrossRef Full Text | Google Scholar

Möller, I., and Spencer, T. (2002). Wave dissipation over macro-tidal saltmarshes: effects of marsh edge typology and vegetation change. J. Coast. Res. 36, 506–521.

Google Scholar

Morris, J. T., Barber, D. C., Callaway, J. C., Chambers, R., Hagen, S. C., Hopkinson, C. S., et al. (2016). Contributions of organic and inorganic matter to sediment volume and accretion in tidal wetlands at steady state. Earths Future 4, 110–121. doi: 10.1002/2015EF000334

PubMed Abstract | CrossRef Full Text | Google Scholar

Narayan, S., Beck, M. W., Reguero, B. G., Losada, I. J., van Wesenbeeck, B., Pontee, N., et al. (2016). The effectiveness, costs and coastal protection benefits of natural and nature-based defences. PLoS One 11:e0154735. doi: 10.1371/journal.pone.0154735

PubMed Abstract | CrossRef Full Text | Google Scholar

Narayan, S., Beck, M. W., Wilson, P., Thomas, C. J., Guerrero, A., Shepard, C. C., et al. (2017). The value of coastal wetlands for flood damage reduction in the northeastern USA. Sci. Rep. 7:9463. doi: 10.1038/s41598-017-09269-z

PubMed Abstract | CrossRef Full Text | Google Scholar

Reguero, B. G., Beck, M. W., Schmid, D., Stadtmüller, D., Raepple, J., Schüssele, S., et al. (2020). Financing coastal resilience by combining nature-based risk reduction with insurance. Ecol. Econ. 169:106487. doi: 10.1016/j.ecolecon.2019.106487

CrossRef Full Text | Google Scholar

Rupprecht, F., Möller, I., Paul, M., Kudella, M., Spencer, T., van Wesenbeeck, B. K., et al. (2017). Vegetation-wave interactions in salt marshes under storm surge conditions. Ecol. Eng. 100, 301–315. doi: 10.1016/j.ecoleng.2016.12.030

CrossRef Full Text | Google Scholar

Shepard, C. C., Crain, C. M., and Beck, M. W. (2011). The protective role of coastal marshes: a systematic review and meta-analysis. PLoS One 6:e27374. doi: 10.1371/journal.pone.0027374

PubMed Abstract | CrossRef Full Text | Google Scholar

Silliman, B. R., Schrack, E., He, Q., Cope, R., Santoni, A., Van Der Heide, T., et al. (2015). Facilitation shifts paradigms and can amplify coastal restoration efforts. Proc. Natl. Acad. Sci. U.S.A. 112, 14295–14300. doi: 10.1073/pnas.1515297112

PubMed Abstract | CrossRef Full Text | Google Scholar

Smith, C. S., Puckett, B., Gittman, R. K., and Peterson, C. H. (2018). Living shorelines enhanced the resilience of saltmarshes to Hurricane Matthew (2016). Ecol. Appl. 28, 871–877. doi: 10.1002/eap.1722

PubMed Abstract | CrossRef Full Text | Google Scholar

Sparks, E. L., Cebrian, J., Biber, P. D., Sheehan, K. L., and Tobias, C. R. (2013). Cost-effectiveness of two small-scale salt marsh restoration designs. Ecol. Eng. 53, 250–256. doi: 10.1016/j.ecoleng.2012.12.053

CrossRef Full Text | Google Scholar

Spivak, A. C., Gosselin, K., Howard, E., Mariotti, G., Forbrich, I., Stanley, R., et al. (2017). Shallow ponds are heterogeneous habitats within a temperate salt marsh ecosystem. J. Geophys. Res. Biogeosci. 122, 1371–1384. doi: 10.1002/2017JG003780

CrossRef Full Text | Google Scholar

Sweet, W. V., Kopp, R. E., Weaver, C. P., Obeysekera, J., Horton, R. M., Thieler, E. R., et al. (2017). Global and Regional Sea Level Rise Scenarios for the United States (Tech. Rep. NOS CO-OPS 083). Silver Spring, MD: National Oceanic and Atmospheric Administration.

Google Scholar

Temmerman, S., Meire, P., Bouma, T. J., Herman, P. M. J., Ysebaert, T., and de Vriend, H. J. (2013). Ecosystem-based coastal defence in the face of global change. Nature 504, 79–83. doi: 10.1038/nature12859

PubMed Abstract | CrossRef Full Text | Google Scholar

U.S. Army Corps of Engineers. (2022). Thin-Layer Placement of Dredged Material. Available online at: https://tlp.el.erdc.dren.mil/

Google Scholar

Vuik, V., Jonkman, S. N., Borsje, B. W., and Suzuki, T. (2016). Nature-based flood protection: the efficiency of vegetated foreshores for reducing wave loads on coastal dikes. Coast. Eng. 116, 42–56. doi: 10.1016/j.coastaleng.2016.06.001

CrossRef Full Text | Google Scholar

Wamsley, T. V., Cialone, M. A., Smith, J. M., Ebersole, B. A., and Grzegorzewski, A. S. (2009). Influence of landscape restoration and degradation on storm surge and waves in southern Louisiana. Nat. Hazards 51, 207–224. doi: 10.1007/s11069-009-9378-z

CrossRef Full Text | Google Scholar

Wasson, K., Ganju, N. K., Defne, Z., Endris, C., Elsey-Quirk, T., Thorne, K. M., et al. (2019). Understanding tidal marsh trajectories: evaluation of multiple indicators of marsh persistence. Environ. Res. Lett. 14:124073. doi: 10.1088/1748-9326/ab5a94

CrossRef Full Text | Google Scholar

Wu, W. C., Ma, G., and Cox, D. T. (2016). Modeling wave attenuation induced by the vertical density variations of vegetation. Coast. Eng. 112, 17–27. doi: 10.1016/j.coastaleng.2016.02.004

CrossRef Full Text | Google Scholar