New Methods for Processing and Quantifying VO2 Kinetics to Steady State: VO2 Onset Kinetics

Current methods of oxygen uptake (VO₂) kinetics data handling may be too simplistic for the complex physiology involved in the underlying physiological processes. Therefore, the aim of this study was to quantify the VO₂ kinetics to steady state across the full range of sub-ventilatory threshold work rates, with a particular focus on the VO₂ onset kinetics. Ten healthy, moderately trained males participated in five bouts of cycling. Each bout involved 10 min at a percentage of the subject's ventilation threshold (30, 45, 60, 75, 90%) from unloaded cycling. The VO₂ kinetics was quantified using the conventional mono-exponential time constant (tau, τ), as well as the new methods for VO₂ onset kinetics. Compared to linear modeling, non-linear modeling caused a deterioration of goodness of fit (main effect, p < 0.001) across all exercise intensities. Remainder kinetics were also improved using a modified application of the mono-exponential model (main effect, p < 0.001). Interestingly, the slope from the linear regression of the onset kinetics data is similar across all subjects and absolute exercise intensities, and thereby independent of subject fitness and τ. This could indicate that there are no functional limitations between subjects during this onset phase, with limitations occurring for the latter transition to steady state. Finally, the continuing use of mono-exponential modeling could mask important underlying physiology of more instantaneous VO₂ responses to steady state. Consequently, further research should be conducted on this new approach to VO₂ onset kinetics.

Introduction

Earlier work in oxygen uptake (VO₂) kinetics suggested a three-phase approach, from the onset of—or increase in—work to the attainment of steady state VO₂ in sub-threshold intensities (Whipp et al., 1982) (Figure 1). Phase-I is said to represent the oxygen (O₂) exchange associated with the initial elevation of cardiac output, and therefore pulmonary blood flow (Jones and Poole, 2005). Phase-II is traditionally identified as an increase toward a steady state VO₂ following the cessation of phase-I, to be reflective of the increased VO₂ of contracting muscle and is primarily modeled using a mono-exponential equation (Whipp and Ward, 1990). Finally, phase-III indicates the attainment of steady state VO₂ (Whipp et al., 1982).

FIGURE 1

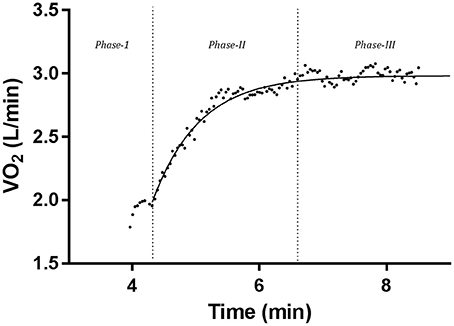
Figure 1. Individual $\dot{\text{V}}$O₂ kinetics data displaying the three-phase approach to steady state $\dot{\text{V}}$O₂ following the onset of, or increase in, work load. This bout involved baseline unloaded cycling, followed by a workload increase of 75% VT. Data was averaged at a 7-breath average.

As stated, phase-II is modeled using a mono-exponential equation (Equation 1) incorporating a time constant (tau; τ) that was originally adapted to VO₂ kinetics data processing by Whipp (1971). This method has been used almost exclusively to process VO₂ kinetics data over the past 45 years (Linnarsson, 1974; Hughson and Morrissey, 1982; Barstow et al., 1993; Brittain et al., 2001; Spencer et al., 2013). The equation, which is sometimes slightly modified to suit study methodologies (for example, the inclusion or exclusion of a time delay), is generally presented as:

$\begin{array}{cc}\Delta \text{Y}(t)=\Delta \text{Y}(\text{ss})\{1-e^{-1(t-\text{TD})/\tau }\}& (1)\end{array}$

where Y represents VO₂; ΔY(t) is the increase in Y above the prior steady state value at time t; ΔY(ss) is the steady state increase in Y; TD is the phase-I time delay; and τ is the time constant of the response.

This method of data processing, although extensively used in research, has at times displayed inconsistencies in past research. In particular, phase-II kinetics are ascribed to a first order mechanism (i.e., follow a time constant that is invariant across increasing exercise intensities). Some earlier research has discussed irregularities with this interpretation (Linnarsson, 1974; Casaburi et al., 1989), while Hughson and Morrissey (1982) and Koppo et al. (2004) identified a clear slowing (increasing τ) of phase-II kinetics to steady state. They claimed that a three-phase model of VO₂ kinetics was only useful when all three phases are very clearly defined. This is often not the case, and is highly dependent on subject's health status, fitness, and comfort with the study's mode of exercise (Weltman and Katch, 1976; Spiro, 1977; Hickson et al., 1978; Nery et al., 1982). Stirling et al. (2005) also stated that it was unclear whether the three distinct phases would provide a reasonable model of the underlying physiological processes.

Through work in the field, the authors have identified a trend within the VO₂ data which they believe needs to be further assessed. From the termination of phase-I (where it exists in the data), until the beginning of a non-linear response in phase-II, there is a clear linear pattern within the data. The authors have termed this initial linearity as “onset kinetics”. As can be seen in Figure 2, the onset kinetics follow a previously non-modeled linear approach for the first ~1 min following the initiation of phase-II.

FIGURE 2

Figure 2. Single bout $\dot{\text{V}}$O₂ data with a 90% VT increase from unloaded cycling, fitted with a linear regression to the onset segment, and overlayed with a mono-exponential model (dashed line) without a time delay from the parameters of the entire data set.

Given that past researchers have acknowledged that the VO₂ kinetic response to increases in steady state workload is quite complex (Hughson and Morrissey, 1982; Koppo et al., 2004; Stirling et al., 2005; Robergs, 2014; McNulty et al., 2015), and that the initial onset response (see Figure 2) appears to be linear, there is merit to investigate the linear onset kinetics of VO₂ during exercise transitions to steady state. Consequently, the aims of this paper were to (a) compare a linear regression fit of the onset kinetics to a mono-exponential fit of the data, (b) compare the mono-exponential model fit for phase-II to a linear regression fit for the onset kinetics + mono-exponential fit to the remainder of phase-II.

Materials and Methods

Participants

Ten male subjects (mean age = 28 ± 9 years; height = 178.4 ± 7.7 cm; weight = 76.9 ± 11.3 kg) were recruited and completed the exercise trials of this study. The criteria for recruitment were healthy males aged between 18 and 45 years, and free from musculo-skeletal injury, or the presence of cardio-pulmonary and metabolic disease or more than two risk factors for disease. In addition, each participant was recruited on a basis of self-reported physical fitness (the minimum requirements for recruitment purposes were current endurance training for at least 45 min, three times per week), with a measured VO_2max ≥ 40 mL/kg/min. Recruitment occurred at a country NSW university, as well as local gymnasiums. All participants were asked to complete an Exercise and Sports Science Australia: Adult Pre-Screening System tool (Exercise and Sports Science Australia, 2014) to determine that they were in good physical health with no musculoskeletal disorders or risk factors for sedentary lifestyle diseases. Written informed consent was obtained from each participant prior to data collection and all methods were approved by the institution's Human Research Ethics Committee.

Familiarization and Baseline Testing

After completion of informed consent, a familiarization session, as well as a VO₂ maximum ramp protocol cycle ergometer test, were administered for each participant. During the familiarization session, the subject's height and mass were recorded, and the cycle ergometer's seating and handle bar arrangement were adjusted for each subject's preference and biomechanical needs. These adjustments were recorded and maintained for all future bouts. Before exercising, the subjects were asked to remain seated for 5 min in order to ascertain a resting HR measure. The subjects were then asked to cycle at 100 W for several minutes until they had established a comfortable, and constant pedaling cadence. This cadence was the set point for the entirety of the testing for that individual subject.

Prior to conducting the VO₂ ramp test (and for all subsequent trials) the subject was fitted with a multiple one-way valve mouthpiece system supported by an acrylic head unit. Electrocardiography (ECG) was used to acquire heart rate throughout the VO₂ max test and trials using a 5-lead ECG configuration (CASE, GE Healthcare, Waukesha, USA). The ECG leads were attached using gel electrodes placed over the spine of both scapulae, the iliac crest of both ilia, and between the 4th and 5th intercostal space along the mid-axillary line of the left side of the torso. For indirect calorimetry, expired gas analysis was acquired using a 3 L latex compliant and elastic mixing bag placed on the expired port of the mouthpiece, and mixed expired air was sampled continuously and pumped to rapid response O₂ and carbon dioxide (CO₂) gas analysers (AEI Technologies, Pittsburgh, PA, USA). During and following each breath, the elastic recoil of the mixing bag caused air to be vented through a 1 cm diameter hole in the inferior end of the mixing bag. Expired gas signals were acquired for 100 ms at the start of each inspired breath and aligned to the timing of end expiration based on a pre-determined measured time-delay. Ventilation was measured by a flow turbine (UVM, VacuMed, Ventura, CA, USA) connected to the inspired side of the mouthpiece. All data were acquired using custom developed software (LabVIEW™, National Instruments, Austin, TX) and commercial electronic acquisition devices (National Instruments, Austin, TX). The breath-by-breath system was calibrated before the ramp test and before each bout in both trials using a 3 L syringe and commercial medical grade calibration gas (16.00% O₂ and 5.00% CO₂). These methods have been validated and described in more detail elsewhere (Kim and Robergs, 2012).

Administration of the VO₂ ramp test had the subject cycle at their predetermined cycling cadence, for which they were asked to maintain for the entire test. The ramp function for each subject was based on their self-reported endurance fitness, and the need to constrain the test to between 8 and 12 min (Buchfuhrer et al., 1983; Astorino et al., 2005; Yoon et al., 2007), and consequently varied between 25 and 35 W/min between subjects. The VO₂ ramp protocol consisted of 2 min of rested breathing (to attain a baseline reading), followed by 2 min at double the ramp function Watts, and then followed by a near continuous ramp function (increment at 0.5 Hz). The subjects were also instructed to continue cycling until volitional exhaustion which was defined by a decrease in cadence <40 rev/min (Astorino et al., 2000).

Using the breath-by-breath VO₂ data collected from the ramp test, the VT of each subject was determined visually by the ventilatory equivalent method (Gaskill et al., 2001) using a custom designed computer program (LabVIEW™, National Instruments, Austin, TX, USA). The VT was detected by the program through the user directed application of three linear segments to the data. The VT was computed as the time of the intersection between segment 1 (baseline response, slope ~ 0) and segment 2 (initial deviation from baseline). The detection of the VT required agreement between two investigators (agreement was set at ± 10 s). Where there was opposing detection, a third researcher was asked to interpret the data. The VT was then used to determine to cycle ergometer power output required for the two exercise trials.

Exercise Protocol

Research on phase-II VO₂ kinetics has only used a minimal number of magnitude increases (Whipp, 1971; Whipp et al., 1982; Whipp and Ward, 1990; Barstow et al., 1996). Therefore, a multiple-bout cycling trial was developed. The trial involved five separate bouts of unloaded cycling to different increments.

Following a 5 min warm-up at 50 W on the cycle ergometer, each participant was again fitted for indirect calorimetry and ECG. The cycling trial involved five bouts to differing magnitudes from unloaded cycling, with 15 min of seated rest between each bout to limit trial-to-trial variability due to post-exercise O₂ consumption (Borsheim and Bahr, 2003). The magnitudes in order of intensity were: 30, 45, 60, 75, and 90% of VT. The trial required the subject cycle at an unloaded magnitude for 2 min before completing 10 min at the increased magnitude. The participants were instructed to maintain the same comfortable cycling cadence for each bout despite the electronic ergometer adjusting resistance with changed cadence to ensure a stable power output. The order of administration of each magnitude were determined by a Latin Squares design (Kirk, 2010). A minimum time-frame of 48 h separated the completion of the VO₂ ramp test and the multiple-bout exercise trial.

Data Reduction and Analysis

The raw breath-by-breath data, which included absolute and relative VO₂, respiratory exchange ratio, and the ventilatory equivalent ratios for O and CO₂, were processed using a 7-breath average from custom designed software (LabVIEW™, National Instruments, Austin, TX). Each trial text file was imported into a commercial graphics and curve fitting program (Prism, GraphPad Software, La Jolla, CA, USA), and data were removed for the initial rest data collection of each trial. Data were then graphed and the phase-I VO₂ data were identified and then also removed for each trial. Initially, the whole data sets (phase-II and -III) were fitted using the mono-exponential function of Equation (1).

The onset VO₂ kinetics for each data set were fitted with a linear regression. The onset kinetics were visually identified separately by both authors. The onset data were defined as the data points which follow a near-linear fashion from the onset of phase-II (or the onset of exercise if there was no clear phase-I), until an evident decline in linearity within phase-II. The remainder data, in a separate Prism file, were then fitted with Equation (1).

The onset data were then modeled with Equation (1), using the parameters set by the application of the model to the entire data set (phase-II and -III). From here, standard error of the estimate (S_y.x) and coefficient of determination (R²), as well as their respective confidence intervals, between both linear and mono-exponential modeling were recorded.

The remainder segment of data were fit with a new application of Equation (1) and the model of the entire data set, again using the set parameters. S_y.x and R², as well as their respective confidence intervals, between both non-linear data fits were recorded.

Statistical Analyses

Statistical analysis of the data was performed using SPSS 23 (IBM Corporation, New York, NY, USA). The subjects of this study completed five cycling bouts of varying intensity (30, 45, 60, 75, and 90% VT). The data was processed in order to ascertain S_y.x and R² for VO₂.

To assess the goodness of fit between a linear regression vs. mono-exponential (non-linear) modeling approach to the onset VO₂ data, two separate analysis of variance (ANOVA) were used. A two-way ANOVA (INTENSITY [5] × METHOD [2]) was implemented to analyse the S_y.x for both linear, and non-linear models. Following this, a second two-way ANOVA (INTENSITY [5] × METHODS [2]) was used to analyse the R² values of the data for both linear and non-linear models.

Following the identification and analysis of the onset VO₂ kinetics data, there remains the latter-end of the phase-II breath-by-breath data to phase-III, which continues up until the termination of each exercise bout. This data has been labeled “remainder” by the authors. Another set of ANOVAs were used to analyse both the S_y.x and R² of the remainder data to assess goodness of fit of two non-linear models. A two-way ANOVA (INTENSITY [4] × METHOD [2]) was implemented to analyse the S_y.x for both a non-linear model applied to the remainder data, and the full mono-exponential model (from the entire data set) fitted to the remainder data. Following this, a second two-way ANOVA (INTENSITY [4] × METHODS [2]) was used to analyse the R²-values of the data for both of these fits. Note the 30% intensity data was omitted from the analysis of the remainder as the relatively low increase in power output (and therefore ΔVO₂) would not allow for mono-exponential modeling of most subject data.

Finally, each individual power output (30, 45, 60, 75, and 90% VT) of all bouts for each subject—presented as an absolute Watts value—was graphed against the respective slope (L/min⁻²) of the VO₂ onset kinetics.

Significance was set at p < 0.05. All data are presented as mean ± SD.

Results

For the mean onset linear and non-linear VO₂ data in Figure 3A, S_y.x was significantly lower (main effect, p < 0.001) with the linear model fit compared to the non-linear fit. There was a significant (p = 0.02) main effect for exercise intensity (30, 45, 60, 75, and 90% VT) and a significant interaction (p = 0.04) between exercise intensity and method, where the increase in S_y.x for the non-linear model fit was significant for exercise intensities above 60% VT.

FIGURE 3

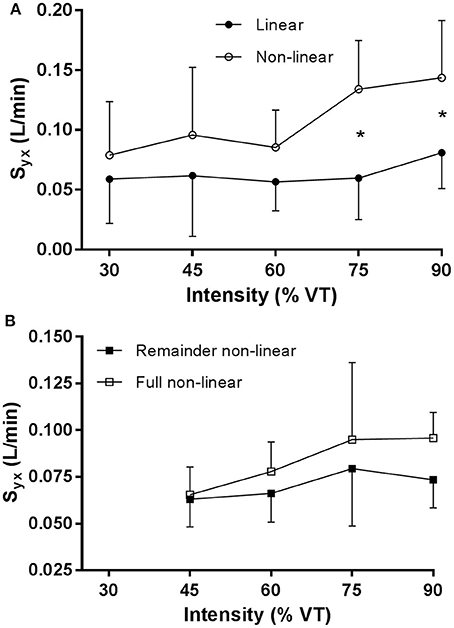
Figure 3. (A) S_y.x $\dot{\text{V}}$O₂ data for the linear regression model and non-linear model for the onset kinetics data, and (B) S_y.x $\dot{\text{V}}$O₂ data for the remainder data and the full mono-exponential fitted to the remainder data. *p < 0.05.

For the mean remainder, non-linear and full mono-exponential VO₂ data in Figure 3B, S_y.x was significantly lower (main effect, p < 0.001) with the non-linear model fit compared to the full mono-exponential fit. There was no significant main effect for exercise intensity. There was no significant interaction between exercise intensity and method.

For the mean onset linear and non-linear VO₂ data in Figure 4A, R² was significantly (main effect, p < 0.001) higher with the linear fit compared to the non-linear fit. There was a significant main effect (p = 0.02) for exercise intensity (30, 45, 60, 75, 90% VT). There was no significant interaction between exercise intensity and method.

FIGURE 4

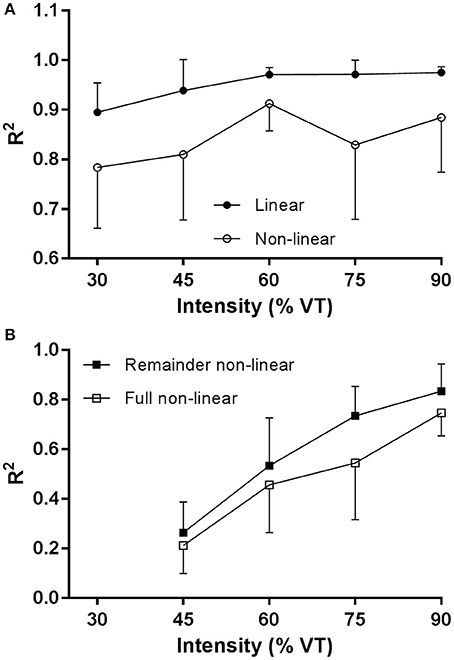
Figure 4. (A) R² $\dot{\text{V}}$O₂ data for the linear regression model and non-linear model for the onset kinetics data, and (B) R² $\dot{\text{V}}$O₂ data for the remainder data and the full mono-exponential fit to the remainder data.

For the remainder non-linear and full mono-exponential VO₂ data in Figure 4B, R² was significantly higher (main effect, p < 0.001) with the non-linear fit compared to the full mono-exponential fit. There was a significant (main effect, p < 0.001) effect for exercise intensity (45, 60, 75, 90% VT). There was no significant interaction between exercise intensity and method.

Finally, Figure 5 presents the power (Watts) vs. slope (L/min⁻²) data for all subjects and all exercise bouts (30, 45, 60, 75, and 90% VT) for the onset linear regression fit. The regression data are incredibly invariant, revealing that despite subjects of differing fitness, the variability in linear onset kinetics was 62% explained by absolute power output (Watts) alone.

FIGURE 5

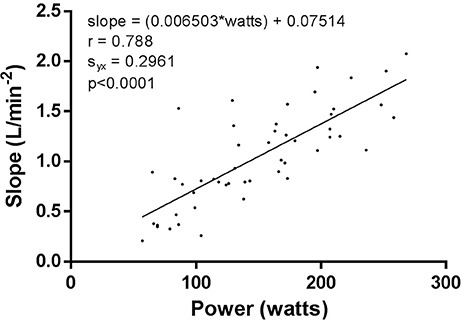
Figure 5. Linear regression of the linear onset data for power vs. slope of all subjects at all exercise intensities.

Discussion

The primary aim of this study was to compare modeling techniques for VO₂ kinetics. Therefore, we compared linear and non-linear modeling of the onset VO₂ kinetics of the phase-II approach to steady state for multiple exercise transitions. We also compared the fit of the remainder VO₂ kinetics using two different mono-exponential models. In doing so, we were able to statistically ascertain the appropriateness of using traditional non-linear modeling techniques of phase-II VO₂ kinetics to steady state vs. a new method based on the linear fit of the initial ~30 s of phase-II data for these exercise transitions.

The main findings of our results are that there was a significantly decreased error (S_y.x) and significantly increased goodness of fit for the linear regression applied to the onset VO₂ kinetics, when compared to the traditional non-linear fit. This pattern was also evident in the remainder segment of VO₂ data. Lastly, slope data (L/min⁻²) for each onset VO₂ kinetics linear regression fit was plotted against the respective cycling power (Watts) for that particular exercise transition. This was completed for each bout (30, 45, 60, 75, and 90% VT) for each participant. This data was then fitted with a linear regression. Results displayed a strong correlation between absolute power output and the speed of onset kinetics.

Currently, the majority of combined phase-II and phase-III VO₂ data are fitted using a single mono-exponential function to derive a time constant (tau, τ) to quantify the rate of the non-linear kinetics (Whipp, 1971; Linnarsson, 1974; Hughson and Morrissey, 1982; Barstow et al., 1993; Brittain et al., 2001; Spencer et al., 2013). In this context, τ represents the time to attain 63.2% of the VO₂ increment for the exercise transition. Whipp (1971) first introduced the use of the mono-exponential equation in an attempt to quantify phase-II VO₂ kinetics. Whipp soon became the leading authority on VO₂ kinetics research, publishing numerous papers (which adopted the mono-exponential method) alongside his doctoral mentor and a number of other colleagues (Whipp, 1971, 1987; Whipp and Wasserman, 1972; Whipp et al., 1982; Whipp and Ward, 1990). The use of a mono-exponential model to fit VO₂ kinetics data has since been used widely, and almost exclusively, in VO₂ kinetics to steady state (and non-steady state) research.

As previously explained, we are concerned that there has been minimal constructive criticism of single mono-exponential modeling of VO₂ data during exercise transitions to steady state. Despite more than 40 years since the original proposed research for adopting a single mono-exponential modeling of phase-II VO₂ kinetics, we have only been able to find a limited number of studies that have provided empirical evidence expressing disagreement with such practice (Hughson and Morrissey, 1982; Brittain et al., 2001; Koppo et al., 2004; MacPhee et al., 2005; Stirling et al., 2005; McNulty et al., 2015) Hughson and Morrissey (1982) showed a slower VO₂ kinetic response with a work-to-work exercise transition, compared with a rest-to-work transition. Research following this discussed a slower kinetic response (and therefore a slowed τ) with increasing exercise increments within the moderate intensity domain (Brittain et al., 2001; Koppo et al., 2004; MacPhee et al., 2005). McNulty et al. (2015) further confirmed these findings with their assessment of the VO₂ kinetic response across a broad range of sub-threshold exercise intensity transitions. Finally, Stirling et al. (2005) assessed the mono-exponential equation (amongst other concepts) on a very mathematical platform. They argued that a mono-exponential function, as part of a 3-phase approach to VO₂ kinetics data to steady state, is a vast oversimplification of the physiological responses. This is also in support of conclusions of the inferiority of the mono-exponential model. Following on from this, Stirling et al. (2005) suggested that modeling the data with a continuous differential function would be more suitable.

VO₂ Onset Segment Kinetics

Traditionally, the phase-II VO₂ kinetic response has been modeled using a mono-exponential function (Whipp, 1971). Unfortunately, this model was never expressed as a theory nor exposed to empirical validation or falsification, yet for the last 45 years it has been accepted as the sole method for use in quantifying the kinetics of the change in VO₂ during exercise transitions to steady state. Contrary to convention, our results indicated that a linear regression is a more appropriate method of modeling onset VO₂ kinetics data.

Treating kinetics as a linear function has extensive application in different disciplines of physiology. The contractile rate of force development describes the ability and rate of a muscle to develop muscular force. This is measured as the linear slope of the force-time curve (Andersen and Aagaard, 2006; Li et al., 2015) and is focused primarily on the very early onset of muscular contraction. From a mathematical viewpoint, time envelopes are used to ascertain linear fits of the muscle force changes over time to quantify the rate of muscle force development (Andersen and Aagaard, 2006; Robergs, 2014). We believe that there is important knowledge of systemic and muscle exercise physiology to be gained from quantifying these more instantaneous kinetics separate to a mono-exponential function that is dependent on the total data set, and hence we have termed this initial linear phase of the exercise transition response as onset kinetics. Such an approach not only has relevance to VO₂, but also other measures of human physiology such as heart rate, ventilation, tidal volume, breathing frequency, muscle oxygenation, central, and peripheral blood flow, etc.

The added relevance of the onset kinetics segment is seen in Figure 5 where we compared the linear onset slope data (L/min⁻²) to the respective absolute power output (Watts) of each bout. Our results indicated a significant increase in slope as absolute exercise intensity increased, and more importantly, a remarkably consistent response across all subjects and exercise intensities. This concept has been previously introduced by Robergs (2014) through a computer simulation. A linear regression was fitted to the initial 30 s of the phase-II response for six exercise transitions (VO₂ plateaus of 0.5, 1.0, 1.5, 2.0, 2.5, and 3.0 L/min). The resulting slope data for each respective exercise transition became progressively steeper at 0.18, 0.54, 0.90, 1.25, 1.61, and 1.97 L/min⁻²). The onset slope data findings in this study further support this prior work, and the uniformity of this response might reveal that the onset kinetics response is largely driven by the motor unit recruitment of the increment in exercise intensity of the exercise transition, with minimal dependence on other subject features such as age, sex, endurance fitness, etc. Such evidence reveals the possible enhanced physiological importance of the subsequent (remainder) non-linear response to steady state. The content we have discussed here reveals directions and topics for future research in VO₂ kinetics modeling.

VO₂ Remainder Segment Kinetics

If the onset kinetics is surprisingly less variable between subjects, then the greatest contribution to determining the between subjects variability of the mono-exponential model might be in the determinants to the remainder segment of the response. It is logical to conclude that the onset kinetics is primarily governed by motor unit recruitment factors. The added features of determinants to pulmonary VO₂ kinetics as defined by Robergs (2014) may be revealed in this slower non-linear response. Such factors include: cardiac output, muscle blood flow, muscle fiber type, muscle VO₂ kinetics, and ventilation. Further investigation into the remainder kinetics (as well as the linear onset kinetics) in different and special populations, could have added benefit to understanding the VO₂ kinetic response.

A New Theory of VO₂ Kinetics during Exercise Transitions to Steady State

The debate surrounding the physiological basis of VO₂ on-kinetics to steady state is ongoing. There has been much experimentation and commentary discussing O₂ delivery and utilization (Hughson and Morrissey, 1982; MacDonald et al., 1997; Grassi et al., 1998; Burnley et al., 2000; Koppo et al., 2004), motor unit recruitment (Cleuziou et al., 2004), muscle fiber type (Barstow et al., 2000), muscle phosphocreatine (Binzoni et al., 1992; Barstow, 1994; Rossiter et al., 2002), and oxidative phosphorylation (Korzeniewski and Zoladz, 2006) in an attempt to formulate a conclusive theoretical framework for explaining VO₂ kinetics to steady state. During this time, a mono-exponential function has been widely accepted to model the phase-II VO₂ kinetics as a first-order linear system (Whipp, 1971). This has also come under enquiry, as its basis of predicting phase-II kinetic behavior has been shown to be inaccurate in some experimentation (Hughson and Morrissey, 1982; Brittain et al., 2001; Koppo et al., 2004; McNulty et al., 2015). As well, the use of a potentially over-simplified model as such combines numerous contributing responses into a single parameter estimate which is likely not attributed to distinct physiological systems (Bakker et al., 1980). Despite this, the mono-exponential equation (and τ) has been continually applied to the majority of VO₂ kinetics data processing. As well, the mono-exponential application has proceeded into other aspects of kinetics processing in regards to exercise transitions to steady state. Intramuscular phosphocreatine kinetics (Rossiter et al., 1999), de-oxygenated hemoglobin kinetics (DeLeroy et al., 2003; MacPhee et al., 2005), and muscle blood flow kinetics (MacPhee et al., 2005) have all been processed using a mono-exponential formula, again without any critical empirical investigation.

We propose that the VO₂ response to exercise transitions to steady state is more complex than a mono-exponential function. It is clear that there is still much research to be completed concerning the physiological processes, and appropriate modeling, of the VO₂ kinetic response to exercise transitions to steady state. The question needs to be raised: how can we blindly follow a mathematical model of such physiological responses, when there is still ongoing debate regarding the underlying physiology itself? As scientists, we need to be open to new fundamental ideas and compelling research as they present themselves.

Conclusions and Recommendations

Historically, VO₂ kinetics research has been focused almost primarily on complete phase-II non-linear modeling of exercise transition to steady state. This may be viewed as a somewhat over-simplification of the response kinetics, more attuned to fitting the data to a chosen model rather than ascertaining the best model to fit the data. Our results indicated that a linear regression fitted to the initial (first ~30 s of exercise transition) phase-II kinetics is more appropriate than a mono-exponential function. Further investigation into the VO₂ kinetic response, as well as other similarly modeled responses (phosphocreatine, de-oxygenated hemoglobin, limb blood flow), and in varied populations, may yield a better understanding of the complimentary effects of the numerous physiological responses underpinning the VO₂ kinetic response to exercise transitions to steady state.

Ethics Statement

This study was carried out in accordance with the recommendations of the Charles Sturt University Human Research Ethics Committee with written informed consent from all subjects. All subjects gave written informed consent in accordance with the Declaration of Helsinki. The protocol was approved by the Human Research Ethics Committee.

Author Contributions

CM and RR designed the study. CM collected data. CM and RR analyzed the data. CM wrote the manuscript. RR edited the manuscript. CM and RR read and approved the final manuscript.

Funding

All funding for this research project was sourced from the lead author's Australian Postgraduate Award scholarship.

Conflict of Interest Statement

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

References

Andersen, L. L., and Aagaard, P. (2006). Influence of maximal muscle strength and intrinsic muscle contractile properties on contractile rate of force development. Eur. J. Appl. Physiol. 99, 46–52. doi: 10.1007/s00421-005-0070-z

PubMed Abstract | CrossRef Full Text | Google Scholar

Astorino, T. A., Robergs, R. A., Ghiasvand, F., Marks, D., and Burns, S. (2000). Incidence of the oxygen plateau during exercise testing to volitional fatigue. J. Exerc. Physiol. Online 29, 60–67.

Google Scholar

Astorino, T. A., Willey, J., Kinnahan, J., Larsson, S. M., Welch, H., and Dalleck, L. C. (2005). Elucidating determinants of the plateau in oxygen consumption at VO_2max. Br. J. Sports Med. 39, 655–660. doi: 10.1136/bjsm.2004.016550

PubMed Abstract | CrossRef Full Text | Google Scholar

Bakker, H. K., Struikenamp, R. S., and Vries, G. A. D. (1980). Dynamics of ventilation, heart rate, and gas exchange: sinusoidal and impulse work loads in man. J. Appl. Physiol. 48, 289–301.

PubMed Abstract | Google Scholar

Barstow, T. J. (1994). Characterization of VO₂ kinetics during heavy exercise. Med. Sci. Sports Exerc. 26, 1327–1334. doi: 10.1249/00005768-199411000-00006

PubMed Abstract | CrossRef Full Text | Google Scholar

Barstow, T. J., Casaburi, R. R., and Wasserman, K. K. (1993). O₂ uptake kinetics and the O₂ deficit as related to exercise intensity and blood lactate. J. Appl. Physiol. 75, 755–762.

PubMed Abstract | Google Scholar

Barstow, T. J., Jones, A. M., Nguyen, P. H., and Casaburi, R. (1996). Influence of muscle fiber type and pedal frequency on oxygen uptake kinetics of heavy exercise. J. Appl. Physiol. 81, 1642–1650.

PubMed Abstract | Google Scholar

Barstow, T. J., Jones, A. M., Nguyen, P. H., and Casaburi, R. (2000). Influence of muscle fibre type and fitness on the oxygen uptake/power output slope during incremental exercise in humans. Exp. Physiol. 85, 109–116. doi: 10.1111/j.1469-445X.2000.01942.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Binzoni, T., Ferretti, G., Schenker, K., and Cerretelli, P. (1992). Phosphocreatine hydrolysis by 31P-NMR at the onset of constant-load exercise in humans. J. Appl. Physiol. 73, 1644–1649.

PubMed Abstract | Google Scholar

Borsheim, E., and Bahr, R. (2003). Effect of exercise intensity, duration and mode on post-exercise oxygen consumption. Sports Med. 33, 1037–1060. doi: 10.2165/00007256-200333140-00002

PubMed Abstract | CrossRef Full Text | Google Scholar

Brittain, C. J., Rossiter, H. B., Kowalchuk, J. M., and Whipp, B. J. (2001). Effect of prior metabolic rate on the kinetics of oxygen uptake during moderate-intensity exercise. Eur. J. Appl. Physiol. 86, 125–134. doi: 10.1007/s004210100514

PubMed Abstract | CrossRef Full Text | Google Scholar

Buchfuhrer, M. J., Hansen, J. E., Robinson, T. E., Sue, D. Y., Wasserman, K., and Whipp, B. J. (1983). Optimizing the exercise protocol for cardiopulmonary assessment. J. Appl. Physiol. Respir. Environ. Exerc. Physiol. 55, 1558–1564.

PubMed Abstract | Google Scholar

Burnley, M., Jones, A. M., Carter, H., and Doust, J. H. (2000). Effects of prior heavy exercise on phase II pulmonary oxygen uptake kinetics during heavy exercise. J. Appl. Physiol. 89, 1387–1396.

PubMed Abstract | Google Scholar

Casaburi, R. R., Barstow, T. J., Robinson, T. T., and Wasserman, K. K. (1989). Influence of work rate on ventilatory and gas exchange kinetics. J. Appl. Physiol. 67, 547–555.

PubMed Abstract | Google Scholar

Cleuziou, C., Perrey, S., Borrani, F., Lecoq, A. M., Courteix, D., Germain, P., et al. (2004). VO₂ and EMG activity kinetics during moderate and severe constant work rate exercise in trained cyclists. Can. J. Appl. Physiol. 29, 758–772. doi: 10.1139/h04-049

PubMed Abstract | CrossRef Full Text | Google Scholar

DeLeroy, D. S., Kowalchuk, J. M., and Paterson, D. H. (2003). Relationship between pulmonary O₂ uptake kinetics and muscle deoxygenation during moderate-intensity exercise. J. Appl. Physiol. 95, 113–120. doi: 10.1152/japplphysiol.00956.2002

PubMed Abstract | CrossRef Full Text | Google Scholar

Exercise and Sports Science Australia (2014). Adult Pre-Exercise Screening Tool. Available online at: https://www.essa.org.au/wp-content/uploads/2011/09/Screen-tool-version-v1.1.pdf

Gaskill, S. E., Ruby, B. C., Walker, A. J., Sanchez, G. A., Serfass, R. C., and Leon, A. S. (2001). Validity and reliability of combining three methods to determine ventilatory threshold. Med. Sci. Sport. Exerc. 33, 1841–1848. doi: 10.1097/00005768-200111000-00007

PubMed Abstract | CrossRef Full Text | Google Scholar

Grassi, B., Gladden, L. B., Samaja, M., Stary, C. M., and Hogan, M. C. (1998). Faster adjustment of O₂ delivery does not affect VO₂ on-kinetics in isolated in situ canine muscle. J. Appl. Physiol. 85, 1394–1403.

PubMed Abstract | Google Scholar

Hickson, R. C., Bomze, H. A., and Holloszy, J. O. (1978). Faster adjustment of O₂ uptake to the energy requirement of exercise in the trained state. J. Appl. Physiol. 44, 877–881.

PubMed Abstract | Google Scholar

Hughson, R. L., and Morrissey, M. (1982). Delayed kinetics of respiratory gas exchange in the transition from prior exercise. J. Appl. Physiol. 52, 921–929.

PubMed Abstract | Google Scholar

Jones, A. M., and Poole, D. C. (2005). Oxygen Uptake Kinetics in Sport, Exercise and Medicine. Oxon: Routledge.

Google Scholar

Kim, D., and Robergs, R. A. (2012). Validation of a new mixing chamber system for breath-by-breath indirect calorimetry. Appl. Physiol. Nutr. Metab. 37, 157–166. doi: 10.1139/h11-147

PubMed Abstract | CrossRef Full Text | Google Scholar

Kirk, R. E. (2010). “Latin square design” in The Corsini Encyclopedia of Psychology, Vol. 2, 4th Edn, eds I. B. Weiner and W. E. Craighead (Hoboken, NJ: John Wiley), 915–916.

Google Scholar

Koppo, K., Bouckaert, J., and Jones, A. (2004). Effects of training status and exercise intensity on phase II VO₂ kinetics. Med. Sci. Sport Exer. 36, 225–232. doi: 10.1249/01.MSS.0000113473.48220.20

PubMed Abstract | CrossRef Full Text | Google Scholar

Korzeniewski, B., and Zoladz, J. A. (2006). Biochemical background of the VO₂ on-kinetics in skeletal muscle. J. Physiol. Sci. 56, 1–12. doi: 10.2170/physiolsci.M93

PubMed Abstract | CrossRef Full Text | Google Scholar

Li, J., Yongjin, Z., Zheng, Y., and Li Guanglin, L. (2015). An attempt to bridge muscle architecture dynamics and its instantaneous rate of force development using ultrasonography. Ultrasonics 61, 71–78. doi: 10.1016/j.ultras.2015.03.009

PubMed Abstract | CrossRef Full Text | Google Scholar

Linnarsson, D. (1974). Dynamics of pulmonary gas exchange and heart rate changes at start and end of exercise. Acta Physiol. Scand. 66, 1–68.

Google Scholar

MacDonald, M., Pedersen, P. K., and Hughson, R. L. (1997). Acceleration of VO₂ kinetics in heavy submaximal exercise by hypoxia and prior high-intensity exercise. J. Appl. Physiol. 83, 1318–1325.

PubMed Abstract | Google Scholar

MacPhee, S. L., Shoemaker, J. K., Paterson, D. H., and Kowalchuk, J. M. (2005). Kinetics of O2 uptake, leg blood flow, and muscle deoxygenation are slowed in the upper compared with lower region of the moderate-intensity exercise domain. J. Appl. Physiol. 99, 1822–1834. doi: 10.1152/japplphysiol.01183.2004

PubMed Abstract | CrossRef Full Text | Google Scholar

McNulty, C. R., Robergs, R. A., and Morris, D. (2015). Influence of increment magnitude and exercise intensity on VO₂ kinetics, time to steady state, and muscle oxygenation. J. Exerc. Physiol. Online 18, 37–58.

Google Scholar

Nery, L. E., Wasserman, K., Andrews, J. D., Huntsman, D. J., Hansen, J. E., and Whipp, B. J. (1982). Ventilatory and gas exchange kinetics during exercise in chronic airways obstruction. J. Appl. Physiol. 53, 1594–1602.

PubMed Abstract | Google Scholar

Robergs, R. A. (2014). A critical review of the history of low- to moderate-intensity steady-state VO2 kinetics. Sports Med. 44, 641–653. doi: 10.1007/s40279-014-0161-2

PubMed Abstract | CrossRef Full Text | Google Scholar

Rossiter, H. B., Ward, S. A., Doyle, V. L., Howe, F. A., Griffiths, J. R., and Whipp, B. J. (1999). Inferences from pulmonary O₂ uptake with respect to intramuscular [phosphocreatine] kinetics during moderate exercise in humans. J. Physiol. 518, 921–932. doi: 10.1111/j.1469-7793.1999.0921p.x

PubMed Abstract | CrossRef Full Text | Google Scholar

Rossiter, H. B., Ward, S. A., Howe, F. A., Kowalchuk, J. M., Griffiths, J. R., and Whipp, B. J. (2002). Dynamics of the intramuscular 31P-MRS spectrum and O₂ uptake during exercise: inferences from the split Pi peak. J. Appl. Physiol. 93, 2059–2069. doi: 10.1152/japplphysiol.00446.2002

PubMed Abstract | CrossRef Full Text

Spencer, M. D., Keir, D. A., Nederveen, J. P., Murias, J. M., Kowalchuk, J. M., and Paterson, D. H. (2013). Prolonged moderate-intensity exercise oxygen uptake response following heavy-intensity priming exercise with short- and longer-term recovery. Appl. Physiol. Nutr. Metab. 38, 566–573. doi: 10.1139/apnm-2012-0244

PubMed Abstract | CrossRef Full Text | Google Scholar

Spiro, S. G. (1977). Exercise testing in clinical medicine. Br. J. Dis. Chest. 71, 145–172. doi: 10.1016/0007-0971(77)90106-1

PubMed Abstract | CrossRef Full Text | Google Scholar

Stirling, J. R., Zakynthinaki, M. S., and Saltin, B. (2005). A model of oxygen uptake kinetics in response to exercise: including a means of calculating oxygen demand/deficit/debt. Bull. Math. Biol. 67, 989–1015. doi: 10.1016/j.bulm.2004.12.005

PubMed Abstract | CrossRef Full Text | Google Scholar

Weltman, A., and Katch, V. (1976). Min-by-min respiratory exchange and oxygen uptake kinetics during steady-state exercise in subjects of high and low max VO₂. Res. Q. 47, 490–498.

PubMed Abstract | Google Scholar

Whipp, B. J. (1971). Rate constant for the kinetics of oxygen uptake during light exercise. J. Appl. Physiol. 3, 261–263.

Google Scholar

Whipp, B. J. (1987). Dynamics of pulmonary gas exchange. Circulation 76, 18–28.

PubMed Abstract | Google Scholar

Whipp, B. J., and Ward, S. A. (1990). Physiological determinants of pulmonary gas exchange kinetics during exercise. Med. Sci. Sports Exerc. 22, 62. doi: 10.1249/00005768-199002000-00011

PubMed Abstract | CrossRef Full Text | Google Scholar

Whipp, B. J., and Wasserman, K. (1972). Oxygen uptake kinetics for various intensities of constant-work load. J. Appl. Physiol. 33, 351–356.

PubMed Abstract | Google Scholar

Whipp, B. J., Ward, S. A., Lamarra, N., Davis, J. A., and Wasserman, K. (1982). Parameters of ventilatory and gas exchange dynamics during exercise. J. Appl. Physiol. 52, 1506–1513.

PubMed Abstract | Google Scholar

Yoon, B. K., Kravitz, L., and Robergs, R. (2007). VO_2max, protocol duration, and the VO₂ plateau. Med. Sci. Sport Exerc. 39, 1186–1192. doi: 10.1249/mss.0b13e318054e304

PubMed Abstract | CrossRef Full Text | Google Scholar