A commentary on
Chloride Regulation: A Dynamic Equilibrium Crucial for Synaptic Inhibition
by Doyon, N., Vinay, L., Prescott, S. A., and De Koninck, Y. (2016). Neuron 89, 1157-1172.
The recent review by Doyon et al. (2016) is for the main part an excellent description of many important aspects of neuronal chloride regulation and will be of good use to many scientists interested in synaptic function. Nevertheless, some information given on the role of the K+ Cl− cotransporter 2 (KCC2) is likely to be misleading. While proposing an explanation for the controversial findings by Glykys et al. (2014) that blockers of cation-chloride cotransporters did not affect the basal intracellular Cl− concentration ([Cl−]i), contrary to expectations from previous transporter manipulations (see e.g., references in Ben-Ari, 2014; Kaila et al., 2014), Doyon et al. (2016) suggest that this may be due to the small degree of inhibitory synaptic activity in the preparation used, with extremely low Cl− load. In their explanation, they give an equation for the equilibrium relation between Cl− flux through an inhibitory (Cl−) conductance (ginh) and the Cl− transported by KCC2. On basis of this equation, the conclusion is that the Cl− equilibrium potential “ECl is sensitive to changes in KCC2 activity (gKCC2) only when Cl− load (ginh) is large.” This conclusion, however, cannot be justified on basis of the relation between Cl− flux through channels and Cl− transported by KCC2. (The equation given by Doyon et al. is not correctly formulated, although the reason for their claim may not depend on this mistake).
For an explanation of our point of view, consider a hypothetical cell with Cl− transport across the outer membrane only via Cl− selective channels and KCC2. At equilibrium, the amount (mol/s) of Cl− transported by the channels must be equal, but opposite, to that transported by KCC2. We thus formulate the relation:
where ICl is Cl− current, F is the Faraday constant and UKCC2 is the driving force for transport by KCC2. gKCC2 is a proportionality factor that may be thought of as an “apparent conductance,” and should reflect the number of transporters in the membrane as well as the transport rate of the individual transporter molecules at fixed K+ and Cl− concentrations, similarly as ICl depends on the Cl− conductance (gCl) which reflects the number of Cl− channels as well as the conductance of individual channels. (gKCC2 is, however, not a conductance in the usual electrical sense). Equation (1) may be reformulated, in several steps, for clarity:
where Vm is membrane potential, R the gas constant, T temperature (in K), EK the K+ equilibrium potential and Cl− and K+ concentrations are given within brackets with subscripts i and o for inside and outside, respectively.
We may use Equation (4) to illustrate the relation between ECl and gKCC2 at various levels of gCl. (Assume that K+ concentrations and membrane potential are fixed, as controlled by other factors, such as the cellular Na+-K+-ATPase, not discussed here). As can be seen in Figure 1A, contrary to the claim by Doyon et al. (2016), ECl is only weakly dependent on KCC2 transport capacity at high Cl− conductance, while it depends strongly on KCC2 when Cl− conductance is low. At the extremes, when gKCC2 = 0, then ECl = Vm and when gCl = 0, then ECl = EK.
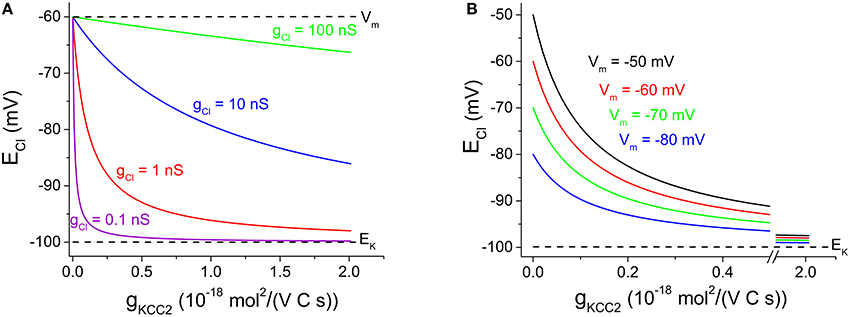
Figure 1. Cl− equilibrium potential — dependence on KCC2 transporter capacity at various Cl− conductance and various membrane potentials. (A) ECl vs. gKCC2 with EK fixed at −100 mV and Vm at −60 mV. gCl as indicated. Note that ECl dependence on gKCC2 is reduced with increased gCl. (B) ECl vs. gKCC2 with EK fixed at −100 mV and gCl at 1 nS. Vm as indicated. Note that ECl dependence on gKCC2 increases when Vm changes in positive direction. Note the x-axis break between 0.5 and 1.9 10−18 mol2/(V C s), to clearer illustrate the steeply decaying region of the curves. Justification of illustrated parameter ranges: The gCl range (in A) was chosen to include cells with a low gCl as evident from the high membrane resistance (Johansson et al., 1995) as well as cells with a high gCl (very low input resistance dominated by inhibitory conductances; Destexhe et al., 2003). The gKCC2 range (in A,B) shown likely covers the capacity for most central neurons: When gKCC2 = 1 10−18 mol2/(V C s), KCC2-mediated transport modeled as described by Karlsson et al. (2011) may reduce [Cl−]i from 20 mM to ~5 mM with approximated time constants of 0.85, 6.8, and 55 s for spherical cells of radius 5, 10, and 20 μm, respectively, assuming 50% cytosolic volume and no other Cl− transport/leak. Experimentally observed [Cl−]i recovery is slower or comparable (Berglund et al., 2006; Lee et al., 2011; Pellegrino et al., 2011).
The relation between ECl (and thus [Cl−]i) and transporter capacity has some bearing for the interpretation of the controversial findings by Glykys et al. (2014). Contrary to the suggestion by Doyon et al. (2016), Figure 1A shows that transporter block is expected to affect basal [Cl−]i especially under conditions when gCl is low. Thus, other explanations than a low gCl must be sought for the controversial findings of Glykys et al. (2014). An increased gCl, perhaps due to increased non-specific leak, could in theory contribute to the limited effect of KCC2 blocker on [Cl−]i.
On the other hand, the lack of excitatory synaptic input may contribute to a reduced sensitivity to transport block. In equation (4) above, a steady excitatory input may be represented simply by a more positive Vm, if we assume that EK is still maintained (by the cellular Na+-K+-ATPase). Figure 1B shows that although ECl is more positive, the dependence of ECl on gKCC2 is clearly stronger at more positive Vm.
As described, an increased gCl (Figure 1A) or a more positive Vm (Figure 1B) will change ECl in positive direction. This may be exploited experimentally e.g., by combining GABA or glycine application with depolarization to achieve a dramatic change in ECl and rise in [Cl−]i (Karlsson et al., 2011). With such manipulations, it is obvious that the neuronal transporter capacity cannot prevent the changes in ECl and in steady-state [Cl−]i.
Doyon et al. (2016) also note the neglected problem of apparent (illusory) conductance decrease based on recordings at different holding potentials when [Cl−]i is changing, a problem which has recently been described in more detail by Yelhekar et al. (2016). It may be noted that experimentally, the effects of a changing [Cl−]i on apparent conductance may be separated from true changes in conductance by using rapid voltage-ramp techniques (Karlsson et al., 2011; Yelhekar et al., 2016).
Author Contributions
SJ made the computations and paper writing. SJ, TY, and MD contributed to the ideas and final content.
Conflict of Interest Statement
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Acknowledgments
Our studies are supported by the Swedish Research Council (grant no. 22292), by Gunvor och Josef Anérs Stiftelse and by Umeå University Medical Faculty (Insamlingsstiftelsen, Karin och Harald Silvanders fond, and Leila och Bertil Ehrengrens fond).
References
Ben-Ari, Y. (2014). The GABA excitatory/inhibitory developmental sequence: a personal journey. Neuroscience 279, 187–219. doi: 10.1016/j.neuroscience.2014.08.001
Berglund, K., Schleich, W., Krieger, P., Loo, L. S., Wang, D., Cant, N. B., et al. (2006). Imaging synaptic inhibition in transgenic mice expressing the chloride indicator, Clomeleon. Brain Cell Biol. 35, 207–228. doi: 10.1007/s11068-008-9019-6
Destexhe, A., Rudolph, M., and Paré, D. (2003). The high-conductance state of neocortical neurons in vivo. Nat. Rev. Neurosci. 4, 739–751. doi: 10.1038/nrn1198
Doyon, N., Vinay, L., Prescott, S. A., and De Koninck, Y. (2016). Chloride regulation: a dynamic equilibrium crucial for synaptic inhibition. Neuron 89, 1157–1172. doi: 10.1016/j.neuron.2016.02.030
Glykys, J., Dzhala, V., Egawa, K., Balena, T., Saponjian, Y., Kuchibhotla, K. V., et al. (2014). Local impermeant anions establish the neuronal chloride concentration. Science 343, 670–675. doi: 10.1126/science.1245423
Johansson, S., Sundgren, A., and Klimenko, V. (1995). Graded action potentials generated by neurons in rat hypothalamic slices. Brain Res. 700, 240–244. doi: 10.1016/0006-8993(95)00969-W
Kaila, K., Price, T. J., Payne, J. A., Puskarjov, M., and Voipio, J. (2014). Cation-chloride cotransporters in neuronal development, plasticity and disease. Nat. Rev. Neurosci. 15, 637–654. doi: 10.1038/nrn3819
Karlsson, U., Druzin, M., and Johansson, S. (2011). Cl− concentration changes and desensitization of GABAA and glycine receptors. J. Gen. Physiol. 138, 609–626. doi: 10.1085/jgp.201110674
Lee, H. H. C., Deeb, T. Z., Walker, J. A., Davies, P. A., and Moss, S. J. (2011). NMDA receptor activity downregulates KCC2 resulting in depolarizing GABAA receptor–mediated currents. Nat. Neurosci. 14, 736–743. doi: 10.1038/nn.2806
Pellegrino, C., Gubkina, O., Schaefer, M., Becq, H., Ludwig, A., Mukhtarov, M., et al. (2011). Knocking down of the KCC2 in rat hippocampal neurons increases intracellular chloride concentration and compromises neuronal survival. J. Physiol. 589, 2475–2496. doi: 10.1113/jphysiol.2010.203703
Keywords: chloride, Cl− channel, K+ Cl− cotransporter 2, conductance, membrane potential, synaptic inhibition, Cl− concentration, equilibrium potential
Citation: Johansson S, Yelhekar TD and Druzin M (2016) Commentary: Chloride Regulation: A Dynamic Equilibrium Crucial for Synaptic Inhibition. Front. Cell. Neurosci. 10:182. doi: 10.3389/fncel.2016.00182
Received: 22 April 2016; Accepted: 06 July 2016;
Published: 19 July 2016.
Edited by:
Marco Martina, Northwestern University, USAReviewed by:
Yves De Koninck, Laval University, CanadaCopyright © 2016 Johansson, Yelhekar and Druzin. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) or licensor are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Staffan Johansson, c3RhZmZhbi5vLmpvaGFuc3NvbkB1bXUuc2U=
 Staffan Johansson
Staffan Johansson Tushar D. Yelhekar
Tushar D. Yelhekar Michael Druzin
Michael Druzin