Department of Neurobiology, Institute of Life Sciences, and Interdisciplinary Center for Neural Computation, Hebrew University, Jerusalem, Israel
Recent studies have demonstrated that the membrane potential of Purkinje cells is bi-stable and that this phenomenon underlies bi-modal simple spike firing. Membrane potential alternates between a depolarized state, that is associated with spontaneous simple spike firing (up state), and a quiescent hyperpolarized state (down state). A controversy has emerged regarding the relevance of bi-stability to the awake animal, yet recordings made from behaving cat Purkinje cells have demonstrated that at least 50% of the cells exhibit bi-modal firing. The robustness of the phenomenon in vitro or in anaesthetized systems on the one hand, and the controversy regarding its expression in behaving animals on the other hand suggest that state transitions are under neuronal control. Indeed, we have recently demonstrated that synaptic inputs can induce transitions between the states and suggested that the role of granule cell input is to control the states of Purkinje cells rather than increase or decrease firing rate gradually. We have also shown that the state of a Purkinje cell does not only affect its firing but also the waveform of climbing fiber-driven complex spikes and the associated calcium influx. These findings call for a reconsideration of the role of Purkinje cells in cerebellar function. In this manuscript we review the recent findings on Purkinje cell bi-stability and add some analyses of its effect on the regularity and variability of Purkinje cell activity.
Like most neurons, Purkinje cells (PCs) are classically described as stable electrical elements where the resting potential is continuously interrupted by synaptic potentials. Once the synaptic potentials reach a certain threshold, an action potential is generated. This somewhat simplistic description implies that the firing rate of a cell mirrors its synaptic input. This view of PCs has recently been challenged by the finding that they operate as bi-stable elements. PCs seem to have two stable levels of membrane potential: a hyperpolarized state that is devoid of simple spike firing (down state), and a depolarized state in which the cell spontaneously fires at high frequencies even in the absence of any synaptic input (up state) (Williams et al., 2002
; Loewenstein et al., 2005
; Fernandez et al., 2007
). Hence, PC firing rate does not reflect a simple summation of its inputs. This alternative view of PCs is not commonly accepted. De Zeeuw and colleagues reported that although bimodal distributions of firing rate are frequently encountered in anesthetized animals, PCs switch to a continuous firing mode once the anesthesia is removed (Schonewille et al., 2006
). Conversely, recordings from awake, restrained cats demonstrated that ∼50% of the cells exhibit bi-modal firing dynamics (Yartsev et al., 2009
), and preliminary results also documented bi-modal firing of Purkinje cells in freely moving rats (Lev et al., 2006
). One way to reconcile these contradictory reports is to assume that the balance between continuous and bi-modal firing is modulated by the behavioral state of the animal such as exploration, stress or alertness. In the following we describe the phenomenon of bi-stability and its effect on the responses of PCs to synaptic inputs.
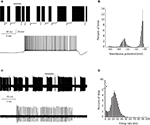
PC bi-stability is readily observed in intra- and extra-cellular recordings in both in vitro and in vivo preparations. In slice preparations it is occasionally periodic, where the state durations are on a time scale of seconds (Figure 1
A). An epoch of up state (Figure 1
A lower trace) starts with a slow membrane depolarization that upon reaching threshold elicits a prolonged firing period characterized by initial fast rate that slowly settles to a steady state firing frequency with different degrees of regularity (see below). The firing epoch terminates abruptly where the membrane potential is rapidly hyperpolarized below the original level. The membrane potential (after omitting action potentials) attains two distinct levels. In the example shown in Figure 1
A an average value of −57 mV was observed during the down state, whereas the depolarized up state was ∼−47 mV (Figure 1
B). Similar behavior can be observed when the activity of a single PC is extracellularly monitored (Figure 1
C). The alternation between firing and quiescent epochs on time scales of seconds is a robust feature of the activity. As with the intracellular recording, a firing epoch starts abruptly with a high firing rate that quickly settled to a somewhat lower frequency of various regularities. In contrast to the intracellular recording, here the bi-stability is quantified by calculating the instantaneous firing frequency. The distribution of the frequencies (Figure 1
D) shows that almost half of the time the cell is quiescent, whereas when it is active, it tends to fire at 20–30 Hz. Several lines of evidence support the intrinsic origin of PC bi-stability. First, brief intracellular current injections are sufficient to induce state transitions (see Figure 3
). Second, a dc current injection that hyperpolarizes the membrane potential maintains the cell in the down state and does not reveal any bi-modal inputs (Loewenstein et al., 2005
), and third, the spontaneous firing rate of PCs is not altered by synaptic blockers (Cerminara and Rawson, 2004
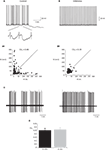
). Yet, synaptic inputs may still affect the timing of spikes. As intrinsic firing is expected to be regular, we estimated the firing regularity during up states using CV2 analysis (Holt et al., 1996
). As shown in Figure 2
, simple spike firing is rather regular both in vitro (Figure 2
A) and in vivo (Figure 2
C). The mean CV2 value in vitro was 0.25 ± 0.03, indistinguishable from the CV2 values obtained in vivo (0.26 ± 0.03, p > 0.5; Figure 2
D). The regularity of firing was also examined by plotting the relationship between consecutive ISIs (Figure 2
A1). Most of the ISIs were in the range of 20–40 ms however occasional prolonged ISIs were observed. These prolonged ISIs were accompanied by hyperpolarizations, suggesting the involvement of spontaneous IPSPs (see inset in Figure 2
A). To test this possibility we measured the effect of gabazine (0.5 μM) on firing regularity. Although the maximal firing frequency was not affected by gabazine, a significant increase in firing regularity was observed (Hausser and Clark, 1997
). In the example shown in Figure 2
the prolonged ISIs and the accompanied hyperpolarizations (Figure 2
A) were absent in the presence of GABAzine (Figure 2
B), and accordingly the CV2 value was reduced from 0.45 to 0.19. These observations lead to the conclusion that the firing during the up state in vitro is intrinsically generated and can be modulated by inhibitory synaptic potentials.
Figure 1. PC bi stability recorded intracellularly in a cerebellar slice (A) and extracellularly in a ketamine anesthetized rat (C) (see Tal et al. , 2008
for methods). An epoch of up state (marked by horizontal bars in the upper traces) is displayed on an extended time scale in the lower traces in (A) and (C). (B,D) Show the percentage of time spent in each membrane potential (B) and instantaneous frequency (D).
Figure 2. The regularity of PC firing during upstate. (A,B) Traces showing the up state firing of a PC in a slice preparation (see Tal et al., 2008
for methods) before (A) and after (B) application of GABAzine (0.5 μM). Inset in (A) shows examples of the IPSPs in the original trace in an expanded scale. (A1,B1) the relations between consecutive ISIs before (A1) and after (B1) GABAzine application constructed from 2 min of recordings of the same cells as in (A) and (B). (C) Two extreme examples of the most non-regular (left) and the most regular (right) units that were recorded in an anesthetized rat in vivo. (D) The average CV2 values obtained in vitro and in vivo n = 12 and 6, respectively.
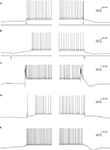
The intrinsic bi-modality of simple spikes implies that PC inputs to the cerebellar nuclei (CN) are binary signals and therefore can report the PC state but not individual synaptic events. Nonetheless, for these signals to be meaningful, the transitions between the states should be governed by synaptic inputs. Indeed, a variety of input signals are extremely efficient in inducing state transitions (Figure 3
). Interestingly, most of these signals can induce both upward and downward shifts. For example, a brief hyper- or depolarizing current injection during a down state will shift the membrane potential to an up state whereas the same current during an up state will shift the cell to a down state (Figures 3
A,B). Similarly, climbing fiber (CF) inputs can induce bi-directional state transitions (Figure 3
C). In fact transitions are so easily induced that they occasional occur spontaneously (Figure 3
E). In a recent study we showed that the mossy fiber – granule cell input can also induce bi-directional transitions (Jacobson et al., 2008
). Transitions from a down to an up state occurred when an excitatory granule cell input was activated, while transitions from an up to a down state occurred when the molecular layer interneurons were activated (Figure 3
D). We found that the probability of transitions induced by granule cell inputs is tightly linked to EPSP amplitude. At high stimulation intensity that generated an EPSP of ∼15 mV and usually elicited an action potential, transitions from down state to up state occurred at 74% and transitions from up state to down state at 62%. At low stimulation intensity that generated an EPSP of ∼8 mV and only occasionally an action potential, transitions from down state to up state occurred at 19% and transitions from up state to down state at 3%. It is important to note that transitions were not limited to stimulations that directly triggered an action potential.
Figure 3. State transitions can be induced by current injections (A,B) synaptic inputs (C,D) or can occur spontaneously (E). (A) A 35-ms depolarizing current pulse of 100 pA induced a transition to an up state (left) and to a down state (right). (B) The same as in (A) for a hyperpolarizing current pulse. (C) Transitions induced by CSs that were evoked by stimulating the white matter just below the recorded PC. (D) State transitions induced by stimulating the granule cells (left) and the molecular layer interneurons (MLIs; right). For granule cells activation the stimulating electrode was placed at the granular layer just below the recorded PC and for activation of the MLIs the electrode was placed either in the molecular layer or in the granular layer laterally to the recorded PC. (E) Spontaneous transitions. All traces were obtained with whole cell recordings in slice preparations (see Tal et al., 2008
for methods).
There is ample evidence to support the idea that a significant excitatory input to PCs is organized along the ascending branch of the granule cell axons whereas the inhibitory input, presumably activated by the same mossy fibers, are likely to be most effective at the perimeter of the excitatory input (Cohen and Yarom, 1998
; Isope and Barbour, 2002
; Sims and Hartell, 2005
, 2006
; Rokni et al., 2007
; Lu et al., 2009
; Walter et al., 2009
). Thus, a mossy fiber input can, theoretically, generate a spatially organized patch of PCs in their up state. It follows that the role of the mossy fibers input onto PCs is to induce state transitions rather than control their firing rate or spike timing.
As described above the climbing fiber (CF) input into PCs can induce state transitions. This is not surprising since this unique input is one of the most powerful synapses in the nervous system. Activation of the CF input results in an all-or-none response known as a complex spike (CS) (Eccles et al., 1966
). In contrast to the brief simple spike, the complex spike consists of a large initial spike followed by a train of secondary spikes or wavelets. This complex response, which occasionally appears in the form of a voltage ripple, is triggered by an enormous synaptic potential generated by the activation of hundreds of synaptic releasing sites (Rossi et al., 1993
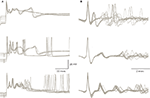
). The exceptionally high quantal content of this synapse ensures very small variations in the amplitude of the synaptic current triggered by a single pre-synaptic action potential. Nevertheless, as shown in Figure 4
A the response is state dependent, being longer at the depolarized state. The trial by trial changes in the CS waveform, despite the invariability of the underlying synaptic potential, suggests that it is governed by the cell’s current excitability state. Similar variation in the CS waveform has been observed in in-vivo extracellular recordings (Figure 4
B). These variations, which have been previously reported (Gilbert and Thach, 1977
; Llinas and Sugimori, 1980
; Callaway et al., 1995
; Hansel and Linden, 2000
; Servais et al., 2004
; Khaliq and Raman, 2005
; Loewenstein et al., 2005
; Sacconi et al., 2008
; Zagha et al., 2008
) may reflect the effect of the PC state on the response. However, under in vivo conditions other parameters contribute to these variations. For example it has been recently demonstrated that the prolonged olivary action potential generates a short burst of action potentials that propagates all the way to the cerebellar cortex. The number of action potentials will undoubtedly change the CS waveform although this effect will be diminished due to synaptic depression (Eccles et al., 1966
; Dittman and Regehr, 1998
). Nevertheless the state of the PC is bound to exert its modulatory effect on the response whether it is evoked by a single spike or a burst of spikes. Furthermore, it is likely that long term changes in the synaptic potential such as different levels of potentiation or depression of the CF–PC synapses contribute to alterations of the CS waveform on a longer time scale (Hashimoto and Kano, 1998
; Hansel and Linden, 2000
; Weber et al., 2003
).
Figure 4. Variability of the CS waveform. (A) Examples of CSs recorded from 3 PCs in slice preparation. The superimposed traces represent CSs that were elicited by stimulating the white matter below the recorded PC. Note the variable waveform and the changes in the resting potential just before the onset of the CSs. (B) Examples of spontaneously occurring CSs recorded from 3 PC in anesthetized rats. Note the limited variability of the initial part of the waveform as opposed to the extensive variations in the late wavelets (figure modified from Tal et al., 2008
).
The variability in CS waveform is demonstrated in Figure 4
for intracellular recordings in slice preparation (Figure 4
A) and extracellular recordings in anesthetized rats (Figure 4
B). Significant variation in the waveform duration was observed in both modes of recordings. The variability in duration is due to variability in both the number of wavelets and their duration. In a recent study (Tal et al., 2008
), we explored whether bi-stability could account for the variability in CS waveform. We found that indeed there is a significant difference between the CSs generated during the up state and those generated during the down state. The difference between these groups lies in the amplitude of the initial overshooting action potential and the amplitude and dynamics of the subsequent train of wavelets (Tal et al., 2008
). Following this study we concluded that indeed the CS waveform is state dependent but the characteristics of this dependence are cell specific.
The rather long durations of the up state, suggest that the dynamics of calcium current and intracellular calcium concentration ([Ca++]), may be involved in the mechanism underlying the formation of the state as well as the state transitions. [Ca++] is a multifunctional parameter that participates in a variety of processes including synaptic release (Simon and Llinas, 1985
; Mulkey and Zucker, 1993
), direct activation of K currents (Meech and Standen, 1974
; Yarom et al., 1985
), and long term modulation of synaptic efficacy (Christie et al., 1996
; Ito, 2001
; Sjostrom and Nelson, 2002
). Therefore we have recently examined the dynamics of [Ca++] in the two states and the effect of these states on synaptically evoked Ca++ influx (Rokni and Yarom, 2009
). Using Ca++ imaging to record the changes in [Ca++], we found that epochs of up states are associated with a somatic increase in [Ca++], resembling the increase in [Ca++] induced by intracellularly evoked bursts of Na+-spikes (Lev-Ram et al., 1992
). We further demonstrated that the Ca++ signal associated with CSs is state dependent. At the somatic level the Ca++ signals during the up state were smaller than those occurring during the down state. In contrast, the Ca++ signals at the dendritic level were larger in the up state than in the down state.
Here, we examined the trial by trial variability of the Ca++ waveform. Generally speaking, Ca++ signals covaried in different areas of the dendritic tree. However there were some exceptions. One of these is shown in Figure 5
. This PC had two dendrites emerging from the cell body (Figure 5
A). Fluorescence was averaged in each dendritic region of interest (ROI) (Figure 5
A) to generate the transients shown in Figures 5
B,C. Comparing the amplitudes of these transients in the two dendrites (Figure 5
D) revealed that: (a) There are significant trial by trial changes in the amount of Ca++ influx, and (b) The variations occurred independently in the two branches of the dendritic tree; and (c) Although most of the variability in the right dendrite could be attributed to noise, variability in the left dendrite clearly exceeded the noise level. This spatial specificity is most likely determined by interneuron activity (Callaway et al., 1995
). We concluded that the variability in the Ca++ signal can only partially account for the variability of the CS waveform and that the mechanisms underlying the variations in CS waveforms differ from the mechanisms that induce variation in the dendritic Ca++ signals (see Callaway et al., 1995
; Davie et al., 2008
).
Figure 5. Independence of Ca++ transients in two dendrites. (A) An image of a PC with two dendrites emerging directly from the soma. (B,C) 27 superimposed Ca++ transients recorded at 2.5 ms/frame at the right (B) and left (C) dendrites (ROIs marked by the red ovals in (A); see Rokni and Yarom, 2009
for methods). (D) A scatter plot of the relationship between the amplitudes of the Ca++ transients at the right and left dendrites.
The Controversy over Purkinje Cell Bi-Stability
Although it has become widely accepted that PCs fire intrinsically and do not reflect granule cell inputs in a simple manner, the exact nature of PC firing is a matter of controversy. This controversy cannot be attributed only to differences in preparations, conditions or anesthesia, as even in slices the descriptions of PC firing range from tonic firing (Williams et al., 2002
; Schonewille et al., 2006
), through bi-stable (Loewenstein et al., 2005
; Tal et al., 2008
), to tri-stablel (Womack and Khodakhah, 2002
). These differences are partially due to terminology or the investigators point of view. For example, bi-modal firing of PCs has been described already by Bell and Grimm (1969)
in the late 1960s, and later again by Llinas and Sugimori (1980) and yet these authors have not used the term bi-stability. In addition to these technicalities that may generate an apparent controversy, real differences in physiological observations have been reported. These differences can be attributed to a different state of the system. For example different levels of neuromodulators may either induce a bi-stable state or alternatively abolish it. Indeed Williams et al. (2002)
show that serotonin transforms tonic firing PCs in vitro to bi-stable cells. In our hands PCs can be transformed from bi-stable to tonic firing and back by application of small dc currents. Further investigations are needed in order to unravel how the modulation of intrinsic firing of PCs serves behavior.
Functional Implications of PC Bi-Stability
The involvement of the cerebellum in temporal coordination of motor tasks as well as in a variety of behavioral paradigms is well documented (Ivry et al., 1988
; Timmann et al., 1999
, 2001
; Zackowski et al., 2002
; Ackermann, 2008
; O’Reilly et al., 2008
). These paradigms typically require representation of temporal information in timescales of tens to hundreds of milliseconds. The capacity of PCs to attain prolonged firing states endows the system with intrinsically generated prolonged timescales without the need for a continuous synaptic input. We showed that the firing during these states has a certain degree of irregularity. This irregularity has recently been suggested to encode meaningful information (Steuber et al., 2007
; De Schutter and Steuber, 2009
). However, the ability of the PC-CN synapse to reliably transmit this information is an open question. A partial answer to this question can be found in numerous reports on the properties of the PC-CN synapse (Aizenman et al., 2000
; Kreiner and Jaeger, 2004
; Telgkamp et al., 2004
). This synapse is characterized by the rapid depression of its efficacy upon high frequency stimulation. Thus it is expected that during PC up states the amplitude of the PC-CN synaptic potential will decrease to about 50% of its original value (Telgkamp and Raman, 2002
; Pedroarena and Schwarz, 2003
). Furthermore, because of the massive convergence of hundreds of PC axons onto each CN neuron, the ability of this synapse to transmit the information encoded in the inter spike intervals (ISIs) of PC simple spikes firing, is severely limited. Since there is no indication for tight correlation in simple spike timing in neighboring PCs, we conclude that if the irregularity encodes information it cannot be read by CN neurons. The massive convergence of PC axons in the CN also raises doubts about the ability of CN neurons to resolve changes in PC firing within the up states. This issue has been discussed by Jacobson et al. (2008)
.
We have recently suggested a conceptual framework in which the olivo-cerebellar system serves as a generator of temporal patterns. These patterns, which are needed to perform specific behavioral tasks, are the product of the oscillatory activity in the inferior olive and the bi-stability of PC firing (Jacobson et al., 2008
). Accordingly, the onset and duration of the oscillatory activity in the inferior olive is administrated by the firing states of the PCs. The spatial arrangement of the firing states, which is organized by mossy fiber inputs, governs olivary activity via the GABAergic projection neurons of the CN. According to this framework the information delivered by PCs is the onset and duration of the firing states rather than the irregularities of the inter-spike intervals.
State Transitions
The induction of state transitions by a variety of input signals indicates that indeed PC states are under strict neuronal control and therefore likely to contain valuable information. The most interesting mode of control is that of the mossy fibers. Here the direct excitatory input, via the granule cells, can shift the PCs to their up state, while the indirect inhibitory input via the MLI can shift the cells to their down state or alternatively prevent the shift to an up state. The spatial organization of these two inputs to the cerebellar cortex as well as their temporal relationships will undoubtedly generate a specific spatial organization of the PC states. This spatial organization will be determined by the efficacy of the parallel fiber input. The well documented long term plasticity of the parallel fibers input into PCs offers an additional possibility to this scheme. It implies that the specific spatial organization is a dynamic feature that is molded by experience. Plasticity at the mossy fiber granular cell synapse (Mapelli and D’Angelo, 2007
) can contribute to the dynamic control of the spatial organization of PC states. These possibilities are in line with the commonly accepted notion that the cerebellum is the site of learning and storage of motor skills. The spatial and temporal distributions of the firing states of PCs that are formed by the CF input, have completely different properties. The isochronic organization along the parasagittal plane is a rather rigid organization. The effectiveness of this input in upswing shifts renders this input a somewhat different role. A tempting possibility is that the shifts induced by the CF input serve in emergency situations where rigid, fast and instinctive measures are to be implemented.
It is interesting to note that all types of synaptic inputs can operate as toggle switches, inducing bi-directional transitions. It follows that the response of a PC to synaptic input (the direction of state transition) depends on the current state. For example the response of a quiescent PC to a CF input would be a transition to the up state and prolonged firing. Conversely, in the up state the same PC may respond to the CF input by seizing fire. Hence to reach a particular spatial organization of firing states the input has to be designed according to the current situation. In other words the ‘designer’ of the input should know the current state of the cells. Such knowledge is most unlikely. An alternative possibility is that the efficiency of an input to shift the state of the neuron depends on its state. The slow processes that are responsible for the spontaneous transitions suggest that the threshold for transitions decreases with time and thus the state itself may determine the threshold. This is supported by the observation that the ability to shift state, depends on the time of the input relative to the onset of the state. Thus, a depolarizing input from down state that shifts to an up state is more efficient the longer the cell has been in the down state. The ease in which each input shifts PCs between states needs a thorough examination and characterization.
The Stability of the Input Signal
As stated above, in order to reach a particular spatial organization of firing states, the response to a specific input has to be state dependent. In previous work we demonstrated that the response to a CF input is state dependent. During a depolarizing state complex spikes tend to be prolonged (Tal et al., 2008
) and are associated with differential changes in the calcium influx (Rokni and Yarom, 2009
). A dendritic increase in calcium influx was observed during the up state whereas a decrease was observed at the soma. Here we further investigated the calcium influx associated with the response to CF inputs. First we demonstrated that there is a trial by trial variability in the amount of calcium influx that seems to be independent of the variability in the voltage responses. Second, we presented a specific example of the independent variability of calcium influx that occurred in two dendrites of the same PC. Together these observations suggest that the calcium influx in response to CF inputs is modulated by both, the inhibitory network of the molecular layer interneurons and the PC’s membrane potential. These two modes of modulation of the calcium influx, can furnish the system with the ability to organize the spatial distribution of the firing state independently of the current states.
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Schonewille, M., Khosrovani, S., Winkelman, B. H., Hoebeek, F. E., De Jeu, M. T., Larsen, I. M., Van der Burg, J., Schmolesky, M. T., Frens, M. A., and De Zeeuw, C. I. (2006). Purkinje cells in awake behaving animals operate at the upstate membrane potential. Nat. Neurosci. 9:459–461; author reply 461.