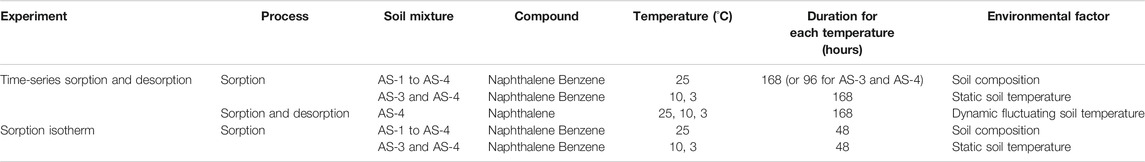
- 1Ecohydrology Research Group, Department of Earth and Environmental Sciences and Water Institute, University of Waterloo, Waterloo, ON, Canada
- 2Environmental, Radiological and Chemical Sciences Division, Canadian Nuclear Laboratories, Chalk River, ON, Canada
- 3KWR Water Research Institute, Nieuwegein, The Netherlands
- 4School of Science and the Environment, Grenfell Campus, Memorial University of Newfoundland, Corner Brook, NL, Canada
- 5Sarnia Technology Applications & Research, Imperial, Sarnia, ON, Canada
- 6Department of Civil and Architectural Engineering, College of Engineering, Qatar University, Doha, Qatar
Petroleum hydrocarbon (PHC) contamination is a global environmental issue. Understanding the key factors and mechanisms controlling the fate and mobility of PHCs in soils and aquifers is critical for environmental risk assessment, the development of remediation strategies, and policy decisions. This study focuses on the effects of soil composition and temperature on the sorption and desorption of two representative aromatic PHC compounds: naphthalene and benzene. The experiments were carried out using artificial sandy loam soil mixtures with temperatures ranging from 3 to 25°C. As expected, the sorption capacities of the soils were primarily controlled by the organic carbon content, while barely affected by the clay content. The sorption data for benzene and naphthalene followed linear to near-linear isotherms. Naphthalene sorption further increased with decreasing temperature, whereas temperature had little effect on benzene sorption. The latter was consistent with the very small magnitude of the sorption enthalpy of benzene. Under imposed dynamic temperature fluctuations, naphthalene sorption and desorption were shown to be reversible: model simulations demonstrated minimal kinetic limitation of the temperature-dependent soil-water partitioning. Our results imply that even in simple artificial soil systems, temperature variations can have complex, but predictable, effects on the soil-pore water partitioning of PHCs and, hence, on their mobility and bioavailability. Understanding the role of temperature is thus a prerequisite to unraveling the coupled abiotic and biotic processes that modulate the fate of PHCs in real-world soils.
Introduction
Although hydrocarbon extraction and storage are increasing all over the world, large and medium oil-spilling events have fortunately been decreasing since the 1970s (ITOPF, 2019). Nonetheless, spills continue to occur during extraction, transport, storage and refining of petroleum. These spills can have severe impacts on terrestrial, freshwater and marine ecosystems, because of the high toxicity of many petroleum hydrocarbons (PHCs) (Onwurah et al., 2007; Varjani, 2017).
In subsurface environments, the fate of PHCs and the associated contamination risks depend on the actual compounds, as well as their migration and degradation. For example, spilled PHCs can penetrate the soil profile due to gravity, sorb on and desorb from minerals and soil organic matter (OM), migrate with groundwater as a dissolved or free phase, be emitted to the atmosphere through volatilization, and transformed into other compounds through abiotic and biotic reactions. Thus, the fate of PHC contaminants is controlled by various environmental factors, including soil geochemistry and hydrology, climatic conditions, and the resident microbial community.
Soil composition exerts a key geochemical control on the sorption and desorption of PHC contaminants (Fine et al., 1997; Falciglia et al., 2011). For instance, OM is a dominant sorbent in soils and, therefore, sorbed PHC concentrations are routinely normalized to soil organic carbon (OC) contents and the sorption strength of the compounds expressed as their OC-water partition coefficients (KOC) (Means et al., 1980; Grathwohl, 1990; Conte et al., 2001). While it is widely accepted that OM dominates sorption of aromatic compounds in soils, expandable clays (e.g., smectite) that have adequate interlayer space, high surface areas, and a relatively high abundance may be equally important sorbents for PHC contaminants (Sheng et al., 2001).
PHC contaminants can be degraded into smaller molecules by naturally occurring abiotic (e.g., photo-oxidation) and biotic reactions. These natural degradation processes are a more effective and preferable method for long-term PHC remediation than removal by mechanical and chemical interventions that are invasive to the soil ecosystem and tend to be expensive (Das and Chandran, 2011; Varjani, 2017). Biodegradation of PHCs may be limited by the supplies of electron acceptors (e.g., oxygen, sulfate, nitrate, oxidized organic compounds, etc.) and nutrients, which can be temporally and spatially constrained by the geochemical and hydro(geo)logical conditions at contaminated sites (Das and Chandran, 2011; Geng et al., 2015; Garg et al., 2017; Ngueleu et al., 2019). In natural soil environments, sorption–desorption processes may also be rate-controlling steps in PHC biodegradation (Al-Bashir et al., 1990; Mihelcic and Luthy, 1991; Guerin and Boyd, 1992). Therefore, a comprehensive understanding of the environmental variables controlling the sorption–desorption dynamics of PHCs is critical to predict their mobility and bioavailability in the subsurface.
Temperature is one of the master variables affecting the fate of PHCs in soils. To variable extents, all soil abiotic and biotic processes are dependent on temperature (Piatt et al., 1996; ten Hulscher and Cornelissen, 1996; Tremblay et al., 2005; Wang et al., 2011; Ngueleu et al., 2018). For instance, Ngueleu et al. (2018) measured higher sorption capacities of benzene and naphthalene to (semi-)arid coastal soils at 5°C than 35°C. However, how temperature affects the reversibility of PHC sorption and desorption is still debated (Wang et al., 2007). Some studies have observed hysteresis, that is, where sorption and desorption isotherms do not coincide. This hysteresis has been attributed to experimental error, changes in the chemical interactions during sorption and desorption due to physical processes such as the mechanical trapping of the sorbed PHC, and inelastic modification of the sorbent following sorption of the sorbate molecule(s) (Wang et al., 2007; Pignatello, 2012).
The present study builds on the hypothesis that the large seasonal variations in soil temperature in cold to cold-temperate regions, such as found in Canada, play a major role in the sorption and desorption dynamics of PHC contaminants and, thus, in their mobility and bioavailability. We therefore carried out sorption–desorption batch experiments at variable temperatures and with different soil compositions. Naphthalene and benzene were selected as representative PHC components. Soil composition was varied systematically by using artificial soil mixtures that cover the typical ranges of clay and OM contents of natural sandy loam soils. Sorption rates and isotherms were measured at fixed (i.e., static) temperatures. Because benzene sorption exhibited no discernable temperature dependence, naphthalene was further employed in experiments with a dynamic temperature regime to determine the reversibility of its sorption–desorption behavior. The dynamic temperature experiments directly address the hypothesis that soil temperature fluctuations may contribute to regulating PHC uptake from and release to soil pore waters.
Materials and Methods
Artificial Soil Mixtures
Four artificial soil mixtures, denoted AS-1, AS-2, AS-3, and AS-4 (Table 1), with different sandy loam textures were prepared by mixing quartz sand (OK-75, U.S. Silica), silt-sized quartz (Sil-Co-Sil® 53, U.S. Silica), montmorillonite (Ceratosil® WG, Süd-Chemie AG, Germany) and OM. For the quartz sand and silt-sized quartz, the ranges of particle size were 0.053 to 0.6 mm and less than 0.045 to 0.106 mm, respectively, based on the supplier’s product information. The montmorillonite comprised 8% sand-sized (>0.063 mm), 25% silt-sized (0.002–0.063 mm), and 67% clay-sized (<0.002 mm) material (Pronk et al., 2012). Fresh maple leaves were air-dried, ground, sieved (0.063–1 mm), and washed seven times with Milli-Q water, air-dried again and added as the source of soil OM. Because of their small particle sizes and abundant functional groups, the montmorillonite and OM were assumed to be the two soil components controlling PHC sorption/desorption. The montmorillonite and OM contents used were 5 and 15 weight % (wt%), and 1 and 5 wt%, respectively (Table 1). The artificial soil mixtures were analyzed for their organic and inorganic carbon contents on a CHNS Carlo Erba analyzer. The two AS-4 compositions listed in Table 1 are for two separately prepared soil mixtures used in the static and dynamic temperature experiments, respectively.
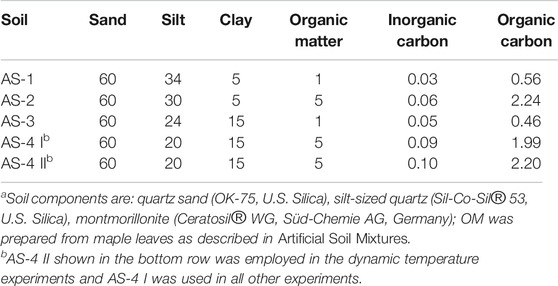
TABLE 1. Mineral and organic contents in weight % of the four artificial soils (AS).a
Petroleum Hydrocarbon Stock and Experimental Solutions
Benzene (C6H6) and naphthalene (C10H8) were selected as representative PHC contaminants for the sorption and desorption experiments. Separate stock solutions of benzene and naphthalene (20 mg L−1) were prepared by adding either benzene (≥99.9%, Sigma-Aldrich®) or naphthalene (≥99%, Sigma-Aldrich®) to Milli-Q water, which contained CaCl2 (905.9 mg L−1) as background electrolyte and HgCl2 (271.5 mg L−1) as biocide to prevent PHC biodegradation. Each stock solution was sealed in an amber bottle with a Teflon-faced septum and minimal headspace. To ensure complete dissolution, the benzene and naphthalene stock solutions were stirred with Teflon-coated magnetic bars for four to seven days. To prepare the experimental solutions, aliquots of the stock solutions were diluted with matrix solutions containing CaCl2 (905.9 mg L−1) and HgCl2 (271.5 mg L−1) and stirred for ∼15 min prior to starting the experiments. The concentrations of benzene and naphthalene in the stock and experimental solutions were determined by gas chromatography (GC, see Analytical Methods) before applied to the sorption and desorption experiments.
Sorption and Desorption Experiments
Time-Series Sorption Experiments
The extent of sorption and the time scale required to reach sorption equilibrium were determined in batch experiments with the four soil mixtures (AS-1 to 4) at different temperatures (Table 2). In an experiment, 15 g of a given AS was added in a 60 ml serum bottle to 53 ml diluted benzene or naphthalene solution (initial concentration C0 = 2 mg L−1). Averaged over all the experiments, the liquid-to-solid ratio was 3.51 ± 0.07 mL g−1. The serum bottles were sealed with Teflon-faced septa and open-top unlined aluminum seals immediately following addition of the solution and AS mixture to the bottles. The headspace in the serum bottles was minimized to limit PHC volatilization. Control bottles were filled with 60 ml diluted benzene or naphthalene solution only. The experimental and control bottles were agitated on a rotary wheel at 10 revolutions per minute (rpm) in an environmental chamber (Percival Scientific CTH-118) with the temperature maintained at 25°C. Based on the results of the time-series sorption experiments at 25°C (see Time-Series Sorption Experiments), only AS-3 and AS-4 were used in the experiments at 10 and 3°C to assess the effect of temperature (Table 2). Each temperature was imposed for seven days, except the 25°C experiments with AS-3 and AS-4 in which temperature was maintained for four days, to allow sorption to reach equilibrium. At selected times, a set of triplicate experimental bottles plus corresponding duplicate control bottles were sacrificially sampled for GC analysis (see Analytical Methods).
Dynamic Temperature Experiments
Based on the results of the separate sorption experiments conducted at the three temperatures (i.e., 25, 10, or 3°C, see Time-Series Sorption Experiments), the effects of a dynamic temperature regime were investigated to test the hypothesis that this would cause reversible PHC sorption and desorption. Based on the results of the separate sorption experiments (see Time-Series Sorption Experiments and Dynamic Temperature Experiments), the sorption and desorption experiments under dynamic temperature were only conducted with AS-4 and naphthalene (C0 = 5 mg L−1, liquid-to-solid ratio = 3.54 ± 0.07 mL g−1). Temperature was first decreased in a stepwise fashion from 25 via 10 to 3°C, and then raised again from 3 through 10 to 25°C (Table 2). Each temperature was maintained for seven days. At selected times, a set of triplicate experimental bottles plus duplicate control bottles were sacrificially sampled for GC analysis (see Analytical Methods).
Sorption Isotherm Experiments
Aqueous-solid distributions of benzene and naphthalene were determined at the three different temperatures. Triplicate benzene or naphthalene solutions with initial concentrations of 1, 2, 5, 8, 11, and 13 mg L−1 (see Supplementary Table S1 for GC determined concentrations of initial solutions) were mixed separately with the four AS at 25°C. Experiments at 10 and 3°C were conducted with AS-3 and AS-4 only (Table 2). The concentrations of benzene or naphthalene were identical in the experimental and control bottles, but the latter did not contain any AS. The isotherm experiments were run for 48 h, which was sufficient to reach sorption equilibrium according to the results of the time-series sorption experiments (see Time-Series Sorption Experiments and Dynamic Temperature Experiments).
Analytical Methods
At selected times during the time-series sorption and dynamic temperature experiments, or after equilibrium was reached in the sorption isotherm experiments, triplicate experimental bottles and corresponding duplicate control bottles were sacrificially sampled. Each bottle was centrifuged (1500 rpm, 10 min) at the same temperature at which they were being incubated during the experiments. Following centrifugation, 19 ml of supernatant was collected from each bottle with a glass syringe and a stainless-steel needle. A micro-extraction method was used to obtain the benzene or naphthalene concentration in the supernatant.
For the micro-extractions, 19 ml of solution (i.e., the stock solutions, initial experimental solutions, or supernatant samples obtained from the experiments) was mixed in 20 ml glass vials with 1 ml of dichloromethane extractant containing meta-fluorotoluene as an internal standard. The glass vials were sealed with Teflon-faced septa and open-top caps. They were shaken at 350 rpm for 20 min and then left to rest bottoms-up for ∼30 min until the organic phase fully settled. The organic phase was extracted using a gas-tight glass syringe and transferred to a screw-thread GC vial (VWR®). The vials were loaded onto a GC sampler and analyzed for the benzene or naphthalene concentration on an Agilent 7890A GC instrument. Organic phase samples were injected at a port temperature of 275°C and passed through a DB5 capillary column. The concentrations of benzene and naphthalene were measured by a flame ionization detector (detection limit = 5 μg L−1).
The fraction (
where
Mathematical Modeling
The measured benzene and naphthalene concentrations in the sorption isotherm experiments were best fitted with linear and Freundlich isotherms:
where
A two-site kinetic sorption model was applied to fit the dynamic temperature sorption data. The model assumes that sorption to a first pool of sites instantaneously equilibrates with the aqueous phase, while a second pool of sites accounts for the slow (kinetic) binding of the sorbate to the sorbent. The slow binding is assumed to follow first-order kinetics with respect to the sorbate occupancy of the kinetic sites (Šimůnek and van Genuchten, 2008; Maraqa and Khashan, 2014). Based on the linear isotherms observed during the sorption of naphthalene to AS-4 at 25, 10, and 3°C, the two-site kinetic sorption model was implemented using Eqs 5–8:
where
When fitting the dynamic temperature sorption data to the two-site kinetic model, the average mass of AS-4 (m = 15 g) and the average volume of naphthalene solution (V = 53 ml) in the experiments were imposed. Sorption isotherm and rate model parameters were obtained using the Melder-Mead simplex algorithm and fminsearchbnd function in MATLAB (Lagarias et al., 1998; Purchase, 2007; D’Errico, 2012). The fitting minimized the value of the root mean square error (RMSE) which was used as a quantitative measure of the goodness of fit.
The enthalpies of sorption,
where
Results
Time-Series Sorption Experiments
Sorption of Benzene and Naphthalene at 25°C
The time-series concentrations of benzene (Figure 1A) and naphthalene (Figure 1B) remaining in the aqueous phase as well as their sorbed concentrations (Figures 1C,D) are normalized to the initial dissolved concentration (
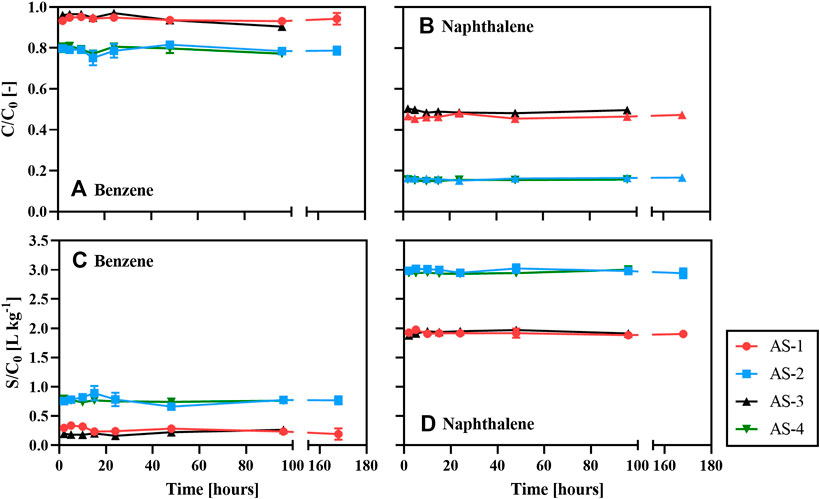
FIGURE 1. Normalized concentrations of aqueous benzene (A), aqueous naphthalene (B), sorbed benzene (C), and sorbed naphthalene (D) in the time-series sorption experiments with the four soil mixtures at 25°C.
Effects of Variable Organic Matter and Clay Contents
The relatively high sorption capacities of AS-2 and AS-4 can be attributed to their higher OM contents (5%), compared to those of AS-1 and AS-3 (1%, Table 1). This is consistent with the frequently reported dominant effect of soil OM on the sorption of benzene and naphthalene, especially at soil OC contents exceeding 0.1% (Karickhoff et al., 1979; Schwarzenbach and Westall, 1981; Redding et al., 2002). It is therefore common practice to normalize the fraction of sorbed hydrocarbon to the soil OC content (
where the values of
For benzene, the values of
Sorption of benzene and naphthalene to the artificial soil mixtures with the same OM content but different clay contents (i.e., AS-1 and AS-3, or AS-2 and AS-4) was undistinguishable (Figure 1). Hence, the montmorillonite content had no impact on the benzene and naphthalene sorption capacities. In contrast with our results, several previous studies (Sheng et al., 2001; Zhang et al., 2010; Yang et al., 2013; Martins et al., 2018) showed that clay minerals can be an important sorbent for organic contaminants. In our experiments, Ca2+ occupancy of the montmorillonite surface sites could have inhibited the sorption of benzene and naphthalene, possibly by shrinking the available sorption domains and/or changing the optimal interlayer spacing (Rogers et al., 1980; Sheng et al., 2001; Zhang et al., 2010). Because Ca2+ is a typical ion in natural soil solutions, we added it as the background electrolyte cation in our experiments (Pronk et al., 2012). This may have inadvertently suppressed the role of the clay mineral fraction.
Sorption of Benzene and Naphthalene at Different Temperatures
Because the OM content was the dominant factor controlling the sorption capacities of the AS mixtures, only AS-3 (1% OM) and AS-4 (5% OM) were selected to represent soils with low and high OC contents in the experiments where the temperature was the variable environmental factor. As described in Sorption and Desorption Experiments, sorption incubations were conducted at temperatures of 25, 10, and 3°C.
As with the prior sorption experiments that were performed at 25°C, most of the sorption occurred within the first 2 h at 10 and 3°C. Assuming that sorption equilibrium was assuredly reached after 48 h, the normalized concentrations of sorbed benzene and naphthalene to AS-3 were lower than those observed for AS-4 at all temperatures (Supplementary Figure S1), indicating that the OM content governed sorption capacities under the imposed experimental conditions. Benzene sorption was slightly less at 25°C than 3°C, in particular for AS-3. However, benzene sorption at 10°C was highest for AS-3 and lowest for AS-4. Thus, no clear temperature trend emerged for benzene sorption (Supplementary Figure S1A). By contrast, a systematic temperature dependence was observed for naphthalene: for sorption to both AS-3 and AS-4, the lowest, intermediate, and highest normalized sorbed concentrations were observed at 25, 10, and 3°C, respectively (Supplementary Figure S1B).
Dynamic Temperature Experiments
The sorption experiments conducted under static temperature revealed that the extent of sorption of naphthalene was not only much higher than for benzene, but also exhibited a consistent dependence on temperature. We therefore chose to use naphthalene and the high-OM soil AS-4 for the experiment where we imposed a dynamic temperature regime (Table 2).
Following the mixing of the dissolved naphthalene solution (
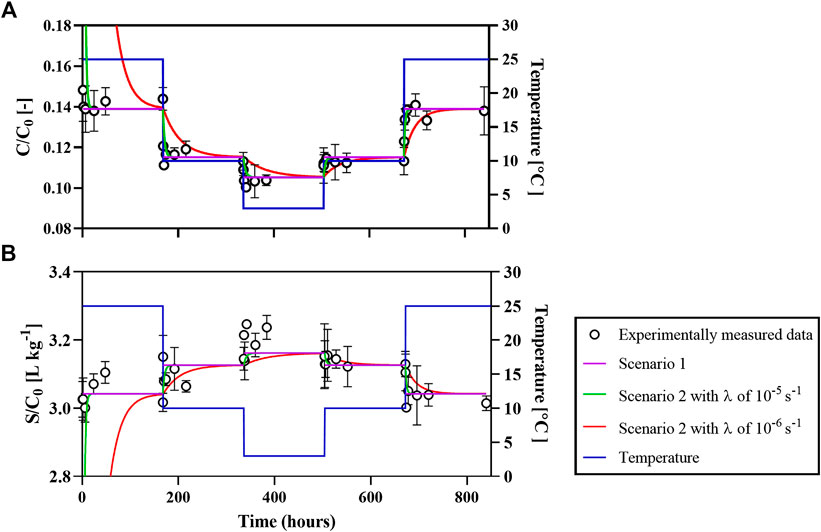
FIGURE 2. Normalized concentrations of aqueous naphthalene (A) and sorbed naphthalene (B) during incubation with AS-4 under dynamic temperature fluctuations (right Y axis). Experimentally measured data and model fits are plotted against the left Y axis. Scenario 1 assumes that AS-4 only carries equilibrium sorption sites (i.e.,
When the temperature was increased back from 3 to 10°C, naphthalene sorption decreased to 88.7% after 168 h (or 672 h in cumulative time). The further elevation of temperature from 10 to 25°C resulted in another drop of
At corresponding temperatures, somewhat higher fractions of sorbed naphthalene were observed in the dynamic temperature experiment compared to those in the time-series sorption experiments. The latter yielded values of 84.2%, 85.7%, and 88.5% at 25, 10, and 3°C, respectively. These differences are attributed to the slightly higher OC in the AS-4 batch used in the dynamic temperature experiment compared to all the other experiments with AS-4 (see Table 1).
Sorption Isotherms
The time-series sorption experiment showed that the sorption of benzene and naphthalene quickly reached equilibrium. Therefore, we derived the sorption isotherms from the data of the incubations of the different AS with benzene or naphthalene solutions measured after 48 h. For the range of hydrocarbon concentrations (
For benzene and naphthalene sorption at 25°C, the distribution coefficients
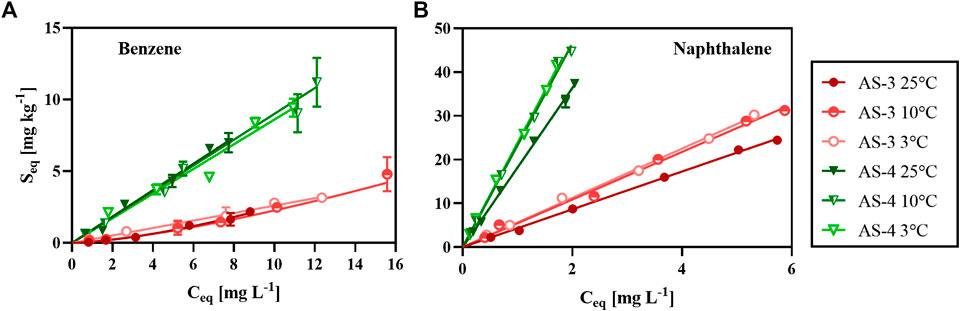
FIGURE 3. Sorption isotherms of benzene (A) and naphthalene (B) for AS-3 and AS-4 at 25, 10, and 3°C. Experimentally measured data for benzene with AS-3 at 25 and 10°C (solid and half-filled circles in A) were fitted to the Freundlich isotherm model (slightly curved lines in A) and all other data (circles and triangles in A and B) were fitted to the linear isotherm model (straight lines).
Discussion
Comparing Organic Carbon-Water Partition Coefficients
The organic carbon-water partition coefficients
Benzene exhibits a lower affinity to the AS than naphthalene: for benzene,
The large differences in partition coefficients between naphthalene and benzene likely reflect differences in sorption mechanisms. The higher
Temperature Sensitivity and Sorption Enthalpy
The partitioning coefficients derived from the sorption isotherm experiments at 3 and 25°C yield
Benzene’s sorption enthalpy with AS-4 (
By extension, for PHC compounds other than naphthalene and benzene, reported sorption enthalpies with organic carbon sorbents could be used as a first indicator to predict how their sorption to soil OC may be affected by temperature. An important control on the magnitude of the sorption enthalpy is likely the compounds’ size expressed by the number of carbon atoms (Campbell and Sellers, 2013). Longer PHCs with more carbon atoms should be expected to have larger negative
Reversible Naphthalene Sorption Under Dynamic Temperature Fluctuations
Visual inspection of Figure 2 shows that the temperature dependent naphthalene sorption and desorption reactions are fully reversible. Thermodynamically controlled reversible sorption of naphthalene to AS-4 is also readily evident from the results of the two-site sorption modeling (Mathematical Modeling) for the following three scenarios. In scenario 1, binding to AS-4 only occurs at equilibrium sorption sites (i.e.,
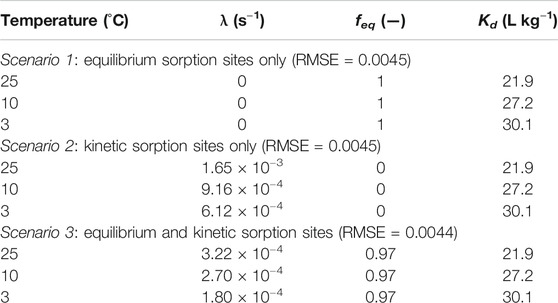
TABLE 3. The best fitting parameters of three scenarios using a two-site kinetic model for naphthalene sorption to AS-4 under the imposed dynamic temperature regime.
In scenario 1, naphthalene sorption to AS-4 reaches equilibrium instantaneously. Therefore, the concentration dynamics of sorbed naphthalene are only a function of the
In scenario 2, the
The modeling results of scenario 3 are in line with the previous simulations. The best fits imply that most (97%) of sorption sites are equilibrium sites (Table 3). Since
In summary, sorption and desorption are reversible under the imposed dynamic temperature changes. The sorption enthalpy derived from the
Implications
The dynamic temperature experiment in Figure 2 demonstrates in a very direct way the very fast and reversible sorption and desorption of naphthalene driven by changes in temperature: sequestration by the OM in AS-4 increases when temperature decreases and vice versa. Furthermore, the temperature sensitivity of the partitioning coefficients of benzene and naphthalene are related to their enthalpies of sorption. The latter are a function of both the molecular structure of the sorbate compound (e.g., the molecular length and aromaticity) and the nature of the binding sites of the soil matrix. In a similar fashion, the
While our study focuses on the temperature effects on abiotic sorption and desorption, other soil reaction processes, including microbially-catalyzed reactions, are temperature-dependent. Microbial activity typically increases with increasing temperature until some optimum temperature is reached (Hobbs et al., 2013). Thus, for example, we would expect that rising soil temperatures in spring would have a dual impact on the bioavailability and degradation of compounds such as naphthalene: 1) desorption of the compound from the soil matrix into the aqueous, bioavailable pool, and 2) higher microbial biodegradation rates of the soluble compound, at least as long as the temperature remains below the temperature optimum. Several approaches exist to predict the effect of temperature on microbial biodegradation rates, such as macromolecular rate theory (MMRT) (Hobbs et al., 2013; Schipper et al., 2014), which accounts for the temperature optimum, or Arrhenius-type relationships (Dale et al., 2006), which do not estimate a temperature optimum. Note, however, that desorption of a PHC does not just increase its bioavailability but may also induce toxic effects to soil biota.
Natural soils are spatially heterogenous with pore spaces and small cavities of various sizes and geometries, which may affect the macroscopic sorption/desorption dynamics of PHCs. These effects are not addressed with the well-mixed artificial soils used in this study. In addition, we used single compounds (benzene and naphthalene) in the experiments to advance our fundamental understanding of the sorption and desorption reactions. However, PHC contamination in the field normally involves mixtures of compounds that may partition over soil, water and gas phases. Future work may therefore focus on testing the extent to which the knowledge of simple systems, such as the ones used here, can be extrapolated to more complex, real-world soil-PHC systems. Moreover, further insights on the mechanisms of PHC interactions with natural soils may require the use of advanced molecular analysis tools.
Conclusions
Under the imposed experimental conditions in this study, the extent of sorption of benzene was significantly lower than that of naphthalene, regardless of temperature and the soil mixture used. We attribute this to the higher
Data Availability Statement
The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.
Conflict of Interest
Author KS was employed by the company Imperial Oil. The remaining authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Author contributions
BS and SN contributed equally to this study in laboratory experiments, data processing and writing the manuscript. SN, FR, PVC, CS, and GP designed the experiments. SN was responsible for the two-site model simulations. PVC and FR supervised the work and helped BS and SN interpret the data. All authors contributed to the preparation of the manuscript and have approved its final version.
Funding
Funding was provided by the Canada Excellence Research Chair (CERC) program, two Natural Sciences and Engineering Research Council Partnership Grants with Imperial Oil Resources Ltd. (Engage Grant to Rezanezhad: EGP-516205-17, Collaborative Research and Development Grant to Van Cappellen: CRDPJ: 533227-18), and Qatar National Research Fund (NPRP9-93-1-021).
Acknowledgments
The authors thank Marianne Vandergriendt, Shirley Chatten, Sierra Cranmer-Smith, and Lindsay Norwood for their assistance with laboratory experiments and analyses.
Supplementary Material
The Supplementary Material for this article can be found online at: https://www.frontiersin.org/articles/10.3389/fenvc.2020.581103/full#supplementary-material
References
Al-Bashir, B., Cseh, T., Leduc, R., and Samson, R. (1990). Effect of soil/contaminant interactions on the biodegradation of naphthalene in flooded soil under denitrifying conditions. Appl. Microbiol. Biotechnol. 34, 414–419. doi:10.1007/BF00170071
Campbell, C. T., and Sellers, J. R. V. (2013). Enthalpies and entropies of adsorption on well-defined oxide surfaces: Experimental measurements. Chem. Rev. 113, 4106–4135. doi:10.1021/cr300329s
Conte, P., Zena, A., Pilidis, G., and Piccolo, A. (2001). Increased retention of polycyclic aromatic hydrocarbons in soils induced by soil treatment with humic substances. Environ. Pollut. 112, 27–31. doi:10.1016/S0269-7491(00)00101-9
D’Errico, J. (2012). Fminsearchbnd, fminsearchcon (bound constrained optimization using fminsearch). MATLAB Central File Exchange. Available at: http://www.mathworks.com/matlabcentral/fileexchange/8277-fminsearchbnd-fminsearchcon. (Accessed March 30, 2020).
Dale, A. W., Regnier, P., and Van Cappellen, P. (2006). Bioenergetic controls on anaerobic oxidation of methane (AOM) in coastal marine sediments: a theoretical analysis. Am. J. Sci. 306, 246–294. doi:10.2475/ajs.306.4.246
Das, N., and Chandran, P. (2011). Microbial degradation of petroleum hydrocarbon contaminants: an overview. Biotechnol. Res. Int. 2011, 1–13. doi:10.4061/2011/941810
Endo, S., and Goss, K.-U. (2014). Applications of polyparameter linear free energy relationships in environmental chemistry. Environ. Sci. Technol. 48, 12477–12491. doi:10.1021/es503369t
Endo, S., Grathwohl, P., Haderlein, S. B., and Schmidt, T. C. (2009). LFERs for soil organic carbon−water distribution coefficients (Koc) at environmentally relevant sorbate concentrations. Environ. Sci. Technol. 43, 3094–3100. doi:10.1021/es803157e
Falciglia, P. P., Giustra, M. G., and Vagliasindi, F. G. A. (2011). Low-temperature thermal desorption of diesel polluted soil: influence of temperature and soil texture on contaminant removal kinetics. J. Hazard Mater. 185, 392–400. doi:10.1016/j.jhazmat.2010.09.046
Fine, P., Graber, E. R., and Yaron, B. (1997). Soil interactions with petroleum hydrocarbons: abiotic processes. Soil Technol. 10, 133–153. doi:10.1016/S0933-3630(96)00088-8
Garg, S., Newell, C. J., Kulkarni, P. R., King, D. C., Adamson, D. T., Renno, M. I., et al. (2017). Overview of natural source zone depletion: processes, controlling factors, and composition change. Groundwater Monit R 37, 62–81. doi:10.1111/gwmr.12219
Geng, X., Boufadel, M. C., Lee, K., Abrams, S., and Suidan, M. (2015). Biodegradation of subsurface oil in a tidally influenced sand beach: impact of hydraulics and interaction with pore water chemistry. Water Resour. Res. 51, 3193–3218. doi:10.1002/2014WR016870
Goring, C. A. I., and Hamaker, J. W. (1972). Organic chemicals in the soil environment. New York, NY: Marcel Dekker, Inc.
Grathwohl, P. (1990). Influence of organic matter from soils and sediments from various origins on the sorption of some chlorinated aliphatic hydrocarbons: implications on Koc correlations. Environ. Sci. Technol. 24, 1687–1693. doi:10.1021/es00081a010
Guerin, W. F., and Boyd, S. A. (1992). Differential bioavailability of soil-sorbed naphthalene to two bacterial species. Appl. Environ. Microbiol. 58, 1142–1152. doi:10.1128/aem.58.4.1142-1152.1992
Halsey, G. (1948). Physical adsorption on non‐uniform surfaces. J. Chem. Phys. 16, 931–937. doi:10.1063/1.1746689
Hobbs, J. K., Jiao, W., Easter, A. D., Parker, E. J., Schipper, L. A., and Arcus, V. L. (2013). Change in heat capacity for enzyme catalysis determines temperature dependence of enzyme catalyzed rates. ACS Chem. Biol. 8, 2388–2393. doi:10.1021/cb4005029
ITOPF (2019). Oil tanker spill statistics 2018. Available at: http://www.itopf.org/fileadmin/data/Documents/Company_Lit/Oil_Spill_Stats_2018.pdf (Accessed July 25, 2019).
Karickhoff, S., Brown, D., and Scott, T. (1979). Sorption of hydrophobic pollutants on natural sediments. Water Res. 13, 241–248. doi:10.1016/0043-1354(79)90201-X
Lagarias, J. C., Reeds, J. A., Wright, M. H., and Wright, P. E. (1998). Convergence properties of the nelder-mead simplex method in low dimensions. SIAM J. Optim. 9, 112–147. doi:10.1137/S1052623496303470
Liu, S. (2015). Cooperative adsorption on solid surfaces. J. Colloid Interface Sci. 450, 224–238. doi:10.1016/j.jcis.2015.03.013
Maraqa, M. A., and Khashan, S. A. (2014). Modeling solute transport affected by heterogeneous sorption kinetics using single-rate nonequilibrium approaches. J. Contam. Hydrol. 157, 73–86. doi:10.1016/j.jconhyd.2013.11.005
Martins, E. C., de Freitas Melo, V., Bohone, J. B., and Abate, G. (2018). Sorption and desorption of atrazine on soils: the effect of different soil fractions. Geoderma 322, 131–139. doi:10.1016/j.geoderma.2018.02.028
Means, J. C., Wood, S. G., Hassett, J. J., and Banwart, W. L. (1980). Sorption of polynuclear aromatic hydrocarbons by sediments and soils. Environ. Sci. Technol. 14, 1524–1528. doi:10.1021/es60172a005
Mihelcic, J. R., and Luthy, R. G. (1991). Sorption and microbial degradation of naphthalene in soil-water suspensions under denitrification conditions. Environ. Sci. Technol. 25, 169–177. doi:10.1021/es00013a020
Ngueleu, S. K., Rezanezhad, F., Al-Raoush, R. I., and Van Cappellen, P. (2018). Sorption of benzene and naphthalene on (semi)-arid coastal soil as a function of salinity and temperature. J. Contam. Hydrol. 219, 61–71. doi:10.1016/j.jconhyd.2018.11.001
Ngueleu, S. K., Al-Raoush, R. I., Shafieiyoun, S., Rezanezhad, F., and Van Cappellen, P. (2019). Biodegradation kinetics of benzene and naphthalene in the vadose and saturated zones of a (semi)-arid saline coastal soil environment. Geofluids 2019, 1–15. doi:10.1155/2019/8124716
Nguyen, T. H., Goss, K.-U., and Ball, W. P. (2005). Polyparameter linear free energy relationships for estimating the equilibrium partition of organic compounds between water and the natural organic matter in soils and sediments. Environ. Sci. Technol. 39, 913–924. doi:10.1021/es048839s
Onwurah, I. N. E., Ogugua, V. N., Onyike, N. B., Ochonogor, A. E., and Otitoju, O. F. (2007). Crude oil spills in the environment, effects and some innovative clean-up biotechnologies. Int. J. Environ. Res. 1, 307–320. doi:10.22059/IJER.2010.142
Piatt, J. J., Backhus, D. A., Capel, P. D., and Eisenreich, S. J. (1996). Temperature-dependent sorption of naphthalene, phenanthrene, and pyrene to low organic carbon aquifer sediments. Environ. Sci. Technol. 30, 751–760. doi:10.1021/es9406288
Pignatello, J. J. (2012). Dynamic interactions of natural organic matter and organic compounds. J. Soils Sediments 12, 1241–1256. doi:10.1007/s11368-012-0490-4
Pronk, G. J., Heister, K., Ding, G. C., Smalla, K., and Kögel-Knabner, I. (2012). Development of biogeochemical interfaces in an artificial soil incubation experiment; aggregation and formation of organo-mineral associations. Geoderma 189–190, 585–594. doi:10.1016/j.geoderma.2012.05.020
Purchase, K. (2007). Fminsearchbnd new (improved bounded fminsearch algorithm). MATLAB Central File Exchange. Available at: https://www.mathworks.com/matlabcentral/fileexchange/17804-fminsearchbnd-new (Accessed March 30, 2020).
Redding, A. Z., Burns, S. E., Upson, R. T., and Anderson, E. F. (2002). Organoclay sorption of benzene as a function of total organic carbon content. J. Colloid Interface Sci. 250, 261–264. doi:10.1006/jcis.2001.8205
Rogers, R. D., McFarlane, J. C., and Cross, A. J. (1980). Adsorption and desorption of benzene in two soils and montmorillonite clay. Environ. Sci. Technol. 14, 457–460. doi:10.1021/es60164a005
Schipper, L. A., Hobbs, J. K., Rutledge, S., and Arcus, V. L. (2014). Thermodynamic theory explains the temperature optima of soil microbial processes and high Q 10 values at low temperatures. Global Change Biol. 20, 3578–3586. doi:10.1111/gcb.12596
Schwarzenbach, R. P., Gschwend, P. M., and Imboden, D. M. (2002). Environmental organic chemistry. Hoboken, NJ, USA: John Wiley & Sons, Inc. doi:10.1002/0471649643
Sheng, G., Johnston, C. T., Teppen, B. J., and Boyd, S. A. (2001). Potential contributions of smectite clays and organic matter to pesticide retention in soils. J. Agric. Food Chem. 49, 2899–2907. doi:10.1021/jf001485d
Šimůnek, J., and van Genuchten, M. T. (2008). Modeling nonequilibrium flow and transport processes using HYDRUS. Vadose Zone J. 7, 782–797. doi:10.2136/vzj2007.0074
ten Hulscher, T. E. M., and Cornelissen, G. (1996). Effect of temperature on sorption equilibrium and sorption kinetics of organic micropollutants - a review. Chemosphere 32, 609–626. doi:10.1016/0045-6535(95)00345-2
Tremblay, L., Kohl, S. D., Rice, J. A., and Gagné, J.-P. (2005). Effects of temperature, salinity, and dissolved humic substances on the sorption of polycyclic aromatic hydrocarbons to estuarine particles. Mar. Chem. 96, 21–34. doi:10.1016/j.marchem.2004.10.004
Varjani, S. J. (2017). Microbial degradation of petroleum hydrocarbons. Bioresour. Technol. 223, 277–286. doi:10.1016/j.biortech.2016.10.037
Wang, G., Kleineidam, S., and Grathwohl, P. (2007). Sorption/desorption reversibility of phenanthrene in soils and carbonaceous materials. Environ. Sci. Technol. 41, 1186–1193. doi:10.1021/es060805s
Wang, L., Yang, Z., and Niu, J. (2011). Temperature-dependent sorption of polycyclic aromatic hydrocarbons on natural and treated sediments. Chemosphere 82, 895–900. doi:10.1016/j.chemosphere.2010.10.054
Yang, L., Jin, M., Tong, C., and Xie, S. (2013). Study of dynamic sorption and desorption of polycyclic aromatic hydrocarbons in silty-clay soil. J. Hazard Mater. 244–245, 77–85. doi:10.1016/j.jhazmat.2012.11.029
Keywords: petroleum hydrocarbons, artificial soil, dynamic temperature, sorption equilibrium, reversible sorption/desorption, thermodynamics
Citation: Shi B, Ngueleu SK, Rezanezhad F, Slowinski S, Pronk GJ, Smeaton CM, Stevenson K, Al-Raoush RI and Van Cappellen P (2020) Sorption and Desorption of the Model Aromatic Hydrocarbons Naphthalene and Benzene: Effects of Temperature and Soil Composition. Front. Environ. Chem. 1:581103. doi: 10.3389/fenvc.2020.581103
Received: 09 July 2020; Accepted: 02 October 2020;
Published: 06 November 2020.
Edited by:
Andrew R. Zimmerman, University of Florida, United StatesReviewed by:
Alfin Kurniawan, National Sun Yat-sen University, TaiwanSubbaiah Muthu Prabhu, Alagappa University, India
Copyright © 2020 Shi, Ngueleu, Rezanezhad, Slowinski, Pronk, Smeaton, Stevenson, Al-Raoush and Van Cappellen. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Bingjie Shi, YmluZ2ppZS5zaGlAdXdhdGVybG9vLmNhLg==
†These authors have contributed equally to this work.
 Bingjie Shi
Bingjie Shi Stephane K. Ngueleu1,2†
Stephane K. Ngueleu1,2† Fereidoun Rezanezhad
Fereidoun Rezanezhad Stephanie Slowinski
Stephanie Slowinski Geertje Johanna Pronk
Geertje Johanna Pronk Christina M. Smeaton
Christina M. Smeaton Riyadh I. Al-Raoush
Riyadh I. Al-Raoush Philippe Van Cappellen
Philippe Van Cappellen