- Department of Mathematics, Technion – Israel Institute of Technology, Haifa, Israel
We study the generic convergence of infinite products of nonexpansive mappings with unbounded domains in hyperbolic metric spaces.
1. Introduction and the Main Result
Let (X, ρ) be a metric space and let R1 denote the real line. We say that a mapping c : R1 → X is a metric embedding of R1 into X if ρ(c(s), c(t)) = |s − t| for all real s and t. The image of R1 under a metric embedding will be called a metric line. The image of a real interval [a, b] = {t ∈ R1: a ≤ t ≤ b} under such a mapping will be called a metric segment.
Assume that (X, ρ) contains a family M of metric lines such that for each pair of distinct points x and y in X, there is a unique metric line in M which passes through x and y. This metric line determines a unique metric segment joining x and y. We denote this segment by [x, y]. For each 0 ≤ t ≤ 1, there is a unique point z in [x, y] such that
This point is denoted by (1 − t)x ⊕ ty. We say that X, or more precisely, (X, ρ, M), is a hyperbolic metric space if
for all x, y, and z in X. An equivalent requirement is that
for all x, y, z, and w in X. A set K ⊂ X is called ρ-convex if [x, y] ⊂ K for all x and y in K.
It is clear that all normed linear spaces are hyperbolic in this sense. A discussion of more examples of hyperbolic spaces and, in particular, of the Hilbert ball can be found, for example, in Goebel and Reich [1] and Reich and Shafrir [2].
Let (X, ρ, M) be a complete hyperbolic metric space, and let K ⊂ X be a nonempty, closed and ρ-convex subset of (X, ρ). For each C: K → K, set C0(x) = x for all x ∈ K. Denote by 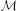 the set of all sequences {At}∞t = 1 of mappings At : K → K, t = 1, 2, …, such that for all integers t ≥ 1,
the set of all sequences {At}∞t = 1 of mappings At : K → K, t = 1, 2, …, such that for all integers t ≥ 1,
For each x ∈ X and each r > 0, set
B(x, r) = {y ∈ X: ρ(x, y) ≤ r} and BK(x, r) = B(x, r) ∩ K.
Fix θ ∈ K. For each M, ϵ > 0, set
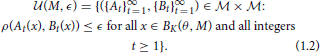
We equip the set 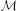 with the uniformity which has the base
with the uniformity which has the base
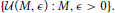
It is not difficult to see that the uniform space 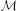 is metrizable (by a metric d) and complete.
is metrizable (by a metric d) and complete.
Denote by 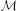 * the set of all {At}∞t = 1 ∈
* the set of all {At}∞t = 1 ∈ 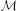 for which there exists a point ∈ K satisfying
for which there exists a point ∈ K satisfying
Denote by 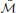 * the closure of the set
* the closure of the set 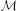 * in the uniform space
* in the uniform space 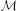 . We consider the topological subspace
. We consider the topological subspace 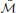 * ⊂
* ⊂ 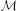 equipped with the relative topology and the metric d.
equipped with the relative topology and the metric d.
In this paper we study the asymptotic behavior of (unrestricted) infinite products of generic sequences of mappings belonging to the space 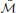 * and obtain convergence to a unique common fixed point. More precisely, we establish the following result, which generalizes the corresponding result in Reich and Zaslavski [3] (see also [4] and [5]). That result was obtained in the case where the set K was bounded.
* and obtain convergence to a unique common fixed point. More precisely, we establish the following result, which generalizes the corresponding result in Reich and Zaslavski [3] (see also [4] and [5]). That result was obtained in the case where the set K was bounded.
Theorem 1.1. There exists a set 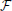 ⊂
⊂ 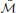 * which is a countable intersection of open and everywhere dense subsets of the complete metric space (
* which is a countable intersection of open and everywhere dense subsets of the complete metric space (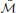 *, d) such that for each {Bt}∞t = 1 ∈
*, d) such that for each {Bt}∞t = 1 ∈ 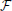 , the following properties hold:
, the following properties hold:
(a) there exists a unique point x ∈ K such that Bt(x) = x for all integers t ≥ 1;
(b) if t ≥ 1 is an integer and y ∈ K satisfies Bt(y) = y, then y = x;
(c) for each ϵ > 0 and each M > 0, there exist a number δ > 0 and a neighborhood 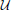 of {Bt}∞t = 1 in the metric space
of {Bt}∞t = 1 in the metric space 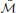 * such that if {Ct}∞t = 1 ∈
* such that if {Ct}∞t = 1 ∈ 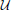 , t ∈ {1, 2, …}, and if y ∈ BK(θ, M) satisfies ρ(y, Ct(y)) ≤ δ, then ρ(y, x) ≤ ϵ;
, t ∈ {1, 2, …}, and if y ∈ BK(θ, M) satisfies ρ(y, Ct(y)) ≤ δ, then ρ(y, x) ≤ ϵ;
(d) for each ϵ > 0 and each M > 0, there exist a neighborhood 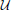 of {Bt}∞t = 1 in the metric space
of {Bt}∞t = 1 in the metric space 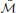 *, a number δ > 0 and a natural number q such that if {Ct}∞t = 1 ∈
*, a number δ > 0 and a natural number q such that if {Ct}∞t = 1 ∈ 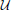 , m ≥ q is an integer, r: {1, …, m} → {1, 2, …}, and if {xi}mi = 0 ⊂ K satisfies
, m ≥ q is an integer, r: {1, …, m} → {1, 2, …}, and if {xi}mi = 0 ⊂ K satisfies
and
then
2. Proof of Theorem 1.1
Elements of the space 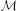 will occasionally be denoted by a boldface letters: A = {At}∞t = 1, B = {Bt}∞t = 1, C = {Ct}∞t = 1, respectively.
will occasionally be denoted by a boldface letters: A = {At}∞t = 1, B = {Bt}∞t = 1, C = {Ct}∞t = 1, respectively.
Let A = {At}∞t = 1 ∈ 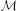 * and γ ∈ (0, 1). There exists a point xA ∈ K such that
* and γ ∈ (0, 1). There exists a point xA ∈ K such that
For each integer t ≥ 1 and each x ∈ K, set
By (1.1), (2.1), and (2.2), for all integers t ≥ 1 and all points x, y ∈ K,
and
In view of (2.2–2.4),
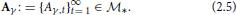
Let n be a natural number. Fix a number
a number
a positive number
and an integer
There exists an open neighborhood V(A, γ, n) of {Aγ, t}∞t = 1 in 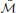 * such that
* such that
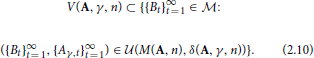
Assume that
is an integer,
and that a sequence {xi}mi = 0 ⊂ K satisfies
and
We now show by induction that for all integers i = 0, …, m,
and if i < m, then
Assume that p ∈ {0, …, m − 1}, (2.16) and (2.17) hold for all i = 0, …, p and that (2.18) holds for all nonnegative integers i < p. [Note that in view of (2.6), (2.7), and (2.14), our assumption holds for p = 0]. It follows from (2.3), (2.4), and (2.15) that
By (2.17), which holds for i = p, (1.2), (2.10), and (2.11),
Relations (2.19) and (2.20) imply that
Thus, (2.18) holds for i = p. It follows from (2.16), which holds for i = p, (2.6), (2.8), and (2.21) that
By the above relation and (2.7),
Hence (2.16) and (2.17) hold for i = p + 1 and the assumption made for p also holds for p + 1. Therefore, our assumptions hold for p = m, (2.16) and (2.17) hold for all i = 0, …, m, and (2.18) holds for all i = 0, …, m − 1.
We claim that for all i = q(A, γ, n), …, m,
First we show that there exists i ∈ {0, …, q(A, γ, n)} such that (2.22) holds.
Assume the contrary. Then
By (2.8), (2.18), and (2.23), for all integers i = 0, …, q(A, γ, n) − 1,
In view of the above inequality and (2.16),
and so,
This contradicts (2.9). The contradiction we have reached proves that there indeed exists an integer j ∈ {0, …, q(A, γ, n)} such that
Next we claim that (2.2) holds for all integers i ∈ {j, …, m}.
Indeed, by (2.24), inequality (2.22) is true for i = j. Now assume that i ∈ {j, …, m}, i < m and (2.22) holds. There are two cases:
Assume now that (2.25) holds. In view of (2.8), (2.18), and (2.25),
Assume that (2.26) holds. Then it follows from (2.8), (2.18), (2.22), and (2.26) that
Thus, in both cases,
This means that we have shown by induction that (2.22) is indeed valid for all i = q(A, γ, n), …, m. Clearly, we have proved that the following property holds:
(P) For each
each integer m ≥ q(A, γ, n), each
and each sequence {xi}mi = 0 ⊂ K which satisfies
and
we have
Set
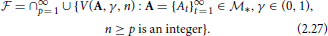
By (1.1), (2.1), and (2.2), for each A = {At}∞t = 1 ∈ 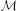 *, each γ ∈ (0, 1), each integer t ≥ 1 and each x ∈ K, we have
*, each γ ∈ (0, 1), each integer t ≥ 1 and each x ∈ K, we have
In view of (1.2) and (2.28),
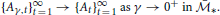
When combined with (2.27), this implies that 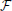 is a countable intersection of open and everywhere dense subsets of
is a countable intersection of open and everywhere dense subsets of 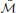 *.
*.
Assume that

and M, ϵ > 0. Choose a natural number p such that
By (2.27) and (2.29), there exist
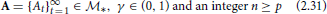
such that
Let
let t ≥ 1 be an integer and consider the sequence {Bit(x)}∞i = 0. By (2.30)–(2.33) and property (P) (applied to {Cs}∞s = 1 = {Bs}∞s = 1 and r(j) = t, j = 1, 2, …), for all integers i ≥ q(A, γ, n), we have
Since ϵ is an arbitrary positive number, we conclude that for each point z ∈ BK(θ, M) and each integer t ≥ 1, {Bit(z)}∞i = 0 is a Cauchy sequence. Since M is any positive number, we see that for each integer t ≥ 1 and each z ∈ K, there exists
in (X, ρ). In view of (3.34), for every integer t ≥ 1 and every z ∈ BK(θ, M),
This implies that for each pair of points z1, z2 ∈ BK(θ, M) and for each pair of natural numbers t1, t2,
Since ϵ, M are arbitrary positive numbers, we may conclude that for each pair of integers t1, t2 ≥ 1 and each pair of points z1, z2 ∈ K,
Let x ∈ K be such that
In view of (2.35),
It immediately follows from (2.35) and (2.36) that properties (a) and (b) hold. We claim that property (c) also holds.
Let
and assume that
Set
It follows from (2.37) and (2.38) that for all integers t ≥ 1,
By (2.30), (2.31), (2.37–2.39) and property (P) applied to any integer m ≥ q(A, γ, n) and xi = yi, i = 0, …, m,
and
In view of (2.30), (2.31), (2.34), (2.35), and (2.40),
Thus, property (c) does hold, as claimed.
Finally, we show that property (d) holds too. It follows from (2.34) and (2.35) that
Assume that
let m ≥ q(A, γ, n) be an integer, r: {1, …, m} → {1, 2, …}, and let {xi}mi = 0 ⊂ K satisfy
and
By the relations above and property (P),
It now follows from (2.30), (2.31), (2.41), and (2.42) that for all integers i = q(A, γ, n), …, m,
Thus, property (d) indeed holds. This completes the proof of Theorem 1.1.
Conflict of Interest Statement
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Acknowledgments
SR was partially supported by the Israel Science Foundation (Grant No. 389/12), by the Fund for the Promotion of Research at the Technion and by the Technion General Research Fund.
References
1. Goebel K, Reich S. Uniform Convexity, Hyperbolic Geometry, and Nonexpansive Mappings. New York, NY; Basel: Marcel Dekker (1984).
3. Reich S, Zaslavski AJ. Convergence of generic infinite products of nonexpansive and uniformly continuous operators. Nonlin Anal. (1999) 36:1049–65.
Keywords: fixed point, generic property, hyperbolic metric space, infinite product, nonexpansive mapping
Citation: Reich S and Zaslavski AJ (2015) Generic convergence of infinite products of nonexpansive mappings with unbounded domains. Front. Appl. Math. Stat. 1:4. doi: 10.3389/fams.2015.00004
Received: 20 March 2015; Accepted: 10 April 2015;
Published: 11 May 2015.
Edited by:
Jin Liang, Shanghai Jiao Tong University, ChinaReviewed by:
Ming Tian, Civil Aviation University of China, ChinaYekini Shehu, University of Nigeria, Nigeria
Copyright © 2015 Reich and Zaslavski. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) or licensor are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Alexander J. Zaslavski, Department of Mathematics, Technion – Israel Institute of Technology, Amado Mathematics Building, Haifa 32000, Israel,YWp6YXNsQHRlY2h1bml4LnRlY2huaW9uLmFjLmls
 Simeon Reich
Simeon Reich Alexander J. Zaslavski
Alexander J. Zaslavski