The Correlation between the Total Magnetic Flux and the Total Jet Power

Magnetic field threading a black hole ergosphere is believed to play the key role in both driving the powerful relativistic jets observed in active galactic nuclei and extracting the rotational energy from a black hole via Blandford-Znajek process. The magnitude of magnetic field and the magnetic flux in the vicinity of a central black hole is predicted by theoretical models. On the other hand, the magnetic field in a jet can be estimated through measurements of either the core shift effect or the brightness temperature. In both cases the obtained magnetic field is in the radiating domain, so its direct application to the calculation of the magnetic flux needs some theoretical assumptions. In this paper we address the issue of estimating the magnetic flux contained in a jet using the measurements of a core shift effect and of a brightness temperature for the jets, directed almost at the observer. The accurate account for the jet transversal structure allow us to express the magnetic flux through the observed values and an unknown rotation rate of magnetic surfaces. If we assume the sources are in a magnetically arrested disk state, the lower limit for the rotation rate can be obtained. On the other hand, the flux estimate may be tested against the total jet power predicted by the electromagnetic energy extraction model. The resultant expression for power depends logarithmically weakly on an unknown rotation rate. We show that the total jet power estimated through the magnetic flux is in good agreement with the observed power. We also obtain the extremely slow rotation rates, which may be an indication that the majority of the sources considered are not in the magnetically arrested disk state.

1. Introduction

One of the key issues of theoretical modeling of relativistic jets is determining the magnetic field magnitude. There are several theoretical ways to estimate the latter. The Eddington magnetic field (Beskin, 2010) sets up an upper limit on the magnetic field magnitude in the vicinity of a black hole, since it is set by equipartition of magnetic field density and the total energy density in the accreting plasma that is needed to support the Eddington luminosity. The state of magnetically arrested disk (MAD) (Narayan et al., 2003; Tchekhovskoy et al., 2011; McKinney et al., 2012) sets up the limiting magnetic field that can be accreted onto a black hole basing on an assumption that in such a state the pressure of previously accreted magnetic field can affect the dynamical process of accretion itself.

There are observational means to evaluate the magnetic field in a jet. Blazar spectrum is successfully modeled by the synchrotron self-Compton model. The lower part of the spectrum is dominated by synchrotron radiation of relativistic plasma in a jet magnetic field. Thus, the high-resolution radio interferometry observations provide us with data for unveiling the physical conditions at the very jet origin—in so called radio core. The way to estimate the magnetic field amplitude through the observations is measurements of core shift effect together with several theoretical assumptions on the radiating volume properties (Lobanov, 1998). However, the measurement of radio flux itself, or, equally, the brightness temperature, can provide us with the instrument to probe the magnetic field magnitude (Zdziarski et al., 2015; Nokhrina, 2017).

The magnetic field estimates is an important parameter that allows to test the theoretical models against observations. Zamaninasab et al. (2014) used the magnetic field measurements to calculate the flux and to show that the total flux is in accordance with the magnetically arrested state of the sources. In this paper we make use of the brightness temperature and core shift measurements coupled with the transversal jet model to express the magnetic field magnitude and the magnetic flux contained in a blazar jet through the rotation rate of magnetic surfaces. Our aim is to compare the magnetic flux in a jet against theoretically limited flux by MAD state and to estimate the rotation rate. We also test the obtained magnetic flux against the observed jet power. If the jet power P_Ψ is fully determined by the electromagnetic energy extraction mechanism, so we denote it with the subscript Ψ, than it can be expressed as Beskin (2010)

$\begin{array}{ll}{P}_{\Psi }={\left(\frac{\Psi a}{\pi r_{\text{g}}}\right)}^{2}c,\hfill & (1)\end{array}$

where Ψ is the total magnetic flux, r_g is a gravitational radius, c is a speed of light, and the rotation rate a = r_g/R_L is a ratio of a gravitational radius to the light cylinder radius R_L.

Although there are estimates for magnetic field amplitude (Lobanov, 1998; Hirotani, 2005; OŚullivan and Gabuzda, 2009; Nokhrina et al., 2015; Zdziarski et al., 2015; Nokhrina, 2017), it cannot be explicitly used for flux calculation. Indeed, the magneto hydrodynamical theoretical and numerical modeling (see e.g., Lyubarsky, 2009; Nokhrina et al., 2015; Bromberg and Tchekhovskoy, 2016) show that the toroidal magnetic field is greater than the poloidal one outside the light cylinder radius. Thus, measurements provide us with the magnitude of the toroidal magnetic field, while the poloidal one is needed to estimate the total magnetic flux (Zamaninasab et al., 2014).

2. Magnetic Flux in a Jet

The observed flux, or observed brightness temperature, can be used to estimate the magnetic field in the radiating domain, and, thus, the magnetic flux. The blazar spectrum in radio band is accurately modeled within the self-absorbed synchrotron model (see e.g., Abdo et al., 2011). The simplest model for the source is a uniform sphere with chaotic magnetic field B and relativistic electrons with the power-law energy distribution described by the amplitude particle number density k_e and spectral index p:

$\begin{array}{ll}dn(\gamma )=k_{\text{e}}{\gamma }^{-p}d\gamma ,\hfill & (2)\end{array}$

γ ∈ [γ_min, γ_max] (Gould, 1979), where γ is a Lorentz factor of relativistic plasma. The observed spectral flux S_ν at the frequency ν for the optically thick uniform self-absorbed source of radius R at the distance d can be written using the spectral photon emission rate ρ_ν and effective absorption coefficient æ_ν as:

$\begin{array}{ll}{S}_{\nu }=\pi \hslash \nu \frac{{\rho }_{\nu }}{{\text{æ}}_{\nu }}\frac{R^2}{d^2}u(2R{\text{æ}}_{\nu }),\hfill & (3)\end{array}$

and the function of the optical depth u(2Ræ_ν) is defined by Gould (1979). The emission and absorption coefficients for the self-absorbed synchrotron model ρ_ν and æ_ν are the functions of the magnetic field B and of particle number density amplitude k_e. These coefficients, written in a jet frame, i.e., in a frame where the electric field vanishes, are:

$\begin{array}{ll}{\rho }_{\nu }=4\pi {\left(\frac{3}{2}\right)}^{(p-1)/2}a(p)\alpha k_{\text{e}}{\left(\frac{{\nu }_{\text{B}}}{\nu }\right)}^{(p+1)/2},\hfill & (4)\end{array}$

$\begin{array}{ll}{\text{æ}}_{\nu }=c(p)r_0^2{k}_{\text{e}}\left(\frac{{\nu }_0}{\nu }\right){\left(\frac{{\nu }_{\text{B}}}{\nu }\right)}^{(p+2)/2}.\hfill & (5)\end{array}$

Here ν_B = eB/mc is a gyrofrequency in the jet frame, ℏ is the Planck constant, α = e²/ℏc is the fine structure constant, and the functions a(p) and c(p) of the electron distribution spectral index p are defined in Gould (1979). The spectral flux depends on the magnetic field amplitude, while the particle number density amplitude defines the maximum of a function u and, thus, the position of the observed radio core—the domain where the optical depth τ is equal to unity. So, the spectral flux measurement provide us with an instrument to evaluate the magnetic field in a source. The spectral flux may be expressed through the brightness temperature T_b as

$\begin{array}{ll}{S}_{\nu }=\frac{2\pi {\nu }^2{\theta }^2}{c^2}{k}_{\text{B}}{T}_{\text{b}},\hfill & (6)\end{array}$

where θ is the angular size of a radiating domain. Thus one can express the magnetic field amplitude in a source having measured brightness temperature. The method was first applied by Zdziarski et al. (2015) to check the magnetic field amplitude in AGN radio cores independently of the equipartition assumption. Equating the right-hand sides of Equations (3) and (6), and expressing the jet frame values through the nucleus frame values, we obtain for p = 2 the magnetic field (Zdziarski et al., 2015, see also details in Nokhrina, 2017)

$\begin{array}{ll}\left(\frac{B_{\text{uni}}}{\text{G}}\right)=7.4·{10}^{-4}\frac{\Gamma \delta }{1+z}\left(\frac{{\nu }_{\text{obs}}}{\text{GHz}}\right){\left(\frac{T_{\text{b},\text{obs}}}{{10}^{12}\text{K}}\right)}^{-2}.\hfill & (7)\end{array}$

Here Γ is a flow bulk Lorentz factor, z is a source redshift, and δ is a Doppler factor.

On the other hand, the measurements of core shift effect (Lobanov, 1998; Hirotani, 2005; OŚullivan and Gabuzda, 2009; Zamaninasab et al., 2014) provides the following expression for the magnetic field amplitude B_cs at 1 pc distance from the central source:

$\begin{array}{ll}\left(\frac{B_{\text{cs}}}{\text{G}}\right)=0.17{\left(\frac{{\eta }_{\text{cs}}}{\text{mas}\text{GHz}}\right)}^{0.75}{\left(\frac{D_{\text{L}}}{\text{Gpc}}\right)}^{0.75}\frac{\Gamma }{{\chi }^{0.25}{(1+z)}^{0.75}{\sin }^{0.5}\phi {\delta }^{0.5}},\hfill & (8)\end{array}$

here η_cs is a coefficient determining the slope of the apparent core position dependence on the inverse observation frequency:

$\begin{array}{ll}\left(\frac{\Delta r}{\text{mas}}\right)=\left(\frac{{\eta }_{\text{cs}}}{\text{mas}\text{GHz}}\right){\left(\frac{{\nu }_{\text{obs}}}{\text{GHz}}\right)}^{-1}.\hfill & (9)\end{array}$

χ is the jet opening angle that may obtained having the measured in Pushkarev et al. (2009) apparent opening angle χ_app as χ = χ_appsinφ/2, D_L is a luminosity distance, the bulk plasma motion Lorentz factor is Γ, δ is the Doppler factor, and the observation angle φ = Γ⁻¹ (Cohen et al., 2007). The Expression (Equation 8) has been obtained under the same assumption of synchrotron self-absorbed source, but the method utilizes the core shift effect—the shift of the observed radio core on different frequencies. This shift is due to the fact that the surface of optical depth τ = 1 is situated at different distance from the central source for each frequency. We must stress that the relation (Equation 7) uses only the synchrotron self-absorbed source model, but the position of the radio core in the model is not known. If we use the core shift effect, we may also obtain the core position (Nokhrina, 2017):

$\begin{array}{ll}\left(\frac{r_{\text{core}}}{\text{pc}}\right)=\frac{4.85}{\sin \phi {(1+z)}^2}{\left(\frac{{\nu }_{\text{obs}}}{\text{GHz}}\right)}^{-1}\left(\frac{{\eta }_{\text{cs}}}{\text{mas}\text{GHz}}\right)\left(\frac{D_{\text{L}}}{\text{Gpc}}\right).\hfill & (10)\end{array}$

The relation for B_cs (Equation 8) has been obtained with more assumptions: equipartition between magnetic field energy and plasma energy assumption, and the Blanford-Königl model (Blanford and Königl, 1979) B(r) = B₁(r₁/r), $n(r)=n_1{(r_1/r)}^2$, where n₁ and B₁ are particle number density and magnetic field magnitude at distance r along the jet equal to r₁ = 1 pc. Relation (Equation 8) provides the magnetic field amplitude together with its position along the jet.

Both Expressions (Equation 7) and (Equation 8) are based on the model of uniform radiating sphere (Gould, 1979). In particular, such a model does not allow us to estimate the total magnetic flux, contained in a jet—one of the important values, defining the total jet power, and the value that could be restricted by the magnetically arrested disk model. Indeed, as the toroidal magnetic field B_φ dominates the major part of a jet, it is the toroidal component of a field we imply by the spectral flux measurement. However, it is the poloidal component B_P that carries the magnetic flux. However, the transversal modeling of field profiles allow us in a simple case of blazar jets, i.e., jets pointing almost directly at us, to calculate the flux from non-homogeneous cylindrical self-absorbed synchrotron source, and to correlate the measured magnetic field amplitude with the poloidal field in a jet that defines the total flux. Indeed, it has been shown by Lyubarsky (2009), that the relation

$\begin{array}{ll}{B}_{\text{P}}=B_{\phi }\frac{R_{\text{L}}}{r_{\perp }}\hfill & (11)\end{array}$

holds outside the light cylinder R_L = c/Ω_F. Further we model the transversal magnetic field and particle number density profiles as follows. Inside the light cylinder the poloidal magnetic field remains almost constant (Beskin and Nokhrina, 2009), while B_φ = B₀r_⊥/R_L. Both numerical and semi-analytical modeling (Nokhrina et al., 2015; Bromberg and Tchekhovskoy, 2016) show, that outside the light cylinder the power-law is a good approximation for magnetic field and particle number density profiles across the jet for small opening angles. We set

$\begin{array}{ll}{B}_{\text{P}}=\{\begin{array}{c}{B}_0,r_{\perp }\le R_{\text{L}},\\ B_0{\left(\frac{R_{\text{L}}}{r_{\perp }}\right)}^2,R_{\text{L}}<r_{\perp }\le R_{\text{j}},\end{array}\hfill & (12)\end{array}$

$\begin{array}{ll}{B}_{\phi }=\{\begin{array}{c}{B}_0\frac{r_{\perp }}{R_{\text{L}}},r_{\perp }\le R_{\text{L}},\\ B_0\left(\frac{R_{\text{L}}}{r_{\perp }}\right),R_{\text{L}}<r_{\perp }\le R_{\text{j}}.\end{array}\hfill & (13)\end{array}$

$\begin{array}{ll}n=\{\begin{array}{c}{n}_0,r_{\perp }\le R_{\text{L}},\\ n_0{\left(\frac{R_{\text{L}}}{r_{\perp }}\right)}^2,R_{\text{L}}<r_{\perp }\le R_{\text{j}},\end{array}\hfill & (14)\end{array}$

where B₀ and n₀ are the magnetic field and particle number density amplitudes, i.e., magnitudes at the light cylinder R_L.

For the simplest case of a jet pointing almost at us, when the radiation domain may be treated as a stratified cylinder with the profiles (Equations 12–14), we calculate the spectral flux S_ν (see details in Nokhrina, 2017). Equating the obtained S_ν to the expression (Equation 6), we obtain the following relation for the amplitude magnetic field B₀:

$\begin{array}{ll}\left(\frac{B_0}{\text{G}}\right)=6.4\times {10}^{-4}\frac{R_{\text{j}}}{R_{\text{L}}}\frac{\Gamma \delta }{1+z}\left(\frac{{\nu }_{\text{obs}}}{\text{GHz}}\right){\left(\frac{T_{\text{b},\text{obs}}}{{10}^{12}\text{K}}\right)}^{-2}.\hfill & (15)\end{array}$

Here the fast rotation ΓR_L ≪ R_j is assumed. While R_j can be estimated through observations, the light cylinder radius is usually unknown. However, its value can be somewhat restricted by theoretical models predicting that Blandford-Znajek process works effectively for R_L of the order of 2r_g (Blanford and Znajek, 1977; Tchekhovskoy et al., 2012). Still, the value B₀ cannot be readily extracted from the observations.

The total flux Ψ in a jet with given cross-section magnetic filed profile is defined as

$\begin{array}{ll}\Psi =2\pi {\displaystyle {\int }_0^{R_{\text{j}}}{B}_{\text{P}}}{r}_{\perp }d{r}_{\perp }.\hfill & (16)\end{array}$

Using the magnetic field profile (Equation 12) we obtain

$\begin{array}{ll}\Psi =\pi B_0{R}_{\text{L}}^2\left(1+2\ln \frac{R_{\text{j}}}{R_{\text{L}}}\right).\hfill & (17)\end{array}$

Substituting explicitly B₀R_L into Equation (17) and using the correlation B₀R_L = 0.86B_uniR_j following from Equations (7) and (15), we obtain the relation for the magnetic flux:

$\begin{array}{ll}\Psi =2.7B_{\text{uni},\text{ cs}}{R}_{\text{j}}\frac{r_{\text{g}}}{a}\left[1+2\ln \frac{R_{\text{j}}a}{r_{\text{g}}}\right]=\frac{{\Psi }_a}{a}.\hfill & (18)\end{array}$

Here one may use B_cs or B_uni, since both values are obtained under the same assumptions on the geometry and structure of radiating domain. The amplitude magnetic flux Ψ_a = aΨ. The Equation (18) coincides with the expression for the magnetic flux in Zamaninasab et al. (2014). The expression (Equation 18) for the flux depends inversely on a rotation rate a, because the dependence on the physical values in square brackets is logarithmically weak and can be neglected. Taking the fiducial value for $R_{\text{j}}/r_{\text{g}}~10^3$, we take the expression in square brackets being of the order of a few to ten.

3. The Jet Power and the Rotation Rate

We apply the obtained expression for the flux (Equation 18) to test it against the following theoretical predictions. If we assume that most of the sources are in MAD state, we can compare the amplitude flux Ψ_a and Ψ_MAD and obtain the rotation parameter a = Ψ_a/Ψ_MAD. However, we must bear in mind that the energy losses mechanism Blanford and Znajek (1977) works effectively for relatively high rotation rates a > 0.5. Thus, with the difference in Ψ_MAD and Ψ_a is greater, we might think that the source is not in the MAD state.

We also use the obtained flux (Equation 18) to calculate the total jet power given by the Equation (1). As the Expression (Equation 1) depends on the product Ψa = Ψ_a (Equation 18) that depends on a logarithmically weakly only through the term 1 + 2 ln(R_ja/r_g), so does the total power. So this result is independent on the assumption of the particular value for a. That is why such a test may be important for the flux determination. We do it for the magnetic field estimated by two methods: the brightness temperature measurements and the core shift measurements in order to compare the two methods.

We have found 48 sources meeting the following conditions: (i) the observational angle of a jet must be small enough for the model of head-on jet for B_uni can be applied; (ii) the source has a measured core shift, central black hole mass, and the apparent opening angle. We use the following samples: we take the brightness temperature measured by Kovalev et al. (2005) and the core shifts by Pushkarev et al. (2012). The apparent velocity β_app we take from Lister et al. (2009). We also use the black hole masses M_BH and the accretion luminosities L_acc collected by Zamaninasab et al. (2014). For the unknown Lorentz factor we use Γ = σ_M, and the Michel's magnetization parameter σ_M has been evaluated by Nokhrina et al. (2015), We use for the observed opening angle the results from Pushkarev et al. (2009). This is in contrast with Zamaninasab et al. (2014), where the causal connectivity across the jet Γ χ ~ 1 is assumed. We obtain the magnetic field magnitudes using the above values and Equations (7) and (8). On average, the values of B_uni is less and more scattered than values of B_cs, which is in agreement with results obtained in Zdziarski et al. (2015). To calculate the flux using B_cs we employ R_j = χ × 1 pc. For B_uni we use Equation (10), so we define R_j = r_coreχ.

One of the possible upper limits on the magnetic field amplitude in relativistic jets may be imposed by MAD model. Magnetically arrested disk is a disk in a state of equilibrium of the accretion rate and the pressure of magnetic field frozen in previously accreted matter (Narayan et al., 2003; Tchekhovskoy et al., 2011; McKinney et al., 2012). There is observational support of AGNs staying in MAD state (Zamaninasab et al., 2014). Equation (18) relate the total magnetic flux in a jet with the observable jet radius, magnetic field, gravitational radius (through the black hole mass estimates), and unknown rotation rate a. Setting Ψ_MAD as the upper limit on a magnetic flux, one can obtain the lower limit for the rotational rate of a central black hole. Here we compare the magnetic flux Ψ calculated with expression (Equation 18) for B_uni, obtained by the brightness temperature measurements, or B_cs obtained by core shift measurements, and the magnetic flux Ψ_MAD set by MAD model. In order to obtain the magnetic flux predicted by MAD Ψ_MAD, we use the equation (Zamaninasab et al., 2014)

$\begin{array}{ll}{\Psi }_{\text{MAD}}\approx 50\sqrt{\dot{M}{r}_{\text{g}}^{2}c},\hfill & (19)\end{array}$

where we use relation between disk luminosity $L_{\text{acc}}=\eta Ṁc^2$.

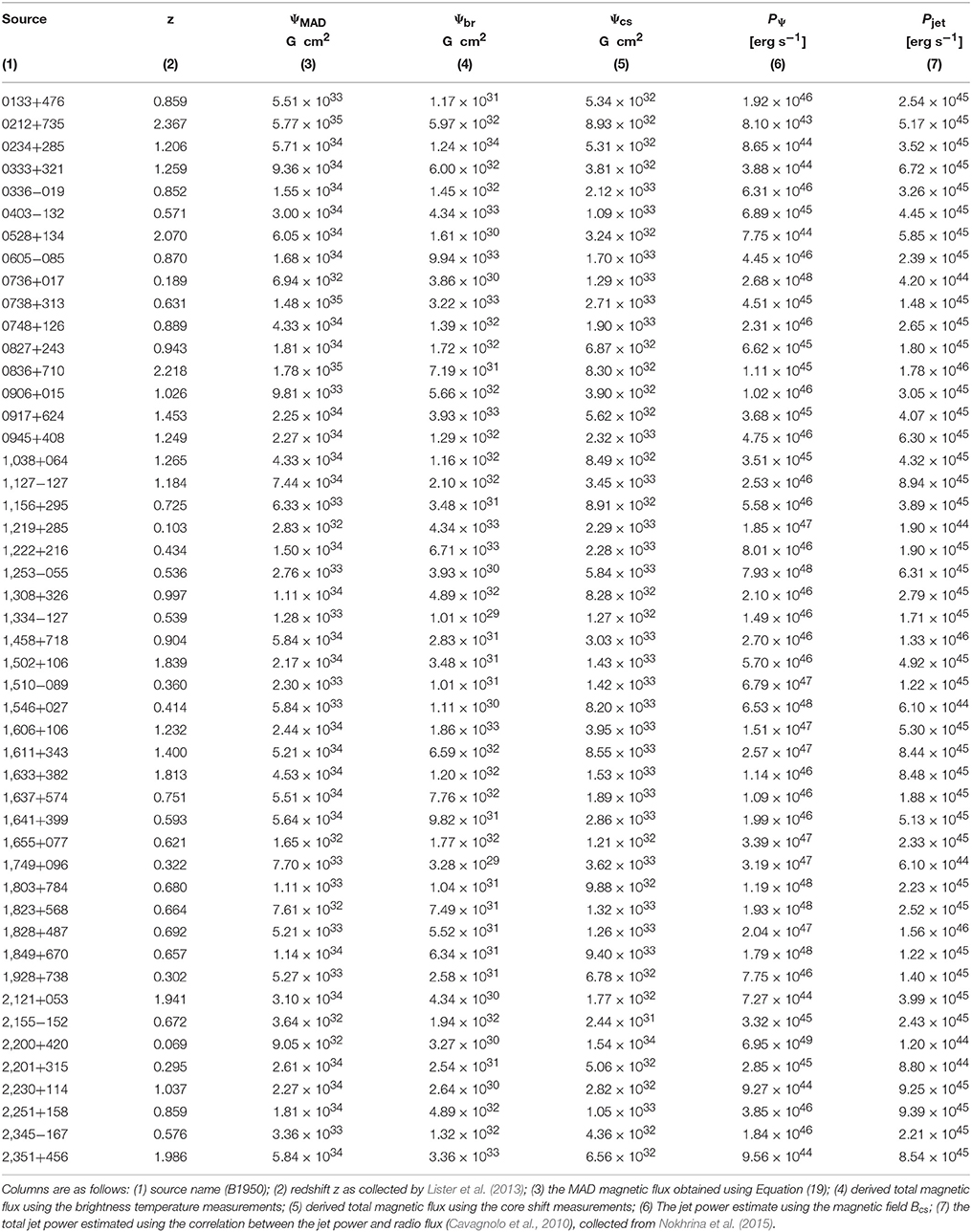
The results for Ψ calculated for a = 0.5 and Ψ_MAD are presented in Table 1. We present in the table the values for Ψ_MAD (Zamaninasab et al., 2014), the total magnetic flux obtained using brightness temperature measurements Ψ_br and core shift measurements Ψ_cs. We see the reasonable agreement between Ψ_br and Ψ_cs, although the former is more scattered. We see that aΨ ≪ Ψ_MAD for almost all the sources for magnetic filed estimates by both methods. If we assume that all the sources are in MAD state, the rotational rate of a black hole must be in a range (0.0001;0.1) for 36 sources. Only 12 have the rotational rate between 0.1 and 1. Thus, assumption of the sources being in the MAD state leads us to a conclusion that the rotation must be much less than the critical one.

TABLE 1

Table 1. Jet important observed and derived parameters.

Otherwise, we may assume that 36 sources have rotation parameters close to critical a ∈ [0.5; 1], but not in a MAD state. We must stress that the expressions for the magnetic field estimate through the core shift measurement are different here from the one used in Zamaninasab et al. (2014). In this paper the Equation (8) uses the assumtions from Nokhrina et al. (2015) of the total outflow magnetization equal to the unity. This condition means that the total Poynting flux in a core region is equal to the total bulk particle kinetic energy flux, with about 1% (Sironi et al., 2013) of radiating particles having the relativistic energy distribution (Equation 2). This assumption has been used to estimate maximal possible Lorentz factor of the flow (Nokhrina et al., 2015), which correlates very good with the Lorentz factor estimates basing the observed super-luminal velocities (Lister et al., 2009). In this point our approach differs from the one used by Zamaninasab et al. (2014).

In order to check our flux estimates, we test it against the total jet power (Equation 1). This result is robust under the model assumptions, since it the total power depends on a very weakly. The calculation of the total jet power for the obtained flux is in Table 1. We compare the total power P_Ψ, calculated substituting Equation (18) into Equation (1), with the jet power, estimated basing on the correlation of P_jet with the luminosities of jet radio band Cavagnolo et al. (2010):

$\begin{array}{ll}\left(\frac{P_{\text{jet}}}{{10}^{43}\text{erg}{\text{s}}^{-1}}\right)=3.5{\left(\frac{P_{200-400}}{{10}^{40}\text{erg}{\text{s}}^{-1}}\right)}^{0.64}.\hfill & (20)\end{array}$

We plot P_Ψ against the obtained with Equation (20) power P_jet in Figure 1. We observe the reasonable correlation of P_Ψ and P_jet. The histogram of the ratio of P_Ψ/P_jet is presented in Figure 2. We see that the ratio has a well determined peak around a few. Although it gives the systematic excess of P_Ψ over P_jet, we state that P_Ψ is in accordance with P_jet bearing in mind uncertainties in the determination of all the values including P_tot. The systematic excess may be attributed to the probable overestimating the magnetic field B_cs, the hints of which we see in discrepancy between B_cs and B_br, the latter being lower.

FIGURE 1

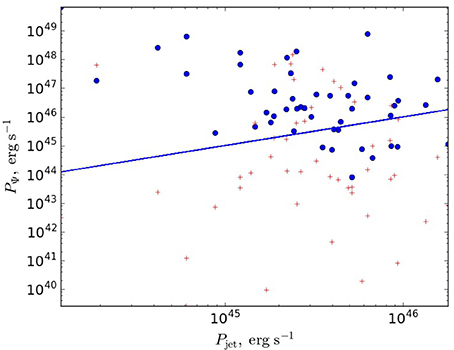
Figure 1. The jet power P_Ψ, calculated using magnetic flux, against the total kinetic jet power. The straight line is a theoretical prediction. The blue circle stand for the total flux obtained using the core shift effect, the red crosses are for the total flux obtained using the brightness temperature. The sources with the flux approximately equal to the MAD flux are in the upper left corner.

FIGURE 2

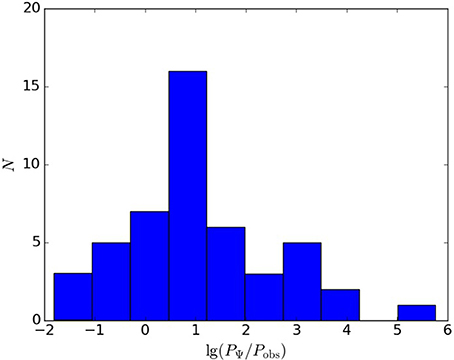
Figure 2. The histogram showing the number of sources with the ration of calculated power P_Ψ to the total jet power P_jet, the ratio is in the log-scale. We see the systematic excess of power estimated through the flux against the jet power by a factor of few.

We have also tested the jet power obtained with the flux determined by B_uni (see Figure 1). The second method provides systematically lower powers and more scattering. This is in agreement with the result by Zdziarski et al. (2015) who have found the scattering in magnetic field amplitude calculated with no equipartition assumption while still have the majority of sources having the equipartition magnetic field.

4. Summary

We have discussed estimates for the magnetic flux using the jet core magnetic field obtained through the brightness temperature measurements and by the core shift effect. Usually, any estimates of the magnetic field in a radiating domain of relativistic jet cannot be readily put into the expression for the magnetic flux. This is because theoretical modeling show, that the toroidal magnetic field dominates the poloidal magnetic field outside the light cylinder. Thus, the field we measure using synchrotron self-Compton model of radiation, must be toroidal, while the magnetic flux is determined by the poloidal one. Consideration of transversal field configuration is needed to estimate accurately the magnetic flux in a jet using the available evaluation of magnetic field magnitude through observations—either using the core-shift effect, or spectral flux measurements. In this work has been considered the simplest case—the transversal structure of jets observed with very small viewing angle.

We test the method of estimating the flux against the limiting flux determined by the magnetically arrested disk model. For 36 of 48 sources the obtained flux is much less than the MAD flux. This suggest either the extremely slow rotation rate a ∈ (0.0001; 0.1) or that the sources are not in MAD state. For 12 sources both fluxes coincide for a ∈ (0.1; 1)—the fast rotation that is needed for the efficient energy extraction from a black hole.

We also test the flux estimate against the total jet power determined by the electromagnetic mechanism of energy extraction. This result does not depend on the particular value for a, as the Expression (Equation 1) depends on the product of Ψ and a Ψ_a that can be estimated directly. In this case we see a good agreement between the total power determined by the flux and the total power obtained from the observations, with the distribution of powers ration being well peaked around a few.

Author Contributions

The results presented in the paper has been obtained by EN.

Funding

This work was supported by Russian Science Foundation, grant 16-12-10051.

Conflict of Interest Statement

The author declares that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Acknowledgments

The author thanks the referees for the suggestions which helped to improve the paper. This research has made use of data from the MOJAVE database that is maintained by the MOJAVE team (Lister et al., 2009).

References

Abdo, A., Ackermann, M., Ajello, M., Baldini, L., Ballet, J., Barbiellini, G., et al. (2011). Fermi large area telescope observations of markarian 421: the missing piece of its spectral energy distribution. Astrophys. J. 736:131. doi: 10.1088/0004-637X/736/2/131

CrossRef Full Text | Google Scholar

Beskin, V. (2010). Magnetohydrodynamic models of astrophysical jets. Phys. Uspekhi 53, 1199–1233. doi: 10.3367/UFNe.0180.201012b.1241

CrossRef Full Text | Google Scholar

Beskin, V., and Nokhrina, E. (2009). On the central core in MHD winds and jets. Month. Notices R. Astron. Soc. 397, 1486–1497. doi: 10.1111/j.1365-2966.2009.14964.x

CrossRef Full Text | Google Scholar

Blanford, R., and Königl, A. (1979). Relativistic jets as compact radio sources. Astrophys. J. 232, 34–48. doi: 10.1086/157262

CrossRef Full Text | Google Scholar

Blanford, R., and Znajek, R. (1977). Electromagnetic extraction of energy from kerr black holes. Month. Notices R. Astron. Soc. 179, 433–456. doi: 10.1093/mnras/179.3.433

CrossRef Full Text

Bromberg, O., and Tchekhovskoy, A. (2016). Relativistic mhd simulations of core-collapse GRB jets: 3d instabilities and magnetic dissipation. Month. Notices R. Astron. Soc. 456, 1739–1760. doi: 10.1093/mnras/stv2591

CrossRef Full Text | Google Scholar

Cavagnolo, K., McNamara, B., Nulsen, P., Carilli, C., Jones, C., and Brzan, L. (2010). A relationship between agn jet power and radio power. Astrophys. J. 720, 1066–1072. doi: 10.1088/0004-637X/720/2/1066

CrossRef Full Text | Google Scholar

Cohen, M., Lister, M., Homan, D., Kadler, M., Kellermann, K., Kovalev, Y., et al. (2007). Relativistic beaming and the intrinsic properties of extragalactic radio jets. Astrophys. J. 658, 232–244. doi: 10.1086/511063

CrossRef Full Text | Google Scholar

Gould, R. (1979). Compton and synchrotron processes in spherically-symmetric non-thermal sources. Astron. Astrophys. 76, 306–311.

Google Scholar

Hirotani, K. (2005). Kinetic luminosity and composition of active galactic nuclei jets. Astrophys. J. 619, 73–85. doi: 10.1086/426497

CrossRef Full Text | Google Scholar

Kovalev, Y., Kellermann, K., Lister, M., Homan, D., Vermeulen, R., Cohen, M., et al. (2005). Sub-milliarcsecond imaging of quasars and active galactic nuclei. IV. Fine-scale structure. Astron. J. 130, 2473–2505. doi: 10.1086/497430

CrossRef Full Text | Google Scholar

Lister, M., Aller, H., Aller, M., Cohen, M., Homan, D., Kadler, M., et al. (2009). MOJAVE: monitoring of jets in active galactic nuclei with VLBA experiments. V. Multi-epoch VLBA images. Astron. J. 137, 3718–3729. doi: 10.1088/0004-6256/137/3/3718

CrossRef Full Text | Google Scholar

Lister, M., Aller, M., Aller, H., Homan, D., Kellermann, K., Kovalev, Y., et al. (2013). MOJAVE. X. Parsec-scale jet orientation variations and superluminal motion in active galactic nuclei. Astron. J. 146, 120–142. doi: 10.1088/0004-6256/146/5/120

CrossRef Full Text | Google Scholar

Lobanov, A. (1998). Ultracompact jets in active galactic nuclei. Astron. Astrophys. 330, 79–89.

Google Scholar

Lyubarsky, Y. (2009). Asymptotic structure of poynting-dominated jets. Astrophys. J. 698, 1570–1589. doi: 10.1088/0004-637X/698/2/1570

CrossRef Full Text | Google Scholar

McKinney, J., Tchekhovskoy, A., and Blandford, R. (2012). General relativistic magnetohydrodynamic simulations of magnetically choked accretion flows around black holes. Month. Notices R. Astron. Soc. 423, 3083–3117. doi: 10.1111/j.1365-2966.2012.21074.x

CrossRef Full Text

Narayan, R., Igumenshchev, I., and Abramowicz, M. (2003). Magnetically arrested disk: an energetically efficient accretion flow. Publ. Astron. Soc. Jpn. 55, L69–L72. doi: 10.1093/pasj/55.6.L69

CrossRef Full Text | Google Scholar

Nokhrina, E. (2017). Brightness temperature - obtaining the physical properties of a non-equipartition plasma. Month. Notices R. Astron. Soc. 468, 2372–2381. doi: 10.1093/mnras/stx521

CrossRef Full Text | Google Scholar

Nokhrina, E., Beskin, V., Kovalev, Y., and Zheltoukhov, A. (2015). Intrinsic physical conditions and structure of relativistic jets in active galactic nuclei. Month. Notices R. Astron. Soc. 447, 2726–2737. doi: 10.1093/mnras/stu2587

CrossRef Full Text | Google Scholar

OŚullivan, S., and Gabuzda, D. (2009). Magnetic field strength and spectral distribution of six parsec-scale active galactic nuclei jets. Month. Notices R. Astron. Soc. 400, 26–42. doi: 10.1111/j.1365-2966.2009.15428.x

CrossRef Full Text

Pushkarev, A., Hovatta, T., Kovalev, Y., Lister, M., Lobanov, A., Savolainen, T., et al. (2012). MOJAVE: monitoring of jets in active galactic nuclei with VLBA experiments. IX. Nuclear opacity. Astron. Astrophys. 545:A113. doi: 10.1051/0004-6361/201219173

CrossRef Full Text | Google Scholar

Pushkarev, A., Kovalev, Y., Lister, M., and Savolainen, T. (2009). Jet opening angles and gamma-ray brightness of AGN. Astron. Astrophys. 507, L33–L36. doi: 10.1051/0004-6361/200913422

CrossRef Full Text | Google Scholar

Sironi, L., Spitkovsky, A., and Arons, J. (2013). The maximum energy of accelerated particles in relativistic collisionless shocks. Astrophys. J. 771:54. doi: 10.1088/0004-637X/771/1/54

CrossRef Full Text | Google Scholar

Tchekhovskoy, A., McKinney, J. C., and Narayan, R. (2012). General relativistic modeling of magnetized jets from accreting black holes. J. Phys. 372:012040. doi: 10.1088/1742-6596/372/1/012040

CrossRef Full Text | Google Scholar

Tchekhovskoy, A., Narayan, R., and McKinney, J. (2011). Efficient generation of jets from magnetically arrested accretion on a rapidly spinning black hole. Month. Notices R. Astron. Soc. 418, L79–L83. doi: 10.1111/j.1745-3933.2011.01147.x

CrossRef Full Text | Google Scholar

Zamaninasab, M., Clausen-Brown, E., Savolainen, T., and Tchekhovskoy, A. (2014). Dynamically important magnetic fields near accreting supermassive black holes. Nature 510, 126–128. doi: 10.1038/nature13399

PubMed Abstract | CrossRef Full Text | Google Scholar

Zdziarski, A., Sikora, M., Pjanka, P., and Tchekhovskoy, A. (2015). Core shifts, magnetic fields and magnetization of extragalactic jets. Month. Notices R. Astron. Soc. 451, 927–935. doi: 10.1093/mnras/stv986

CrossRef Full Text | Google Scholar