- 1Department of Linguistic, Sciences and Mathematics Education, University of Barcelona, Barcelona, Spain
- 2DISORA, Maribor, Slovenia
- 3HU University of Applied Sciences Utrecht, Utrecht, Netherlands
Introduction: The contemporary scientific literature indicates that numeracy is a multifaceted concept. The ongoing societal and technological transformations underscore the imperative to re-evaluate the attributes characterizing a numerate individual and the strategic initiatives that policymakers should devise and implement to ensure that individuals are not marginalized from participation in public and private domains due to their lack of numeracy proficiency. Numerous empirical investigations on numeracy consistently affirm its pivotal role in enabling individuals to engage autonomously across diverse contexts within their daily lives. However, numeracy’s fundamental role has often been neglected in our societies. The present study scrutinizes the overarching challenges associated with numeracy, particularly emphasizing the challenges regarding healthcare, finance, and the critical utilization and interpretation of data awareness.
Methods: A two-phase research framework was adopted to address this inquiry. A comprehensive literature review was conducted to discern the prevalent challenges regarding numeracy awareness. Subsequently, two illustrative case studies were undertaken in Slovenia and Spain to contrast and deliberate upon the insights derived from the literature review. Qualitative research methods were employed to engage in a nuanced exploration of the gathered data.
Results: This empirical analysis deduced guidelines aimed at enhancing awareness and ameliorating some of these challenges.
Discussion and Conclusion: We conclude that making visible the awareness that adults already have about numeracy in aspects of their lives, such as finance, health, or the use and critical interpretation of data, can give policymakers and curriculum developers clues to design effective numeracy programs to address the multifaceted challenges confronting contemporary society, both in the immediate and foreseeable future.
Introduction
In 2000, Coben wrote that some mathematics are invisible and that we tend to refer to them as “common sense,” “the mathematics that one can do but that one does not recognize as mathematics” (Coben, 2000b, p. 55). When we talk about numeracy, one of the main problems is that whatever numeracy is, it tends to go “unnoticed” because it is generally a skill that is not made visible. Despite many efforts to define and substantiate numeracy, it has always been considered as the “little sister” of literacy. This means that either it is omitted, or it appears as just another aspect of literacy. It turns out that many languages do not even have a word for the English concept of “numeracy,” which suggests the secondary role (or subsumed to literacy) attributed to the concept. Only recently attention has been drawn to this circumstance, even though there is already a tradition of more than 30 years of international studies to find out (or determine) the level of understanding and use of mathematics by adults (Coben et al., 2000; Safford-Ramus et al., 2016, 2018).
Numeracy is a much-disputed concept, which has been defined and analyzed on various occasions since it was used for the first time in the middle of the last century (Crowther, 1959). To this day, the OECD defines numeracy in the Program for the International Assessment of Adult Competencies (PIAAC) as “accessing, using and reasoning critically with mathematical content, information and ideas represented in multiple ways to engage in and manage the mathematical demands of a range of situations in adult life” [Organisation for Economic Co-operation and Development (OECD), 2021, p. 93]. This definition has been created to be operational because it is aimed at measuring the level of numeracy of adults in the countries participating in PIAAC. Still, it is not the only definition that exists. If we broaden our gaze, we find that what we now call numeracy was called “quantitative literacy” or “mathematical literacy” years ago and that only after a long process of conceptual transformations did the concept of numeracy end up being defined as a social practice; very far from that initial idea that appeared in the report of Crowther (1959), where numeracy was only identified with knowing how to perform a series of arithmetic operations using the existing algorithms correctly and appropriately. In recent years, numeracy has been integrated with cultural, social, personal, and emotional aspects. Below, we argue that it has become a multifaceted concept.
This article is the product of two case studies that have been carried out in Spain and Slovenia, two of the countries that participate in the Erasmus+ project Numeracy in Practice (NiP), which is currently running for the period 2022–2024. First, we indicate the research work that we present here and the methods that have been used to collect evidence and discuss it. Then, the different findings are presented, organized according to the main areas that have been used in the NiP project concerning numeracy awareness: general, financial, health and critical use and interpretation of media. Based on these four thematic foci, current challenges regarding numeracy awareness are identified. We finalize this paper with a reflection on the impact of this sort of work on future lines of action.
Rationale and research focus: numeracy as a multifaceted concept
Numeracy is a concept that goes beyond arithmetic content. It is considered a transversal competence, which is present not only in the different areas, contents, and processes of mathematics (problem-solving, communication, reasoning, representations, numerical thinking, measurement, geometry, probability, statistics, etc.). It is also part of what we need to take a critical look at our environment and be able to participate actively, informally, and autonomously. This, of course, poses many challenges, especially in today’s world, in which all of us face situations where we must read, understand information, perhaps calculate things, think or interpret numerical or mathematical information, make decisions, and even use technologies (Apps, programs, etc.). All of it contains a good amount of math (sometimes even invisibly, since we use the tool without thinking about the “math” it contains and the “math processing” it performs). Think, for example, of any smartphone or smartwatch App that solves for us a series of practical numeracy problems, such as calculating the restaurant tip, the time zone of the different international participants in an online meeting, the number of calories I must eat to be in the “acceptable” zone of the “body score,” the time at which I have to go to sleep, when the menstrual cycle is expected, and so forth. How much math does the smartwatch do without us knowing? What algorithms are programmed for us and which, often without thinking about it, we trust blindly without asking ourselves how they are made or if they fit our situation? The OECD states that numeracy, literacy, and digital skills are the pillars of the basic skills in the 21st Century [Organisation for Economic Co-operation and Development (OECD), 2021]. This statement is very strong because it shows that our society is undergoing a so-called “mathematisation.” Mathematics and numeracy are increasingly present in our forms of communication and action (Hoogland and Stoker, 2021; Hoogland and Díez-Palomar, 2022).
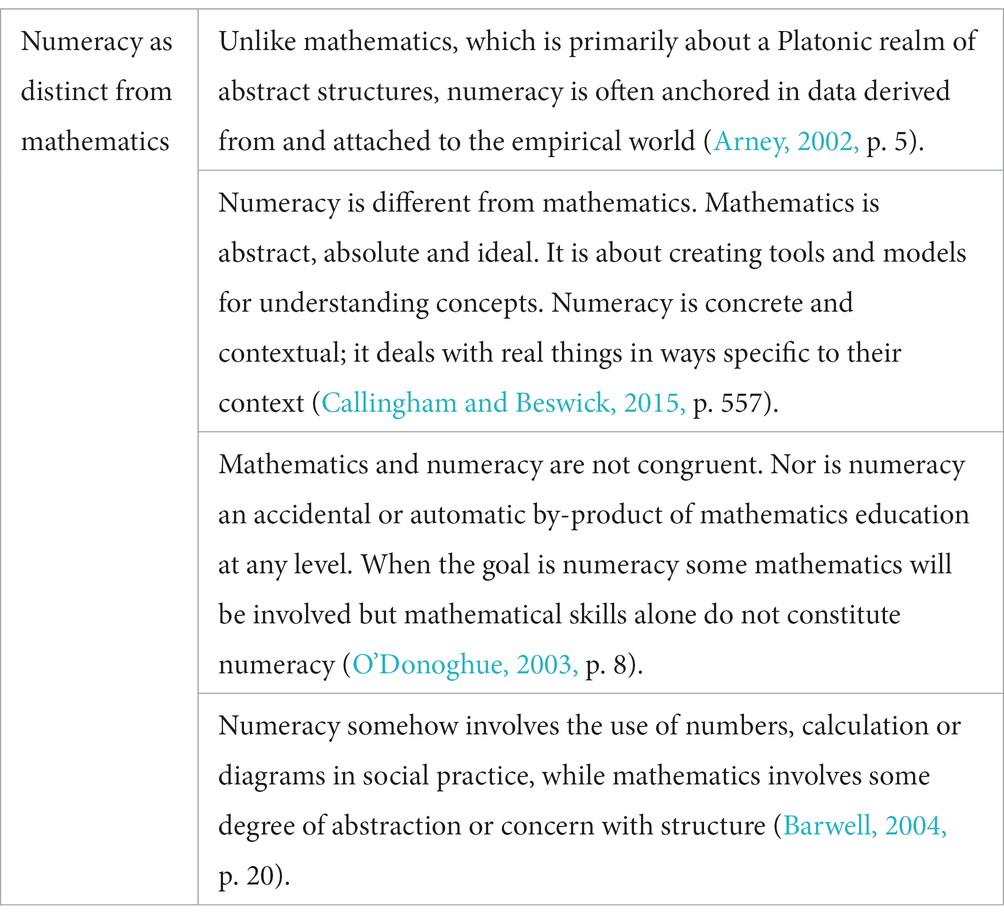
Several authors have endeavored to offer a concrete definition of numeracy. After more than 70 years of discussion, a widespread agreement is that numeracy is not just mathematics, it is more (Arney, 2002; O’Donoghue, 2003; Barwell, 2004; Callingham and Beswick, 2015). In Table 1, some relevant quotes are included endorsing this idea:
For many years, numeracy was associated with “basic arithmetic” until later on the recommendations from the international scientific community repeated and refined the Cockcroft’s rejection of letting numeracy means basic arithmetic (Cockcroft’s, 1982; Gal et al., 2009; Gal and Tout, 2014; Tout et al., 2017; Hoogland et al., 2018). Already in the last two decades of the last century, most authors expanded their point of view and began to see numeracy as a mathematical competence embedded in everyday life (Sewell, 1981; Withnall, 1994), moving from a notion of numeracy as “knowing” to a vision of this competence as “doing” (Hoogland et al., 2019). Coben, for example proposed the following definition of numeracy:
To be numerate means to be competent, confident, and comfortable with one’s judgments on whether to use mathematics in a particular situation and if so, what mathematics to use, how to do it, what degree of accuracy would be appropriate, and what the answer means in relation to the context (Coben, 2000b, p. 35; emphasis in the original; Coben et al., 2003, p. 10).
The article published by Coben (1992) (What do we need to know? Issues in numeracy research) was a turning point that laid the foundations for establishing a new field of research: the learning of mathematics by adults. The international forum Adults Learning Mathematics (ALM) was then created, and an effort to investigate the relationship between adults and mathematics from education was begun in a more rigorous and systematized manner. The OECD endorsed this effort by establishing a research agenda: the international surveys (the first one was the International Adult Literacy Survey—IALS—in 1996), which are still carried out today through the Program for the International Assessment of Adult Competencies, PIAAC (first cycle 2013–2016, second cycle 2018–2024).
What changes were experienced regarding numeracy? Fundamentally, numeracy came to be understood as a set of knowledge and mathematical processes integrated into the cultural, social, personal, and emotional lives of people. Numeracy began to be seen as a “mathematics literacy” (Frankenstein, 1998; de Lange et al., 1999; Gellert et al., 2001; Jablonka, 2003). Years later, at the 2003 ALM congress held in Strobl (Austria), Kaye (2003) presented a selection of definitions of numeracy with which a discussion was opened in which he discussed the convenience of abandoning the term numeracy and replacing it with the term “mathematics literacy.” Twenty years after that debate, it is evident that numeracy won the game. Despite the difficulties in defining its limits (even because there are languages with no comparable word to translate it), numeracy continues to be the international term used when referring to how adults use mathematics in their lives.
In recent years, the international scientific community has reached a certain consensus around the idea that numeracy must be understood as a social practice rather than a set of quantitative contents (numbers, symbols, etc.) or procedures (addition /subtraction structures, multiplication/division structures, proportional reasoning, etc.). Now, “numeracy” focuses on the social use given to these contents and processes (Figure 1).
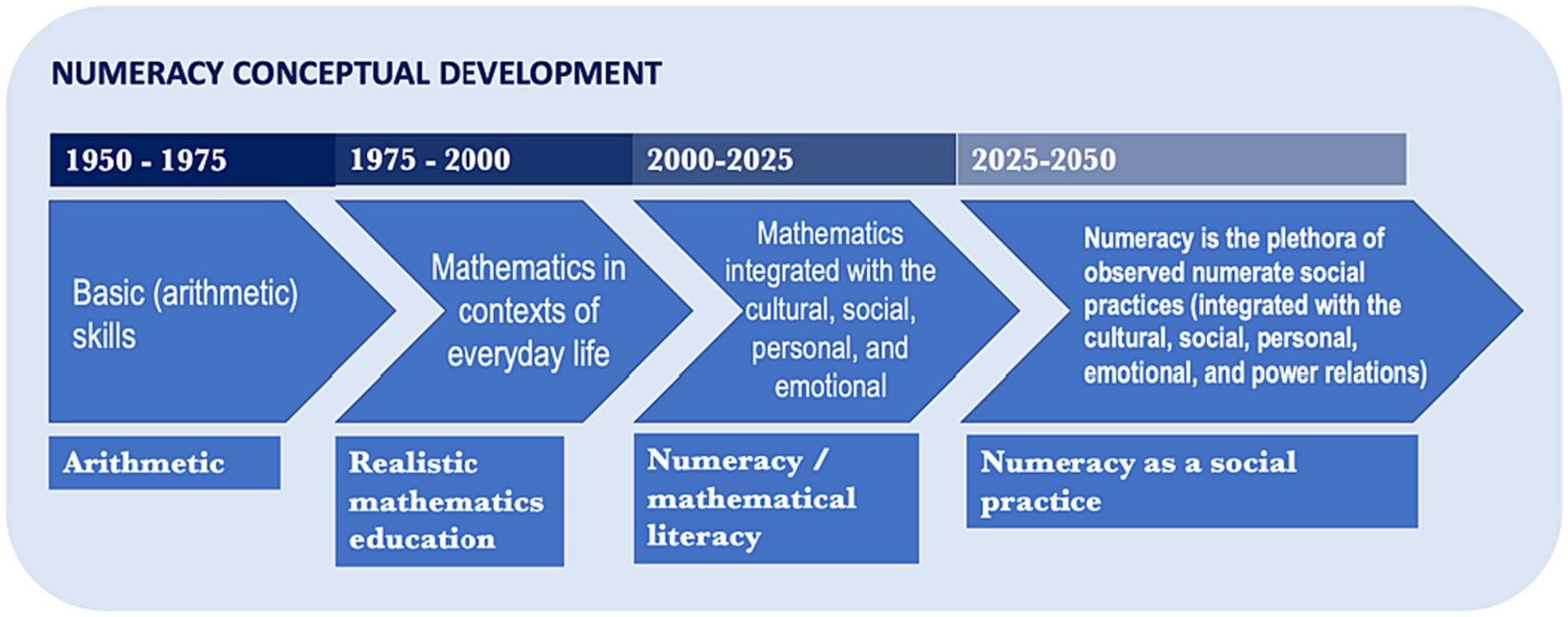
Figure 1. Numeracy conceptual development (Hoogland et al., 2019).
In this context, the Erasmus+ Project Numeracy in Practice that frames the study being presented in this article, the concept of numeracy is defined as a multifaceted concept, which cannot be understood only as a competence, as a set of knowledge or as a social practice alone. It is considered to be all these different aspects together. The concept of numeracy and numerate behavior encompasses more than just substantive mathematical knowledge and skills. It is about managing all kinds of situations that require mathematical insight, knowledge, and skills, as described in the PIAAC definition—competencies which enable individuals to take adequate actions in situations which need further elaboration. Higher-order skills, provisions about mathematics, and the situation in which mathematical activities occur play an essential role. The use of mathematical knowledge and skills (content) in daily life is always situation-specific (in context). The quality of the mathematical action depends on how the person relates himself to his or her mathematical knowledge and skills (dispositions) and the extent to which he/she can oversee and control a situation (higher-order skills).
Numerate behavior is permanently embedded in a context. Some numeracy takes place in everyday life, such as in daily activities of the individual, like cooking. Numeracy can also be in the context of work and professional settings, ranging from simple lists of numbers to complex algorithm-driven applications. In the framework of the second cycle of PIAAC, the contexts in which numeracy is defined include three areas: the personal, the work, and the societal/community (Tout et al., 2021).
On the other hand, being numerically competent involves using a series of higher-order skills. Many of those that appear in Figure 2 have already been mentioned in various frameworks on numeracy [Organisation for Economic Co-operation and Development (OECD), 2021]. There is also an overlap with the so-called 21st Century Skills. Every individual—whatever his/her cognitive abilities and experiences—uses higher-order skills. Everybody makes decisions, reasons, and processes information. These skills include managing and analyzing situations, but also critical thinking and problem-solving and obviously, they can also be improved by many individuals.
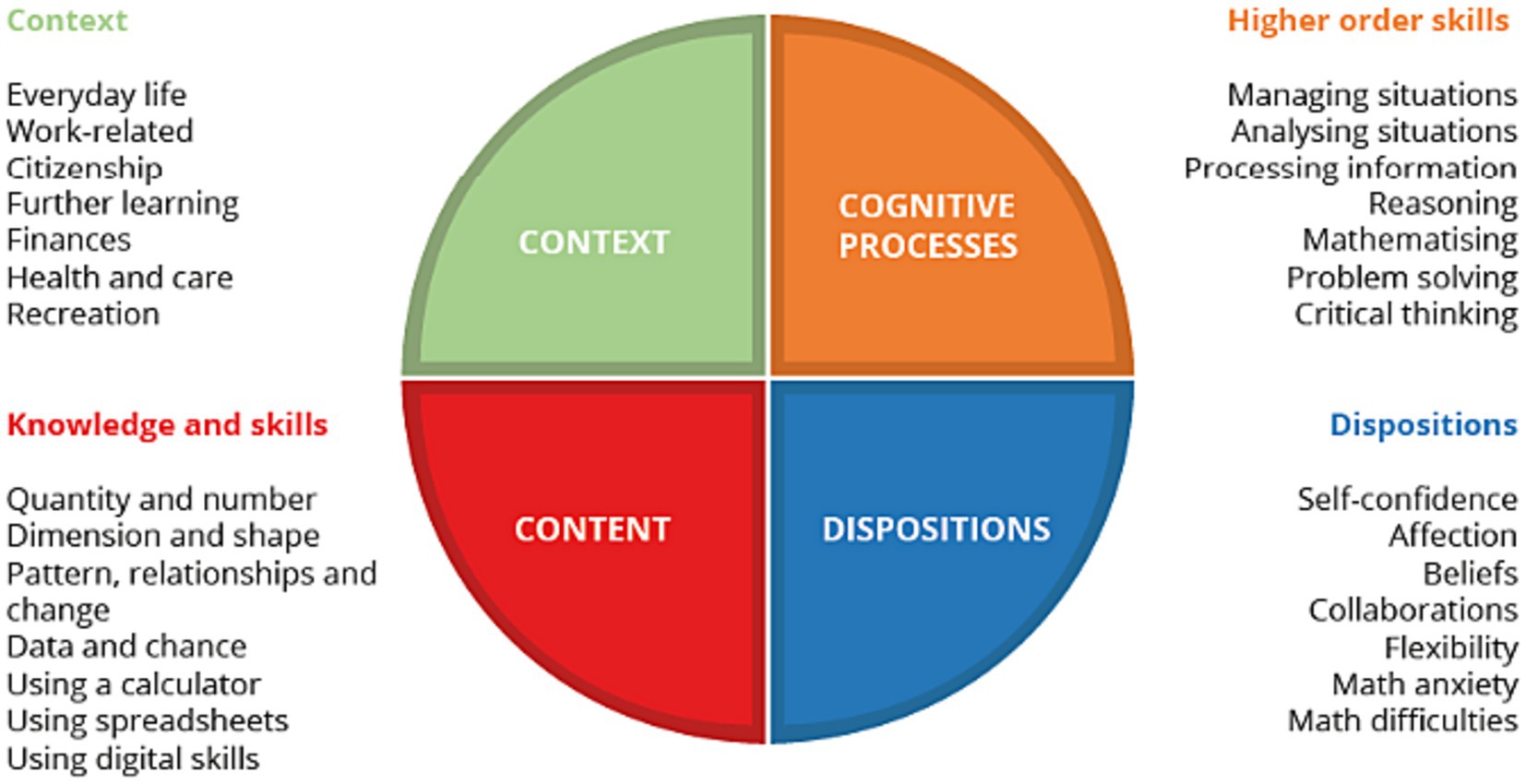
Figure 2. Common European Numeracy Framework (CENF; Hoogland et al., 2021a).
Content knowledge and skills matter to improve numerate behavior. The first four subcategories—quantity and number, dimension and shape, pattern, relationship and change, data, and chance—can be found in most frameworks regarding mathematics or numeracy outside the formal school system. They can be considered the contemporary replacements of the Algebra-and-Geometry division, which dominated the 19th and 20th centuries. The use of (digital) tools and applications is another set of subcategories relevant today to cope with situations in our digitalized society.
Finally, another facet of the framework that we use here is dispositions (Hoogland and Díez-Palomar, 2022). Adult numeracy dispositions develop throughout life, starting from childhood and compulsory school. They depend on people’s capabilities, beliefs and feelings concerning mathematics. Still, they are also influenced by external positive and negative experiences: how and what mathematics was learned in schools and experiences in lived-in situations. Students who perform better in school mathematics may have good math and numeracy provisions: feeling confident with numbers, pleasure with numbers, and recognizing the usefulness of mathematics. Conversely, students with negative experiences may develop negative feelings for numbers, less self-confidence or even math anxiety. The disappointment when receiving low marks for mathematics may have an enormous influence on the numeracy capacities of the future adult.
Materials and methods
In this article, our main objective is to make numeracy visible. Why is numeracy important? Why is it important to run campaigns to draw attention to numeracy? What are the challenges for this? In what situations, behaviors, and contexts in our daily lives is numeracy present, and what challenges does this entail from the point of view of making it visible? What opportunities for future action can we find by making numeracy visible to everyone? Until now, most of the existing scientific literature on numeracy has always been dedicated either to defining the concept or to developing tools (frameworks) to be able to measure it in quantitative or qualitative studies, such as IALS, ALL, PIAAC, In Balance, CENF, and so forth. In this article, we do something that has only been partially done in the field of adult education or studies of a socio-cultural nature (studies of the “mathematics” present in the cultural practices of human groups): we want to identify the numeracy that appears in the different contexts of daily life and make it visible. As this statement is very general, to specify (and operationalize) the study, we talk about four areas: general, finances, health, and critical use and interpretation of media. The objective is to make numeracy visible in these four areas.
Research design
The research questions of the study reported here are: what are the challenges regarding numeracy awareness today? And what prospects for future initiatives can be discerned by rendering numeracy apparent to all? The study we report here was organized in two phases to answer this question.
A systematic review of scientific literature was carried out in the first phase. The objective of this phase was to find the recent contributions made so far on numeracy when trying to make it visible. To do this, we first review what numeracy means since this concept has been defined differently over time (Hoogland et al., 2019, 2021a). The study reported in this paper focuses on numeracy as a multifaceted construct defined within the Common European Numeracy Framework (CENF) and Numeracy in Practice (NiP) projects. To guide the systematic review of the scientific literature, we used the four thematic axes of numeracy defined in the NiP project: general, finances, health and critical use and interpretation of media.
Drawing on identifying the principal contributions within the current body of research on numeracy across the aforementioned four domains, we proceeded to the second phase of our study. In this subsequent phase, we embarked on a further examination, contrasting the findings extracted from our systematic literature review with the perspectives and insights of adults. To facilitate this undertaking, we conducted two case studies, one in Spain and the other in Slovenia, given that these two countries are members of the consortium of partners involved in the Numeracy in Practice (NiP) project, which serves as the overarching framework for our investigation. We used a “focus group” as a research instrument in both case studies.
The primary aim of these two-fold case studies was to contrast the conclusions from the studies scrutinized in our systematic literature review against the experiential knowledge imparted by participants in the focus groups held as part of the case studies. In this manner, we endeavored to incorporate the voices of adults into a dialog designed to delve deeper into the findings about numeracy awareness within the four thematic dimensions outlined in the NiP project. This methodological approach adheres to the communicative methodology articulated by Gómez et al. (2013), which is grounded in the ontological principle positing that a comprehensive understanding of reality is constructed by engaging all the actors involved. Details about the data collection tools and the subsequent analytical procedures are elaborated upon in the following sections.
Data collection instruments
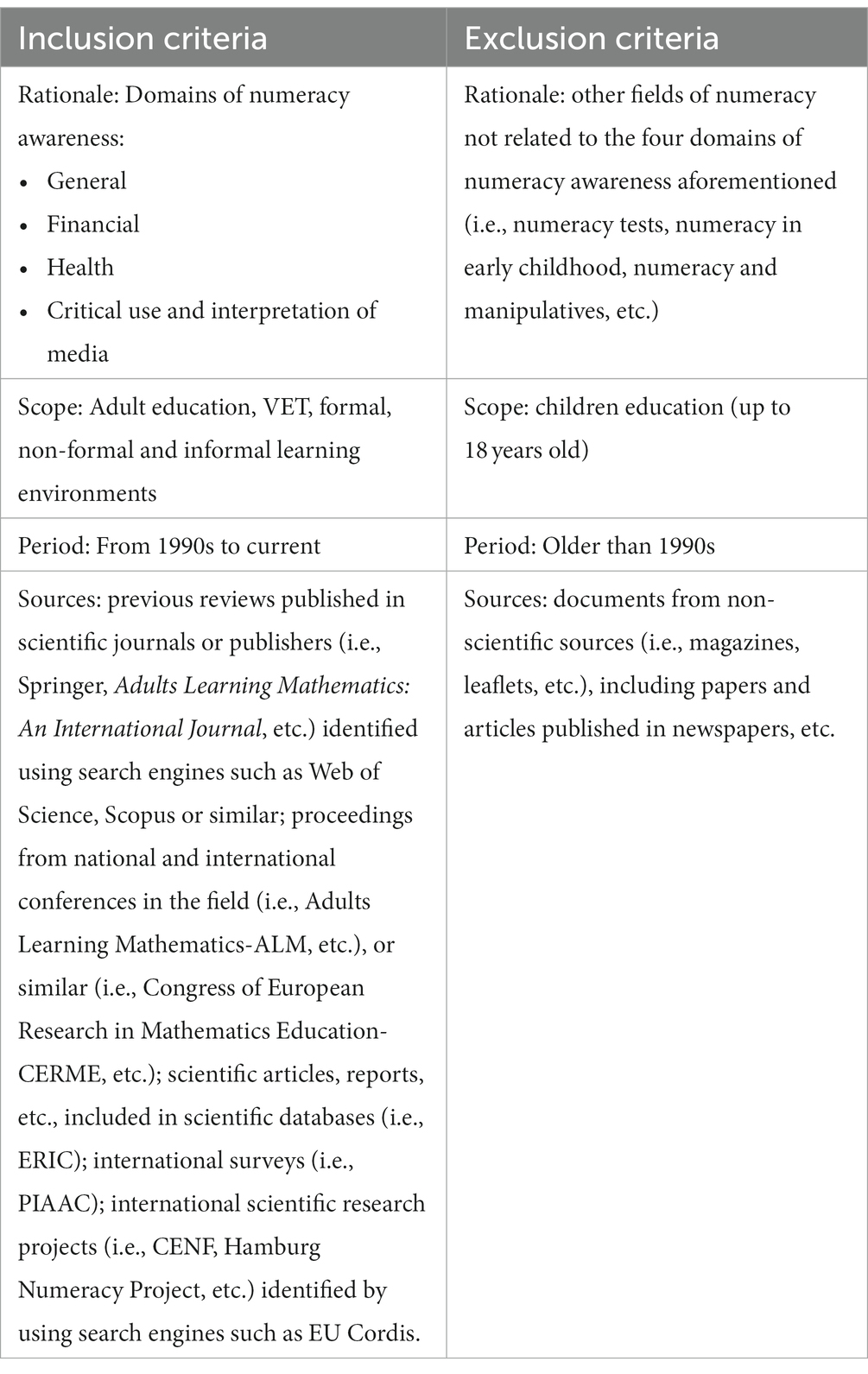
First, we conducted a systematic literature review. Following Egger et al. (2008), we defined the following inclusion and exclusion criteria (see Table 2).
We decided not to use the PRISMA method (Moher et al., 2010) to perform the literature review. The reason for our decision is that PRISMA is based on a targeted search with standard words and combinations of words (keywords) in databases without considering prior knowledge on the subject, so this method has the serious flaw of including irrelevant or little-known studies in the literature review. At the same time, it omits other studies that all of us who work in numeracy studies know are essential references but that the algorithm does not recognize (due to, for instance, the use of everyday expressions or non-normative ones in the title of the paper, or because the word “numeracy” does not appear in it, or similar reasons), and therefore are excluded from the search results.
For this reason, we have preferred to use an approach closer to the Delphi method (Brady, 2015), to carry out a targeted search drawing on the inclusion criteria displayed in Table 2, including authors and common sources in the field of numeracy study [i.e., international surveys, such as ALL, PIAAC; well-known research studies, such as CENF, Hamburg Numeracy Project, Numeric (All), MiA, In Balance; Handbooks and reference proceedings in the field, such as Coben et al., 2000, Safford-Ramus et al., 2016, among others]. At the same time, this search has been complemented with the use of search engines such as Google Scholar, the Web of Science search engine, Scopus, and the EU Cordis research projects search engine to complement the literature review as we are aware that in addition to expert knowledge on the subject, the use of these other sources is relevant to find, identify and include other less known studies that also respond to the objective of our study.
Secondly, we carried out two focus groups (one in Spain and the other in Slovenia). Focus groups are commonly used in educational research (Denzin and Ryan, 2007). Focus groups stand apart from other forms of group interviews by the researcher’s use of a predetermined set of directed inquiries tailored to evoke group perspectives on a particular subject. Participants in the focus groups (both in Spain and Slovenia) included: adult learners (with basic academic skills up to ISCED level 2) and teachers in adult education. The objective of these two focus groups conducted in Spain and Slovenia was to collect the voices of the participants (mathematics teachers in adult education, adult learners, and other stakeholders) to validate the contributions identified through the review of scientific literature. It is essential to say that a representative sample has yet to be made and that the results presented below cannot be generalized. The intention has been to identify relevant topics that future research would need to contrast with surveys carried out with representative samples. Our objective here has only been to identify items that could raise awareness and make numeracy visible in the four areas defined above: general, finances, health and critical use and interpretation of media.
The systematic literature review was employed to methodically consolidate the principal contributions of the international scientific community on our subject of inquiry. This review served the two-fold purpose of refining the scope of our investigation and identifying the key insights substantiated by prior research. Equipped with this structured foundation, we formulated an instrument tailored to probe the extent of these contributions within two distinct groups—adult learners and teachers of adult learners—drawn from Spain and Slovenia. These countries are members of the NiP project consortium, and our selection criterion focused on individuals engaged in adult education, with participants falling into the categories of adult education students (lacking higher education) and adult education teachers.
Our choice of employing focus groups was deliberate, given that the conversational dynamics among participants engender spontaneous discussions that often yield unanticipated, non-standardized ideas. This conversational spontaneity enriches the original question script of the instrument. Additionally, an instrument featuring a predefined set of questions to steer focus group conversations ensures reproducibility across diverse contexts, as was the case in Spain and Slovenia. This, in turn, facilitates the combination of data for comparative analysis. Nonetheless, it is important to acknowledge that, as detailed in the limitations section toward the conclusion of this paper, the focus group methodology is inherently circumscribed in its capacity for generalization, extending only to the participants within the two case studies. While focus groups permit in-depth exploration of the subject of study by affording interviewers the flexibility to pursue unscripted inquiries, these findings must subsequently be corroborated through studies employing representative samples, thereby allowing for broader applicability to the entire study population.
Data analysis instruments
To analyze the data collected, we have drawn on the Grounded Theory (GT; Strauss and Corbin, 1994; Glaser and Strauss, 2017). The GT is a research approach for the study design, data collection and analysis. According to the GT, the categories of analysis (the codes) emerge from the discourse analysis (Gee, 2014) of the data collected during the fieldwork. Thanks to the literature review, we had prior knowledge about the object of study (numeracy), which we had already defined and categorized elsewhere (CENF framework, Hoogland et al., 2021b). Building on that extensive work, we use the areas and facets defined in the CENF and NiP projects. There are four areas of awareness: general, finance, health, and critical use and interpretation of media. The facets are context, mathematical knowledge and skills, higher-order skills, and dispositions (see Figure 2). Both the domains and the facets have been combined during the analysis (that is, we looked for evidence corresponding to the four facets for each of the domains). On the other hand, as the objective of this study was to find challenges regarding numeracy awareness in these four areas, we also used the GT to maintain an open stance regarding new topics, items, or variables that we could not know a priori before to carry out fieldwork. The result has been organized in Table 3.
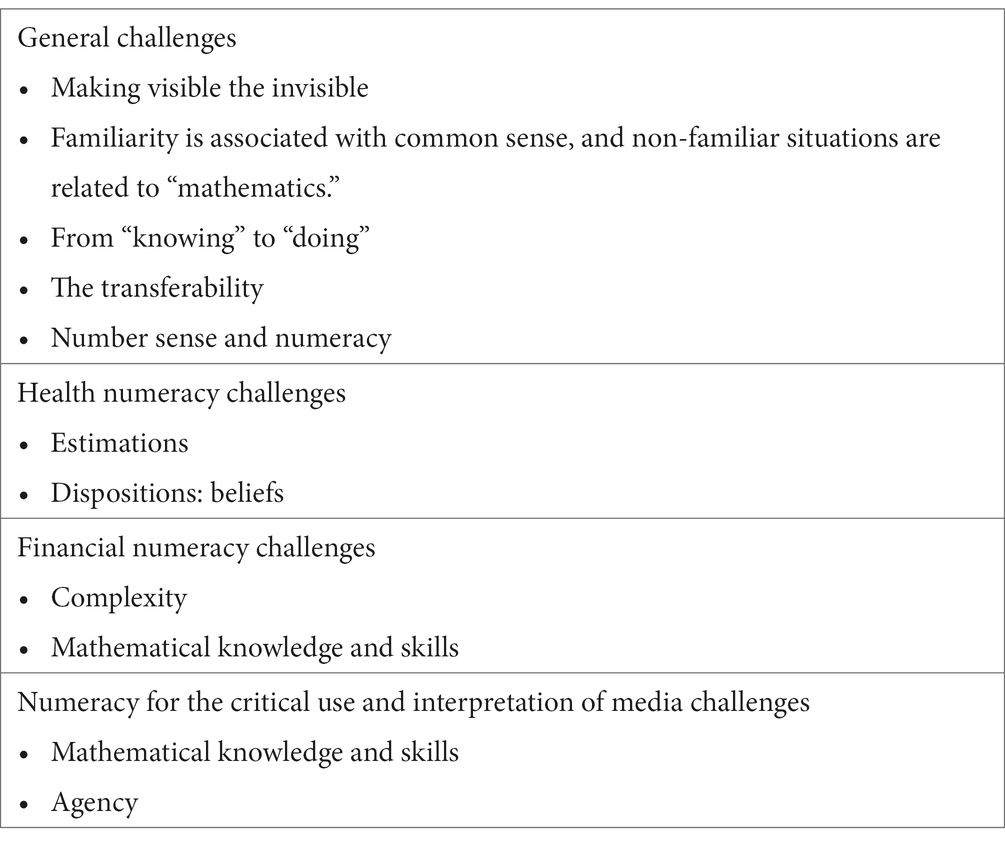
Table 3. Challenges related to numeracy awareness in the four dimensions raised in the NiP research project.
Results
In what follows, we present the results that have been obtained, both stemming from the revision of the scientific literature as from the contributions drawn from the two focus groups conducted in the two countries participating in this study. The findings are organized into topics to facilitate their presentation.
Challenges regarding numeracy awareness, in general terms, in the 21st century
The first challenge we have encountered is the difficulty in identifying numeracy aspects, contents, or behaviors from daily life that are currently considered components of numerate behavior (Hoogland et al., 2021b; Tout et al., 2021). In the framework from where we depart as a reference in this study (the Common European Numeracy Framework), numeracy is a multifaceted concept that refers to mathematical knowledge and skills (including quantity and number, dimension and shape, patterns, relationships and change, data and chance, using a calculator, using spreadsheets, and using digital skills), that are used in different contexts (everyday life, working place, citizenship, further learning, finances, health and care, and recreation), and that puts in place a series of higher order skills measured also by the dispositions of the person.
Numeracy appears when a person makes a sum and when solving a problem in everyday life. However, while performing an arithmetic operation is usually always identified as an example of numeracy, other actions, such as solving a problematic situation, are only sometimes associated with using either mathematical knowledge and skills or higher-order skills related to mathematical thinking. For example, when one decides to take an alternative route during rush hour to avoid traffic on the main road, this behavior is usually associated with common sense rather than with a rational calculation of the time it will take to reach the destination. Coben (2000a) relates several similar examples, which broadly coincide with what we have found two decades later, both in the case of the participants in the focus group in Slovenia and in the one we carried out in Spain. When asked about examples to identify numeracy, the most common one is usually either “doing the shopping,” “adapting a recipe for four people to a larger number of diners,” or “doing chores.” The following quote illustrates the type of comments made by people who participated in this study:
Some days ago, for example, I bought four things. It was not my initial idea to buy so much, so I had little money in my purse. But then I was counting, and when I went to pay, it was just what I had in my purse (Adult learner, Spanish focus group).
According to Coben (2000b), mathematics is associated with common sense when it is invisible. She illustrates it with the following quote:
You measure, put up shelves, distance, sire, and the brackets, where they go – that all involve general maths. To me, though, that’s just common sense. […] You do not think of [it] as being math (Coben, 2000b, p. 57).
Our data aligns with Coben’s assertion concerning the invisibility nature of numeracy. For instance, in the case study carried out in Slovenia, one of the participants noticed that:
Although we use numeracy quite a lot on a daily basis, we are rarely aware of it (Adult learner, Slovenian focus group).
This person acknowledges the ubiquity of numeracy within everyday life scenarios. Nevertheless, it is noteworthy that people need to maintain a conscious awareness of this omnipresence.
While numeracy practices are not visible, they are part of a numerical behavior, a way of acting and resolving the different situations that we encounter in daily life, in the workplace, when we exercise our citizenship, or in any of the other situations that Hoogland et al. (2021b) highlight as “contexts.”
However, when one asks about instances of employing numerical concepts in any of the contexts emphasized by Hoogland et al. (2021b), the notion of “common sense” appears to yield to a recognition that the activities in question are intricately connected to the domain of mathematics.
Unconsciously, all our lives, we are doing mathematics. Without us realizing. Even when we go to the supermarket.
Well, there’s more, is not it?
Yes, of course. How much does each thing you have in the trolley cost? To know more or less what you are going to pay (Adult learner, Spanish focus group).
As these mathematical aspects cease to remain concealed, they undergo a transformation into explicit mathematics within the discourse of the individuals involved in the two focus groups. The subsequent quotation from the Slovenian case study exemplifies this assertion:
Numeracy is associated closely to mathematics, so people think they are the same thing (Adult learner, Slovenian focus group).
It is of paramount importance to underscore that the participants in this study consciously avoid the use of the term “numeracy” in their discourse, primarily because neither the Spanish nor Slovenian languages possess a direct equivalent for this concept. When explicitly discussing their engagement with numerical concepts, they employ the term “mathematics.”
On the other hand, another critical aspect to highlight in the discourse of the people who participated in this study is that they seem to establish a distinction between “daily life mathematics” and “school mathematics.” The teachers who work in adult education centers who participated in the Spanish focus group say that the difficulties occur in the second case when we are talking about “school mathematics.”
The difficulties, where do you find them? In the fact that they do not know how to do subtraction, or that they do know how to subtract in their life, but they do not know how to do it in their notebook? In the notebook. They do not know how to do it in the notebook because, in the real world, no one tricks them. They know how to count. But of course, you do subtraction, and if you have to “carry,” they no longer know… the concept of… how do I explain it to them: units, tens, hundreds. There is no way. And here I find that I do not know how to do it. I look at little children’s books, but of course, it’s so evident here. If only I could have such a basic thing. Think that sometimes it’s because some people do not even know how to hold a pencil and have never been to school (Teacher, Spanish focus group).
The review of the scientific literature is consistent with this distinction (Civil, 2002). Díez-Palomar (2020) states that women who go to adult schools to study the basic level of education usually use different mental strategies to count and solve problems, sometimes totally different from “pencil and paper” school strategies. For example, the case of subtraction by inverse addition is a widespread mental strategy when shopping and differs entirely from subtraction “by positional value.” An older woman, for an entire semester, was trying to understand the meaning of “borrowing one” to apply the subtraction algorithm when the minuend unit is smaller than the subtrahend unit, and it is necessary to “borrow a unit from the tens” (for example, when you want to subtract 25 minus 19; to subtract nine from five, you have to ask a 10 from 20 to have 15 and then be able to subtract the 9). Nobody does that kind of subtraction when they go shopping. Thanks to the egalitarian dialog established in that case, that lady could overcome a negative disposition toward the place value algorithm of subtraction (since she had developed a negative self-confidence, saying that she was incapable of subtracting). Talking with the facilitator made it evident to her that she knew how to subtract because she “added” from 19 to 25 to obtain the result (the difference), and that result was correct. This example, consistent with the comments that came out in the focus groups of our study, shows another key finding: that there is a tension between “invisible” numerical behavior (those strategies that we use as if it were common sense, in Coben’s terms), and visible mathematics in school through algorithms.
Numeracy and health awareness
Health is another of the areas highlighted in the Erasmus+ project Numeracy in Practice that frames the study we are presenting in this paper. There is an exhaustive list of works that have been done on numeracy and health. Most fall within the health field as a “profession” (for example, nursing). Many studies on nursing and numeracy highlight the critical role of context. In a well-known ethnographic study on proportional reasoning, Hoyles et al. (2001) highlighted that nurses could use proportional calculation strategies in practices situated in context. Still, when the same knowledge was abstractly presented to them in very different situations far from their daily practice, they had difficulties coordinating their mathematical knowledge. Coben and Weeks (2014) also highlight the importance of using real contexts (as part of designing an environment for evaluating nurses’ numeracy). Eastwood et al. (2011) highlight the low numeracy competencies among qualified nurses and nursing students. The main errors they identify include conceptual, arithmetic, and computational ones. In their study, many nurses appeared to make errors in precision calculations (both conceptual and arithmetic). This analysis is consistent with other similar studies (Jukes and Gilchrist, 2006), and has motivated the design of professional training programs aimed at addressing these types of problems (Warburton and Kahn, 2007; O'Reilly et al., 2020).
From the point of view of health as a practice that people carry out in their lives, there is also abundant literature suggesting that many people have difficulties understanding (and following) the timing of the treatments prescribed by the doctor, the calculation of dosages, understanding laboratory values in individual reports, risks, and benefits of therapies, or getting (or not) a vaccine, etc. (Ancker and Kaufman, 2007). Golbeck and colleagues define health numeracy as “the degree to which individuals have the capacity to access, process, interpret, communicate, and act on numerical, quantitative, graphical, biostatistical, and probabilistic health information needed to make effective health decisions” (Golbeck et al., 2005, p. 375). Starting from this definition, most studies have analyzed aspects such as people’s ability to manipulate percentages and proportions, read and understand medication leaflets (Sanmamed et al., 2013), understand, organizing and communicate the schedules to take medicines, or the ability to use tables and graphs. While some studies talk about the competencies that people have in this type of practice, in others, the focus is on the effects of changing the numerical representation (for example, going from using a graph to a table of values).
In the case of the people who have participated in the case studies of this study, a clear example is that which refers to diets and the calculation of the number of calories and the amount of food to eat. Diet is a topic that is of interest to some of the people who participated in the study, and they use its numeracy to calculate the calorie content of foods, make comparisons, estimates, and make decisions about what to eat and what not to eat, based on the results of those calculations. In this case, one of the key issues that arises here is the estimation and precision of the calculations. Most of the time, the decision is based on an estimate (which, from a purely mathematical point of view, implies assuming tolerance margins rather than considering the precise calculation).
Another recurring example is calculating dosages; in this case, the task consists of taking half of a pill (which shape is a cercle). How exactly to cut a pill which is a cercle, in half? This situation is common and involves geometric thinking, estimation, and most of the time, the person ends up using a “visual” approach to break the pill “more or less” by what looks like “half.”
They call you from the hospital and tell you: look, I’m from the hospital and we’ll change your appointment. Sometimes you do not even know how to take the date they tell you… But you have made me think about medication: look, the other day we did this in the classroom: the teacher told us: imagine that you must take a pill, and you have it to split in two. You must take half of the pill; But how much is “half”? How much is a “quarter”? What if the pill is a round one? How do you make sure you cut “half” exactly, so you do not take more than the dose? (Adult learner, Spanish focus group).
Similarly, a teacher working with adult people in Slovenia stated that:
Interpreting data like dosage and frequency of medicine intake can be challenging for low-educated individuals. As a teacher of disadvantaged adults, I come across examples of needing help understanding these things when taking medicine (Teacher, Slovenian focus group).
Undoubtedly, innumeracy, or the possession of limited numeracy skills, exerts a profound and far-reaching influence on individuals’ health status and their capacity to oversee their own well-being or the well-being of those reliant upon them.
Being able to interpret numerical data can mean a difference between positive and negative health outcomes (Teacher, Slovenian focus group).
Several previous studies have confirmed the use of estimation as a preferred decision-making strategy (Curry et al., 1996). Adults prefer to make approximate (mental) calculations and adjust their actions to these calculations in most contexts (everyday life, recreation, citizenship, etc.). Ancker and Kaufman (2007) point to finance as one of the exceptions to this rule. According to them, “applying an estimation heuristic is generally quicker and simpler than calculating and is often sufficient for decision-making situations (e.g., choosing groceries while meeting a budget)” (Ancker and Kaufman, 2007, p. 715). It is also a behavior consistent with Coben’s (2000b) idea of the invisibility of mathematics.
In our case, in addition, another variable to take into account also appears: as a result of the pandemic situation and the debate about whether or not to get the COVID-19 vaccines, some of the people who participated in the focus group in Spain stated that their decision was motivated more by confidence (or not) in the safety of the vaccine, than by a precise calculation of the probabilities of contracting (or not) the disease, percentage of effectiveness of the vaccine, and percentage of cases in which the vaccine had produced adverse side effects. Using beliefs as criteria for making numerical decisions instead of numerical reasoning has been reported in many other cases (Plaza, 2021).
Numeracy and finances
According to the EU, only 45% of the European population understand simple and compound interest, and 20% understand the link between interest rates and bond prices; 35% need to understand what inflation is, and 34% do not understand that an investment with a higher return is likely to be riskier (Eurobarometer, 2023). In Spain, for example, people remember the case of the futures market. A decade ago, when the global financial crisis occurred due to the real estate market bubble, many people were recruited by banks to invest in the futures market, buying shares in products that did not exist, with the confidence that they would later become produce and investors were going to earn large amounts of money through interest. The financial market crashed, and those people lost their life savings. Something similar has happened (or is happening) with cryptocurrencies. How do we calculate financial risk? Financial literacy (financial numeracy) is a crucial aspect, and often, the mathematical algorithms embedded in finance are so complicated that not even the experts themselves can know for sure what is in those stock packages.
As Lusardi (2012) warns in the case of the USA, “In the last few decades, financial markets around the world have become increasingly accessible to the “small investor” as new products and financial services have come on the market “Nevertheless, many of these products are complex and difficult to grasp., especially for financially unsophisticated investors” (Lusardi, 2012, p. 2). This researcher analyses cases such as borrowing money (loans, mortgages, credit cards) and using financial services (payday loans, pawn shops, tax refund loans, rent-to-own shops, etc.), among other cases. In situations like calculating compound interest, only 18% of people who participated in the U.S. Health and Retirement study conducted in 2004 (Lusardi, 2012) were able to find the correct answer (the survey question was the following: “Let us say you have 200 dollars in a savings account. The account earns 10 percent interest per year. How much would you have in the account at the end of two years?,” Lusardi, 2012, p. 3).
The results of previous research are also consistent with what people participating in case studies say. Regarding the mathematical knowledge and skills facet of the CENF framework, people tend to have more difficulties with calculations involving percentages (quantity and number) or the calculation of probabilities (data and chance).
Different contexts also appear. The literature highlights the world of finance as the one that usually presents the most significant difficulties because it involves very complex and non-transparent algorithms, which are difficult to understand even for people with expert financial literacy. But the literature also mentions other contexts, such as domestic finances (calculating bills, understanding taxes on purchase receipts, calculation of tips, electricity bills, telephone bills, etc.). Among the people who participated in this study, the examples that are repeated the most are understanding the receipt of electricity, telephone, or payroll. Another example is understanding the purchase receipt:
… and we see the purchase receipt of Mercadona [grocery store], to see where the decimals are placed … (Adult learner, Spanish focus group).
There is also a person who talks about the retirement pension. These are examples that illustrate different contexts in which one (or several) of the mathematical knowledge and skills defined in the CENF framework appear.
On the other hand, another of the relevant findings that stand out from the contributions of the focus groups refers to higher-order skills—specifically, problem-solving. In the following quote, we see how an adult teacher states that for some adults, the difficulty is knowing what type of calculation to do when faced with a text problem (something similar to what happens to children, just as has been reported by previous problem-solving research—Pólya, 1957; Schoenfeld, 1992).
For example, the problems: addition problems… I bought a fish that cost me 3,50. Well, there are things that are difficult to understand. And look, some of them are readers, they swallow books. But understanding is difficult. The first thing they ask, like children, is: What do I have to do, add, or subtract? That’s your question (Teacher, Spanish focus group).
However, when the situation refers to numerical competencies in situated contexts familiar to adults, according to the mathematics teachers who have participated in the focus group, adults know perfectly how to do the calculations (although it is not the academic “paper and pencil” algorithm), so they know perfectly well what the correct result is. The following quote illustrates a prevalent case: people who have not attended school (and who, therefore, do not have academic mathematical literacy) know how to calculate perfectly (in a similar way to how a person who does not know how to read or write, knows perfectly how to speak, and communicate in his native language). This result suggests that when we talk about numeracy, we must include the idea of innate “number sense” that Baroody already studied (and characterized) in the case of children (Baroody, 2009; Baroody et al., 2014), and other authors have discussed in the case of adults (Barth et al., 2003; Galangco, 2023).
But those women tricked, no one tricks them. I had one who had a bar, and she did not know how to add or subtract or anything. And yet, they never cheated on her, when she charged for a coffee when she charged…because mentally [she knew how to calculate] … but later when it came to… [in school] … well, those are the elements they must live with (Adult learner, Spanish focus group).
Possessing a high level of financial literacy exerts a significant influence on individuals’ prospects. Additionally, numeracy also provides a “critical sense” that bears substantial consequence in the lives of individuals, enabling them to make enlightened and responsible choices. This “critical disposition” holds the capacity to shield individuals from potential risks and uncertainties, as expounded by Beck (1992). The subsequent quotation from the Slovenian case study serves as an illustrative embodiment of this revelation:
Financial literacy does not only give the individuals the ability to understand amounts, percentages, and risks. It also provides the basis for improvements in attitude toward finances and a more reasonable and responsible use of resources that benefits not only the individual but also the people and the environment around them (Adult learner, Slovenian focus group).
Numeracy and the critical use and interpretation of media
Finally, the last of the areas included in the study that is being discussed in this paper is the critical use of numerical information that appears in the media (mass media, but also social media). Much has been written on this topic, especially about using tables and statistics to represent, systematize or communicate ideas from quantitative data (Gal, 2002; Evans and Ruane, 2019). Paulos’s book on innumeracy and the cases that appear in it is already a classic on the misuse (or biased use) that is sometimes made of numbers in the media to influence and condition the general opinion of the public. Paulos (1988) relates typical cases, such as reading about voting trends or electoral results. Any electoral debate between candidates is another relevant example of the biased use of statistical data.
However, although there is extensive literature on the analysis of errors or biased information in the media and resources to reveal them and protect us against this type of misinformation (Maier, 2002; Evans et al., 2017), there are almost no studies that make a decalogue of numeracy situations in the media. The summary is almost reduced to using statistics and the different forms of data representation (Huff, 1993; Paulos, 2013). Evans and his colleagues report several sources to this effect.
For people who took part in the focus groups, the fact that media use mathematics content is obvious: they are very aware that mathematics appears in the media. The main challenges that are derived thereof include checking the source of the data, what they refer to, if the form of representation is correct (i.e., the use of the scale in the graphs, the intervals in which the data are grouped are equal), if the measures of central tendency or dispersion are used correctly, avoiding biases, etc.
All this has been thoroughly studied, and it is part of the do’s and don’ts of the critical reader of information in the media. The following quote from one of the adult learners in the Spanish case illustrates this “critical spirit” in the reading of information provided by the media:
… the evolution of the mortgages in the last years. Sometimes, graphs can provide us with biased information. They do not provide false information, but they represent it in a tendentious way so that the interpretation that we can do is not correct (Adult learner, Spanish focus group).
Diminished numeracy skills can likewise constrain individuals’ aptitude for interpreting data and comprehending the information disseminated by the media, consequently impeding their capacity to respond effectively and initiate appropriate actions. The subsequent quotation from the Slovenian case study serves as an exemplification of this phenomenon:
People need to pay more attention to numerical skills for critical interpretation of important information. For example, 1-degree rise in global temperature may seem like a small thing, but it is not. People can underestimate the importance of such change because they do not know what it means in the context of climate and not act to counter global warming decisively enough. Even verse, because of lack of critical interpretation skills, they can vote for policies that make global warming even worse (Adult learner, Slovenian focus group).
Now, one of the things that follows from the study that we report here is that although the people who have participated in the focus groups know that the media use numerical information and that they share the need to read the information of the media critically, it is also true that this way of acting implies an active attitude, an agency. From our point of view, playing agency in reading the information offered by the media is related to people’s disposition. Carrying out a passive reading of the media without paying attention to what is read can have the effect of being exposed to biased information, whether involuntarily or in a tendentious way. But neither in the review of the literature nor in the data from the focus groups have we managed to find more information on this topic.
Discussion
The objective of this paper is to find (and discuss) challenges regarding numeracy awareness in our daily lives where numeracy is pertinent (focusing on the challenges in health, finance, and critical use and interpretation of the media), exploring the obstacles to making numeracy conspicuous and the potential avenues for future action that can be unveiled by enhancing numeracy awareness across the board. Table 3 summarizes the main challenges related to numeracy awareness embedded in the different social contexts of our lives.
Both the contributions of the literature review, as well as the testimonies collected in the two focus groups that were carried out in Slovenia and Spain, suggest that numeracy is a competence to access, use and critically recognize the contents, information, and mathematical ideas that can be represented in multiple ways (Tout et al., 2021). As we have defined in the CENF framework, it is a multifaceted concept, which combines different aspects, not always visible, but embedded in different contexts, practices, situations, actions, objects, etc. Some researchers consider that it is a social practice (Yasukawa et al., 2018). Coben (2000a) had already defined being numerate as being “competent, confident, and comfortable with one’s judgments on whether to use mathematics in a particular situation and if so, what mathematics to use, how to do it, what degree of accuracy is appropriate, and what the answer means in relation to the context” (Coben, 2000a, p. 35). Similar definitions are found in Gal and Schuh (1994), Tout (1996), O'Rourke and O'Donoghue (1997), Tout and Marr (1998), van Groenestijn and Schmitt (2000), Kaye (2018), among others.
The fact that numeracy is often presented to us in an invisible way (implicit, embedded) represents a challenge for adult teachers who design the lessons, study programs and evaluation and assessment instruments (and for the authorities who design adult education standards). It is also a challenge for adults themselves since the “invisibility” of mathematics in certain situations is related to action dispositions such as “using intuition,” “beliefs,” and “emotional decisions” instead of using mathematical reasoning to solve problems and/or make decisions (like when I buy a lottery ticket because it coincides with my date of birth, without thinking that any ticket has the same probability of winning).
The objects that appear in the “mathematics knowledge and skills” facet in the CENF framework can be visible or invisible (embedded in the human practices of our societies). But in all cases, they are equally mathematical. Just because some practices are “common sense” does not mean they are any less mathematical. Bernstein's (2000) concept of horizontal discourse has sometimes been used to refer to numeracy.
Bernstein (2000) builds his theory based on the work of authors such as Bourdieu (1987b) (and specifically on concepts that appear in The Distinction). None of Bourdieu’s (1987a) concepts, nor his work, has ever served to improve education. There is no evidence that privileging and privileged relationships (a concept on which Bernstein builds his restricted and elaborate codes) serve to improve education. When Bernstein (2000) proposes that there are two discourses, the horizontal and the vertical, both regulated by rules of discourse that are structured in restricted and elaborate codes, what he is saying is that people who do not know how to use the elaborate code of the vertical discourse (academic mathematics) have a lower level than those who use the restricted code of the horizontal discourse. The evidence that we have collected in the fieldwork shows that the numeracy that adult learners use in their daily lives also presents elements of the “mathematics knowledge and skills” facet, as well as what we call “academic mathematics” (or “pencil and paper”).
It is important to highlight this aspect since, otherwise, it could be understood that the mathematics that we associate with numeracy is “less” complex, deep, rigorous, etc. (for a discussion on the naturalness of mathematics, we can refer to the work of Ernest, 2018). There is an explicit agreement among people dedicated to researching numeracy that the mathematics we include in this concept is not basic mathematics (simple arithmetic calculation algorithms; Tout et al., 2021). The challenge is how to make this mathematics visible in the classroom as well, as in the case of the example reported by Díez-Palomar (2020) of the adult woman who subtracted using the inverse addition algorithm.
Another general challenge that emerges from our work is that we must break with the idea that familiarity is associated with “common sense,” and when situations are unfamiliar, then the contents are perceived as “mathematics” (in the academic sense of the term). For example, consider the case of someone thinking that if twice as many people come to dinner, what they will have to do is calculate twice as much food so that everyone can have dinner is “common sense”; and then consider this other case of someone thinking that if the prospect of medicine gives the indications for the case of an 80 kg adult, but in his case, he have to administer that medicine to his son, who weighs 45 kg, and he has to apply a conversion rule that is not linear (but considering the volumetric difference). This is considered “doing math.” The challenge is to consider that we are talking about numerical awareness in both cases.
Gramsci (1971) already gave us elements in his philosophical reflections to dismantle this obstacle: for him, “common sense” is immediate knowledge, which serves to solve problems, and which is the result of a deep knowledge of all the variables present in the situation or problem to be solved. In our field of mathematics teaching, the Gramscian concept of common sense is very close to the concept of intuition of Poincaré (1908). Mathematical intuition cannot appear without a deep base of knowledge. It is a spontaneous form of knowledge but deeply rooted.
In the same way, common sense is the expression of profound knowledge. In other disciplines, such as Sociology, this type of knowledge has been widely studied. Schütz’s concept of the “lifeworld” is precisely the expression of everything that is part of tacit knowledge (which we take for granted), and that we use to make decisions in our daily lives (to solve problems, interpret and explain situations, in decision-making, etc.; Schütz and Luckmann, 1973).
Finding ways to recognize and make visible this numbered knowledge is one of the challenges we face in the immediate future in the field of ALM. It also has consequences in terms of transferability. Some authors have discussed the transferability of this type of knowledge, for example, in the field of vocational educational and training activities—VET—(FitzSimons, 2001; FitzSimons and Wedege, 2007; Black and Yasukawa, 2011; Keogh et al., 2018). For some authors, the conditions for transferring mathematical knowledge from school to the workplace or between different social contexts are unclear (Strässer, 1996; Evans, 1999; Wedege, 1999). However, what is clear from the literature review and from the testimonies of the people who have participated in this study is that numeracy is always contextualized. We cannot separate numeracy from the context in which it occurs. It is a multifaceted concept, as appears in the CENF framework (Hoogland et al., 2021b). The challenge that some people like Schliemann and Acioly (1989), Evans (1999), or Wedege (1999) noticed regarding the difficulty of transferring the content of the “mathematical knowledge and skills” facet to the “context” facet is still open. It is also part of the task to make numeracy more visible.
In this paper, we have organized the analysis in four areas (general, health, finances, and critical use and interpretation of media). However, it is relevant to indicate that numeracy is a social practice in all areas and contexts of our lives. Many of the challenges already discussed above are common to all four areas.
If we focus on health, the challenge is not to raise awareness about the “mathematical knowledge and skills” aspect. The objects, processes, and ways of representing them are part of the health practice. The challenges that arise today have more to do with the emergence of “expert systems” (computer programs that calculate dosages, Smartphone app algorithms that tell us what or how much to eat, automatic tables that tell us at what time we need to take a medicine, etc.) that “make decisions for us.” What happens when those expert systems break down, are unavailable, or give incorrect information because we have entered the data incorrectly because we do not understand what we are entering into the application or how the application uses that data? In this case, the challenge has to do with both mathematical knowledge and dispositions, and more specifically with the disposition to be critical, to think that we are the ones who understand mathematics, make decisions, and know how to interpret the data and use it correctly. It is a challenge that relates to both facets. Previous research has given numerous examples in professions such as nursing (Hutton et al., 2010) or in people’s health care (people are responsible for correctly administering doses of medicine to family members, for reading and interpreting the package inserts), to understand the brands and the different units of measurement on the cap of a medicine, or on the body of a syringe, etc. (Sanmamed et al., 2013).
In finance, the mathematical knowledge and skills facet is also related to dispositions, just as in health. In cases such as financial mathematics (bank products, investments, loans, rent, pensions, inflation, etc.), mathematics is evident (often in a very complex way). But as the testimonies of the people who participated in this study show, finances do not only appear in the professional context; We also see them in everyday life (at home, through bills, for example, when shopping, when calculating the tip at the restaurant, etc.). As we have seen, it happens that sometimes we prefer to make a decision based on a belief (disposition), not as a result of a rational calculation of different options (for example, I invest in shares of a company because everyone invests and I think I will be able to benefit; but if suddenly confidence is lost, the share price drops and I lose my money, as happened during the Crash of ‘29, for example). The fact that we rely on a series of beliefs without numerical basis to act (and that this leaves us in a situation of profound vulnerability) is also another of the challenges that we have been able to detect.
Finally, considering that several of the arguments we have used so far also apply to the field of critical use and interpretation of media, one of those that most clearly emerges is the case of agency. Many previous studies have drawn attention to the deceptions arising from using mathematics (statistics, data representations, etc.) in the media (news, advertisements, voting trend surveys, reports, etc.). People know this can happen (and it does), but sometimes, we do not pay attention (we see the data provided to us with a passive attitude). Therefore, one of the main challenges regarding numeracy awareness in this area is to find a way for everyone to have an active attitude (agency) to read the information about the world around us critically. Of course, this applies not only to cases in which media misinforms, but also when media use numbers to report truth, thus people also need to be numerate to interpret correctly. As one of the participants in this study stated in the focus group:
Critical thinking can be improved and supported by strong numeracy skills as it often Relies on estimates and predictions of trends that can be expressed through numbers, percentages, and other mathematical ways (Adult learner, Slovenian focus group).
Hence, the identification of prevailing numeracy awareness challenges may constitute a means to enhance individuals’ capacity for critical thinking and their preparedness to confront the exigencies of our contemporary society.
Limitations and outreach of the study
In the study we discuss here, we wanted to identify some of the challenges regarding numeracy awareness in the four areas defined in the Erasmus+ project Numeracy in Practice. We do not intend to generalize these challenges, nor these cases, to all adults. They have emerged from the analysis of previous studies (literature review) and from the testimonies of people who have participated in two case studies carried out through focus groups in Slovenia and Spain. The scope is, therefore, limited to the fact of identifying the challenges. Future studies must verify whether these challenges are specific or can be generalized to most “adults.” To do this, it will be necessary to conduct quantitative studies with representative samples.
Data availability statement
The raw data supporting the conclusions of this article will be made available by the authors, without undue reservation.
Author contributions
JD-P: Formal analysis, Investigation, Methodology, Writing – original draft, Writing – review & editing. MR-S: Formal analysis, Methodology, Writing – original draft. IM: Investigation, Writing – original draft. MS: Investigation, Writing – original draft. KH: Conceptualization, Funding acquisition, Investigation, Project administration, Supervision, Writing – review & editing.
Funding
The author(s) declare financial support was received for the research, authorship, and/or publication of this article. This paper has been produced with the support of the research project “Numeracy in Practice,” funded by the European Commission under the grant: 2021-1-NL01-KA220-ADU-000 026 292.
Conflict of interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
Publisher’s note
All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.
References
Ancker, J. S., and Kaufman, D. (2007). Rethinking health numeracy: a multidisciplinary literature review. J. Am. Med. Inform. Assoc. 14, 713–721. doi: 10.1197/jamia.M2464
Arney, C. (2002). Mathematics and democracy: the case for quantitative literacy. Math. Comput. Educ. 36:84.
Baroody, A. J. (2009). “Fostering early numeracy in preschool and kindergarten” in Encyclopedia of language and literacy development. Eds. R. E. Tremblay, M. Boivin, and R. de V. Peters. (London, ON: Canadian Language and Literacy Research Network), 1–10.
Baroody, A. J., Lai, M. L., and Mix, K. S. (2014). “The development of young children’s early number and operation sense and its implications for early childhood education” in Handbook of research on the education of young children. eds. B. Spodek and O. N. Saracho (London and New York: Routledge), 205–240.
Barth, H., Kanwisher, N., and Spelke, E. (2003). The construction of large number representations in adults. Cognition 86, 201–221. doi: 10.1016/S0010-0277(02)00178-6
Black, S., and Yasukawa, K. (2011). Working together: Integrated language, literacy and numeracy support in vocational education and training. Centre for Research in learning and change. Broadway, New South Wales: Faculty of Arts and Social Science, University of Technology Sydney.
Bourdieu, P. (1987b). Distinction: A social critique of the judgement of taste. Cambridge, MA: Harvard University Press.
Brady, S. R. (2015). Utilizing and adapting the Delphi method for use in qualitative research. Int. J. Qual. Methods 14:1609406915621381. doi: 10.1177/1609406915621381
Callingham, R., and Beswick, K. (2015). & Ferme, E. (2015). An initial exploration of teachers’ numeracy in the context of professional capital. ZDM 47, 549–560. doi: 10.1007/s11858-015-0666-7
Civil, M. (2002). Chapter 4: everyday mathematics, Mathematicians' mathematics, and school mathematics: can we bring them together? J Res Maths Educ 11, 40–62. doi: 10.2307/749964
Coben, D. (1992). What do we need to know? Issues in numeracy research. Adults Learn Maths 4, 15–16.
Coben, D. (2000a). “Numeracy, mathematics and adult learning” in Adult numeracy development: Theory, research, practice. ed. I. Gal (Cresskill, NJ: Hampton Press), 33–50.
Coben, D. (2000b). “Mathematics or common sense? Researching ‘invisible’ mathematics through adults’ mathematics life histories” in Perspectives on adults learning mathematics: Research and practice. eds. D. Coben, J. O'Donoghue, and G. E. Fitzsimons (New York, Boston, Dordrecht, London, Moscow: Kluwer Academic Publishers). 53–66.
Coben, D., Colwell, D., Macrae, S., Boaler, J., Brown, M., and Rhodes, V. (2003) Adult numeracy: Review of research and related literature. National Research and Development Centre for Adult Literacy and Numeracy, Kings College, London.
Coben, D., O’Donoghue, J., and Fitzsimons, G. E. (2000). Perspectives on adults learning mathematics: Research and practice (New York, Boston, Dordrecht, London, Moscow: Kluwer Academic Publishers).
Coben, D., and Weeks, K. (2014). Meeting the mathematical demands of the safety-critical workplace: medication dosage calculation problem-solving for nursing. Educ. Stud. Math. 86, 253–270. doi: 10.1007/s10649-014-9537-3
Curry, D., Schmitt, M. J., and Waldron, S. (1996). A framework for adult numeracy standards: The mathematical skills and abilities adults need to be equipped for the future. The Adult Numeracy Practitioners Network System Reform Planning Project.
de Lange, J., Bolletta, R., Close, S., Moreno, M. L., Niss, M., Park, K. M., et al. (1999). Measuring student knowledge and skills. A New Framework for Assessment. Paris: OECD.
Denzin, N. K., and Ryan, K. E. (2007). “Qualitative methodology (including focus groups)” in The SAGE handbook of social science methodology. eds. W. Outhwaite and S. P. Turner (Thousand Oaks, London, New Delhi: SAGE Publications Ltd), 578–594.
Díez-Palomar, J. (2020). Dialogic mathematics gatherings: encouraging the other women’s critical thinking on numeracy. ZDM 52, 473–487. doi: 10.1007/s11858-019-01092-2
Eastwood, K. J., Boyle, M. J., Williams, B., and Fairhall, R. (2011). Numeracy skills of nursing students. Nurse Educ. Today 31, 815–818. doi: 10.1016/j.nedt.2010.12.014
Egger, M., Smith, G. D., and Altman, D. G. (2008). Systematic reviews in health care: meta-analysis in context. 2nd Edn. London: BMJ Books.
Ernest, P. (2018). “The philosophy of mathematics education: an overview” in The philosophy of mathematics education today. ICME-13 monographs. ed. P. Ernest (New York, Heidelberg, Dordrecht, London: Springer).
Eurobarometer (2023). Monitoring the level of financial literacy in the EU. Available at: https://europa.eu/eurobarometer/surveys/detail/2953.
Evans, J. (1999). Building bridges: reflections on the problem of transfer of learning in mathematics. Educ. Stud. Math. 39:1058. doi: 10.1023/A:1003755611058
Evans, J., and Ruane, S. (2019). Data in society: Challenging statistics in an age of globalisation. Bristol, Chicago: Policy Press.
Evans, J., Yasukawa, K., Mallows, D., and Creese, B. (2017). Numeracy skills and the numerate environment: affordances and demands. Adults Learn Maths 12, 17–26.
FitzSimons, G. E. (2001). What counts as mathematics in adult and vocational education? Numeracy for empowerment and democracy? In L. Østergaard Johansen and T. Wedege (Eds.), Numeracy for empowerment and democracy? In: Proceedings of 8th international conference of adults learning Maths – A research forum (ALM) (pp. 21–32). ALM-A Research Forum.
FitzSimons, G. E., and Wedege, T. (2007). Developing numeracy in the workplace. Nordic Stud Maths Educ 12, 49–66.
Frankenstein, M. (1998). “Reading the world with Maths: goals for a critical mathematical literacy curriculum” in Proceedings of the first international mathematics education and society conference (180–189). ed. P. Gates (Centre for the Study of Mathematics Education: Nottingham University).
Gal, I. (2002). Adults' statistical literacy: meanings, components, responsibilities. Int. Stat. Rev. 70, 1–25. doi: 10.1111/j.1751-5823.2002.tb00336.x
Gal, I., Alatorre, S., Close, S., Evans, J., Johansen, L., Maguire, T., et al. (2009). PIAAC numeracy: A conceptual framework. In: OECD education working papers, no. 35. OECD Publishing.
Gal, I., and Schuh, A. (1994). Who counts in adult literacy programs? A National Survey of Numeracy Education. Philadelphia, PA: National Center on Adult Literacy Publications.
Gal, I., and Tout, D. (2014). Comparison of PIAAC and PISA frameworks for numeracy and mathematical literacy. Paris: OECD Publishing.
Galangco, J. (2023). Path model of mathematics achievement in senior high school. J. Res. Math. Educ. 12, 246–264. doi: 10.17583/redimat.12759
Gee, J. P. (2014). An introduction to discourse analysis: Theory and method. London and New York: Routledge.
Gellert, U., Jablonka, E., and Keitel, C. (2001). “Mathematical literacy and common sense in mathematics education” in Sociocultural research on mathematics education. eds. B. Atweh, H. Forgasz, and B. Nebres (New York and London: Routlege). 57–73.
Glaser, B., and Strauss, A. (2017). Discovery of grounded theory: Strategies for qualitative research. New York and London: Routledge.
Golbeck, A. L., Ahlers-Schmidt, C. R., Paschal, A. M., and Dismuke, S. E. (2005). A definition and operational framework for health numeracy. Am. J. Prev. Med. 29, 375–376. doi: 10.1016/j.amepre.2005.06.012
Gómez, A., Elboj, C., and Capllonch, M. (2013). Beyond action research: the communicative methodology of research. Int Rev Qual Res 6, 183–197. doi: 10.1525/irqr.2013.6.2
Gramsci, A. (1971). The modern prince. Selections from the prison notebooks. London: Foreign Language Press.
Hoogland, K., and Díez-Palomar, J. (2022). The Mathematisation of society: rethinking basic skills for adults. In: Twelfth congress of the European Society for Research in mathematics education (CERME12). Bolzano, Italy: European Project.
Hoogland, K., Díez-Palomar, J., and Maguire, T. (2018). Towards a second cycle of PIAAC. Programme for the international assessment of adult competencies. In B. Kelly, D. Dalby, G. Griffiths, D. Kaye and J. Stacey (Eds.), Boundaries and bridges: Adults learning mathematics in a fractured world. In: Proceedings of the 25th international conference of adults learning Maths – A research forum (ALM) (pp. 67). ALM-A Research Forum.
Hoogland, K., Díez-Palomar, J., and O’Meara, N. (2021a). Common European numeracy framework—A multifaceted perspective on numeracy. In: Paper presented at the 14th international congress on mathematics education (ICME14), Shanghai, China. Available at: https://www.researchgate.net/publication/368921986.
Hoogland, K., Díez-Palomar, J., Valls, R., O’Meara, N., O’Sullivan, K., Pirklbauer, T., et al. (2019). Common European numeracy framework. Literature overview and review EU Commission, European Project. Erasmus+.
Hoogland, K., and Stoker, M. (2021). The Mathematisation of society. Available at: https://www.youtube.com/watch?reload=9&v=8HisrNPI65E
Hoogland, K., van Groenestijn, M., Díez-Palomar, J., O’Meara, N., O’Sullivan, K., Stanic, M., et al. (2021b). Common European numeracy framework. Aspects and levels EU Commission, European project (funded by Erasmus + programe). Available at: https://cenf.eu/wp-content/uploads/2022/04/2021-11-30-CENF-Numeracy-Literature-Overview-and-Review.pdf.
Hoyles, C., Noss, R., and Pozzi, S. (2001). Proportional reasoning in nursing practice. J. Res. Math. Educ. 32, 4–27. doi: 10.2307/749619
Hutton, M., Coben, D., Hall, C., Rowe, D., Sabin, M., Weeks, K., et al. (2010). Numeracy for nursing, report of a pilot study to compare outcomes of two practical simulation tools–an online medication dosage assessment and practical assessment in the style of objective structured clinical examination. Nurse Educ. Today 30, 608–614. doi: 10.1016/j.nedt.2009.12.009
Jablonka, E. (2003). “Mathematical literacy” in Second International Handbook of Mathematics Education. eds. A. J. Bishop, M. A. Clements, C. Keitel, J. Kilpatrick, and F. K. S. Leung (Dordrecht: Springer), 75–102.
Jukes, L., and Gilchrist, M. (2006). Concerns about numeracy skills of nursing students. Nurse Educ. Pract. 6, 192–198. doi: 10.1016/j.nepr.2005.12.002
Kaye, D. (2003). Defining numeracy. In J. Maasz and W. Schloeglmann (Eds.), Adults learning mathematics–inside and outside the classroom. In: Proceedings of 10th international conference of adults learning Maths – A research forum (ALM) (pp. 194–199). ALM-A Research Forum.
Kaye, D. (2018). “Defining adult and numeracy: An academic and political investigation” in Contemporary Research in Adult and Lifelong Learning of Mathematics: International Perspectives, Eds. K. Safford-Ramus, J. Maaß, and E. Süss-Stepancik. 11–37.
Keogh, J. J., Maguire, T., and O’Donoghue, J. (2018). Adults, mathematics and work: From research into practice. Leiden, Boston: Brill.
Lusardi, A. (2012). Numeracy, financial literacy, and financial decision-making. Working Paper 17821. National Bureau of Economic Research. Cambridge, MA.
Maier, S. R. (2002). Numbers in the news: A mathematics audit of a daily newspaper. J. Stud. 3, 507–519. doi: 10.1080/1461670022000019191
Moher, D., Liberati, A., Tetzlaff, J., and Altman, D. G., Prisma Group (2010). Preferred reporting items for systematic reviews and meta-analyses: the PRISMA statement. Int. J. Surg. 8, 336–341. doi: 10.1016/j.ijsu.2010.02.007
O’Donoghue, J. (2003). Mathematics or numeracy: does it really matter. Adults Learn Maths Newslett 18, 1–8.
O'Reilly, R., Ramjan, L. M., Fatayer, M., Stunden, A., and Gregory, L. R. (2020). First year undergraduate nursing students’ perceptions of the effectiveness of blended learning approaches for nursing numeracy. Nurse Educ. Pract. 45:102800. doi: 10.1016/j.nepr.2020.102800
Organisation for Economic Co-operation and Development (OECD). (2021), The assessment frameworks for cycle 2 of the Programme for the international assessment of adult competencies, OECD skills studies, OECD Publishing, Paris.
O'Rourke, U., and O'Donoghue, J. (1997). Guidelines for the development of adult numeracy materials. In D. Coben, J. O’Donoghue (Eds.), Adults learning mathematics 4—proceedings of 4th international conference of adults learning Maths—A research forum (ALM) (pp. 173–191). ALM-A Research Forum.
Paulos, J. A. (1988). Innumeracy: Mathematical illiteracy and its consequences. London and New York: Viking Penguin.
Plaza, P. (2021). Las matemáticas en la educación de personas adultas. Video document, Available at: https://serveiseducatius.xtec.cat/cesire/recurs-eco2/las-matematicas-en-la-educacion-de-personas-adultas/.
Safford-Ramus, K., Keogh, J., O'Donoghue, J., and Maguire, T. (2018). Adults learning mathematics-A research forum 1993–2018. Celebrating 25 years: A lot done, a lot more yet to do. London: ALM-A research Forum.
Safford-Ramus, K., Misra, P. K., and Maguire, T. (2016). The troika of adult learners, lifelong learning, and mathematics. New York, Heidelberg, Drordrecht, London: Springer.
Sanmamed, F. F., Ruiz Eugenio, L., and Vrecer, N. (2013). La alfabetización en salud y el empoderamiento de las comunidades. Scripta Nova 17. Available at: https://ddd.uab.cat/pub/artpub/2013/218029/scrnov_a2013v17n427p5iSPA.pdf.
Schliemann, A. D., and Acioly, N. M. (1989). Mathematical knowledge developed at work: the contribution of practice versus the contribution of schooling. Cogn. Instr. 6, 185–221. doi: 10.1207/s1532690xci0603_1
Schoenfeld, A. (1992). “Learning to think mathematically: problem solving, metacognition, and sense making in mathematics” in Handbook of research on mathematics teaching and learning. Ed. D. A. Grouws (Reston, VA: National Council of Teachers of Mathematics), 334–370.
Schütz, A., and Luckmann, T. (1973). The structures of the life-world. Evanston: Northwestern University Press.
Sewell, B. (1981). Use of mathematics by adults in daily life. Leicester: Advisory Council for Adult Continuing Education (ACACE).
Strässer, R. (1996). Mathematics for work—a didactical perspective. (Regular Lecture at ICME 8, Sevilla).
Strauss, A., and Corbin, J. (1994). “Grounded theory methodology: an overview” in Handbook of qualitative research. eds. N. K. Denzin and Y. S. Lincoln (Thousand Oaks, London, Delhi: Sage), 273–285.
Tout, D. (1996). “Some reflections on adult numeracy” in Adults learning mathematics. Proceedings of 3rd international conference of adults learning Maths – A research forum (ALM). ed. D. Coben (Brighton, UK: ALM-A Research Forum), 13–16.
Tout, D., Coben, D., Geiger, V., Ginsburg, L., Hoogland, K., Maguire, T., et al. (2017). Review of the PIAAC numeracy assessment framework. Melbourne: ACER.
Tout, D., Demonty, I., Díez-Palomar, J., Geiger, V., Hoogland, K., and Maguire, T. (2021). “PIAAC cycle 2 assessment framework: numeracy” in The assessment frameworks for cycle 2 of the Programme for the international assessment of adult competencies (Paris: OECD Skills Studies, OECD Publishing), 65–154. doi: 10.1787/4bc2342d-en
Tout, D., and Marr, B. (1998). A new curriculum. In D. Coben & M. van Groenestijn (Eds.), Mathematics as part of lifelong learning. In: Proceedings of 5th International Conference of Adults Learning Maths – A Research Forum (ALM) (pp. 145–157). ALM-A Research Forum
van Groenestijn, M., and Schmitt, M. J. (2000). Numeracy assessment for the international life skills survey. In D. Coben, and J. O’Donoghue (Eds.), Adults learning mathematics 4. In: Proceedings of 4th International Conference of Adults Learning Maths – A Research Forum (ALM) (pp. 116–122). ALM-A Research Forum.
Warburton, P., and Kahn, P. (2007). Improving the numeracy skills of nurse prescribers. Nurs. Stand. 21, 40–43. doi: 10.7748/ns.21.28.40.s55
Wedege, T. (1999). To know or not to know – mathematics, that is a question of context. Educ. Stud. Math. 39, 205–227. doi: 10.1023/A:1003871930181
Withnall, A. (1994). Literacy on the agenda: the origins of the adult literacy campaign in the United Kingdom. Stud Educ Adults 26, 67–85. doi: 10.1080/02660830.1994.11730598
Keywords: numeracy, adults learning mathematics, Common European Numeracy Framework, mathematics education, mathematics knowledge and skills
Citation: Díez-Palomar J, Ramis-Salas M, Močnik I, Simonič M and Hoogland K (2023) Challenges for numeracy awareness in the 21st century: making visible the invisible. Front. Educ. 8:1295781. doi: 10.3389/feduc.2023.1295781
Edited by:
Merrilyn Goos, University of the Sunshine Coast, AustraliaReviewed by:
Heidi Biseth, University of South-Eastern Norway (USN), NorwayLena Lindenskov, Aarhus University, Denmark
Copyright © 2023 Díez-Palomar, Ramis-Salas, Močnik, Simonič and Hoogland. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Javier Díez-Palomar, amRpZXpwYWxvbWFyQHViLmVkdQ==
 Javier Díez-Palomar
Javier Díez-Palomar Mimar Ramis-Salas1
Mimar Ramis-Salas1 Kees Hoogland
Kees Hoogland