Developing a visual model to represent the implementation of an ambitious mathematics program

We describe the development of a visual model to represent the implementation of an ambitious mathematics program, which serves as an example of a complex educational reform. Visual models can be both conceptual and empirical, representing aspirational and theoretical perspectives while simultaneously incorporating empirical details specific to the context. Integrating conceptual and empirical aspects leads to tensions in managing the complexity of the model. Our process began with a simple model that guided our empirical work, which involved qualitative analysis. As we explored the systems and resources associated with the implementation of the ambitious mathematics program, the model took on more detail and complexity, both conceptually and empirically. In subsequent iterations of the model, we encountered tensions in balancing conceptual and empirical purposes, as well as challenges in displaying its emerging complexity.

Introduction

Representing complex phenomena using visual models offers benefits and poses challenges. Visual models, including those emerging from qualitative data analyses, potentially generate new insights into phenomena by emphasizing connections and relationships in ways that are difficult to show using text alone (Radnofsky, 1996). Radnofsky describes how “visual representations facilitate an understanding of complex and conflicting views” (p. 385), adding that models are a “set of visual signifiers intended to represent data analyses that are usually communicated in narrative form” (p. 386). Henderson and Segal (2013) explained that “visual representations of qualitative data can reduce and focus text, providing a structure to identify patterns and outliers, or introduce new levels of understanding” (p. 55). In short, visual models can reveal relationships and patterns that might remain hidden in narrative form, offering valuable perspectives.

Despite these affordances, there are challenges when using visual models to represent complex phenomena. First, they have an inferential nature, and thus do not necessarily mimic an objective reality. This leads to questions about the balance between inference and objective detail. Furthermore, there is not a singular or direct approach to developing a visual model (Radnofsky, 1996). Instead, models are developed iteratively, with a reflexive relationship between conceptual perspectives and empirical analyses. Radnofsky states:

Indeed, models are not so much made as they are developed, undergoing various stages-and part of the process must perforce be empirical; one can hardly construct a highly complex model (especially from multiple data sources) purely as a mental image, and then flawlessly put it down on paper or draw it on a computer screen. In this process, then, the researcher is forced to question assumptions, relationships, and connections. (p. 393)

Radnofsky compares the process of model development to the constant comparison process described by Strauss (1987), in which theory and empirical analysis are iteratively put into contact with each other to explain a phenomenon. In addition to the challenges of attending to both theory and empirical data when developing a model, there is the tension between parsimony and complexity. Henderson and Segal (2013) state that a “challenge is adding structure to the data without oversimplifying or misrepresenting them and without losing the subtle meanings or emotions rooted in them” (p. 56). Thus, in the process of iteratively developing a model, a tension arises related to representing the emergent complexity of the phenomenon.

The problem we addressed in this study was how to visually represent a complex educational phenomenon, which in this case was the implementation of an ambitious mathematics program based in a high need school. We began with a relatively simple model that was well established in the literature, that of the instructional triangle (Ball and Forzani, 2009; Cohen and Ball, 1999; Cohen et al., 2003). Using that model as a guide, we collected and analyzed data from the research site. We then used our empirical findings and additional conceptual development around ambitious mathematics teaching to iteratively develop the visual model. As we did so, we encountered tensions related to balancing conceptual and empirical aspects and maintaining parsimony while adding important detail. The statement of the educational problem addressed in this study is: how can we visually represent the implementation of an ambitious mathematics program while managing the tensions between the conceptual / empirical and between complexity / parsimony? In this article, we focus primarily on visually representing the phenomena that occurred inside of mathematics classrooms rather than the broader school and community context.

This article has three purposes. First, we hope to contribute to the field’s understanding of ambitious mathematics teaching and conditions and resources that support its implementation. We hope our representation can be used to spark productive conversation for educators who wish to implement ambitious mathematics teaching. Second, we hope our study is an illustrative case of the tensions that arise when building a visual model of an ambitious educational reform. Third, we hope to contribute to efforts to build visual models emerging from qualitative data analysis.

Below, we describe the study site and our conceptions of ambitious mathematics teaching to provide context for the development of the model. We then describe earlier versions of the instructional triangle before explaining our processes for generating iterations of a visual model.

Study site

We situated our study in a high-need secondary school in New York State. The John Lewis School (a pseudonym) served 1,061 students in grades 6–12. The school was divided into a Lower School (grades 6 to 8) and an Upper School (grades 10–12), with a 9th grade academy bridging the two. The city where the school is located is considered an urban-emergent area (Milner, 2012) because its population is under one million. Though cities of this size have less intensive problems than larger cities, they still face problems such as scarcity of resources and concentrated poverty. 94.5% of students were classified as economically disadvantaged, 54.8% as Black or African American, and 34.7% as Hispanic or Latino. In addition, 16% of students were labeled as Students with Disabilities, and 13% of students were labeled as English Language Learners. In 2014, New York State threatened to close the John Lewis School because of chronically poor performance on a range of metrics; so, starting in the 2015–16 school year, the University of Landover (a pseudonym) partnered with the Fullerton City School District (a pseudonym) and the Fullerton School Board (a pseudonym) to form an Educational Partnership Organization (EPO). The EPO was a partnership between the school district, the community, and the university that garnered considerable local and national attention for the scale of effort, quality of reforms, and sustainability (Larson and Nelms, 2021). In the first few years of implementation, the EPO showed improvement in several areas, including increases in student achievement on high-stakes tests, enrollment in advanced mathematics coursework, and graduation rates in addition to decreases in suspension rates. The EPO effort included a sustained implementation of an ambitious mathematics program, which we studied beginning in spring 2020. Below, we articulate our conception of ambitious mathematics teaching to ground the subsequent discussion and then describe the development of the visual model.

Conception of ambitious mathematics teaching

Ambitious mathematics teaching is a constellation of practices whose purpose is to engage students in mathematical activities that involve core mathematical ideas and that incorporate participation structures and pedagogy that position students as important and competent intellectual contributors (Choppin et al., 2024; Lampert et al., 2010; Singer-Gabella et al., 2016). Mathematics educators describe ambitious mathematics teaching as instruction that actively engages students in mathematical sensemaking in ways that broaden participation in mathematical discourses (Boaler and Staples, 2008) and position students as mathematically competent (Kelley-Peterson, 2010). Initial formulations of ambitious teaching emphasized disciplinary practices, such as solving complex problems, reasoning about mathematics (Bieda et al., 2020), and listening for and responding to others’ intellectual contributions (Lampert et al., 2010; Jacobs et al., 2010; van Es and Sherin, 2008). Recent formulations of ambitious mathematics teaching call for a focus on equity, including the importance of aligning instruction with students’ social, linguistic, and cultural resources (cf. NCTM Research Committee, 2018).

We identified four key components of ambitious mathematics teaching that informed the development of the model: eliciting and responding to student thinking; developing student autonomy and recognizing competence; using complex, authentic, high-demand tasks; and emphasizing multiple dimensions of equity. We briefly summarize these four elements below.

Eliciting and responding to student thinking

In ambitious mathematics, teachers are expected to elicit student explanations to understand student reasoning and to make that thinking public (Boston, 2012; Franke et al., 2007). Anthony et al. (2015) state that eliciting student reasoning helps the teacher to know:

What the student is thinking about and what is important about it; how the student is interacting with a task; what interests and motivates a student; how the student’s understanding is developing; what makes the ideas difficult and what does that student already know that might offer a bridge, and so on. (p. 7-8)

In addition to helping the teacher develop an understanding of student thinking, moves that probe student reasoning “often sustain teacher-student discourse” and “provide other students the opportunity to hear peers’ reasoning or justification” (Boston and Candela, 2018, p. 431).

Developing student autonomy and recognizing competence

An important outcome of eliciting and responding to student thinking is the development of student autonomy and positioning students as mathematical authorities. The focus on student autonomy rests on the assumption that students possess mathematical competencies on which to develop key disciplinary content; thus, AMT coincides with asset-based perspectives (e.g., NCTM Research Committee, 2018). An asset-based approach “is grounded in the belief that students’, families’, and communities’ ways of knowing, including their language and culture, serve as intellectual resources and contribute greatly to the teaching and learning of high-quality mathematics” (NCTM Research Committee, 2018, p. 375). An implication of assuming student competence is that students’ struggles are seen through the lens of opportunities to learn rather than seen as stemming from deficits in students (Horn, 2012).

Using complex, authentic, high-demand tasks

Eliciting and building from student thinking requires the presence of mathematical tasks that incorporate “big ideas” that allow for connections between sets of mathematical experiences; focusing on important, broad ideas in mathematics provides opportunities for students to invent strategies and approaches that emerge from their intuitive and everyday ways of thinking and acting in the world (cf. Gravemeijer and Doorman, 1999; Moschkovich and Brenner, 2002).

Emphasizing multiple dimensions of equity

Ambitious mathematics teaching has increasingly been described in terms of equitable opportunities for students to learn mathematics. Broadly speaking, the focus on equity has positioned teaching practices in terms of culturally responsive instruction. We interpret this to mean attending to the lived experiences of students, incorporating multiple modes of participation, and recognizing and building from students’ social, linguistic, and cultural resources (cf. Moschkovich, 1999).

Our starting point: the instructional triangle

Researchers have drawn on the instructional triangle to explain essential aspects of practice in mathematics classrooms. Cohen and Ball (1999) proposed a set of relationships between students, teachers, and content. They theorized how teacher characteristics (e.g., content knowledge, pedagogical content knowledge, ability to notice student thinking) mediate their use of materials, their interactions with students, and their efforts to build from student thinking. Their model further describes how student characteristics impact interactions between teachers and students as well as between content and students; in addition, students are resources for other students’ learning. In the model, instructional materials mediate student engagement with disciplinary ideas and thus their learning.

Cohen et al. (2003) revised the model to place greater emphasis on the role of student–student interactions, aligning with perspectives of the role of interaction and discourse in the learning of mathematics [e.g., the social turn in perspectives on the learning of mathematics (cf. Lerman, 2001)]. Additionally, there is a greater emphasis on the role of the environment, components that reflect situated and sociocultural perspectives (e.g., Brown et al., 1989; Wertsch, 1991) that influenced thinking in mathematics education research. In this version of the model, teachers deploy various forms of knowledge to make content accessible to the students; furthermore, students learn by interacting with the teachers and classmates. These interactions are mediated by influences from the broader environment.

A further revision of the model proposed by Ball and Forzani (2009) departs from the first two models by explicitly focusing on how teachers take up ambitious instructional practices, which requires “a flexible repertoire of high-leverage strategies and techniques that can be deployed with good judgment depending on the specific situation and context” (p. 503, authors’ italics). Ball and Forzani explained how productively utilizing these practices requires synchrony with various stakeholders and layers in the instructional ecosystem and with educational policies that impact that school. This model provides further nuance and complexity of teacher practices and the role of context, consistent with the increased complexity associated with ambitious forms of mathematics teaching and increasing attention to broader systems in which classrooms exist.

These models provide increasingly complex depictions of the instructional situation in classrooms by incorporating perspectives oriented toward social, discursive, and situated views of learning, as well as an increased focus on the systems that impact classrooms. Furthermore, recent extensions of the instructional triangle have proposed distinct roles for a range of artifacts and other influences (Rezat and Sträßer, 2012; Ruthven, 2012) as additional mediating presences, expanding the triangle to a socio-didactical tetrahedron or didactical tetrahedron, respectively. These broadening conceptualizations of the instructional triangle point to a movement toward a systems-level perspective to study instructional practices.

Version one: revising the instructional triangle to incorporate systemic perspectives

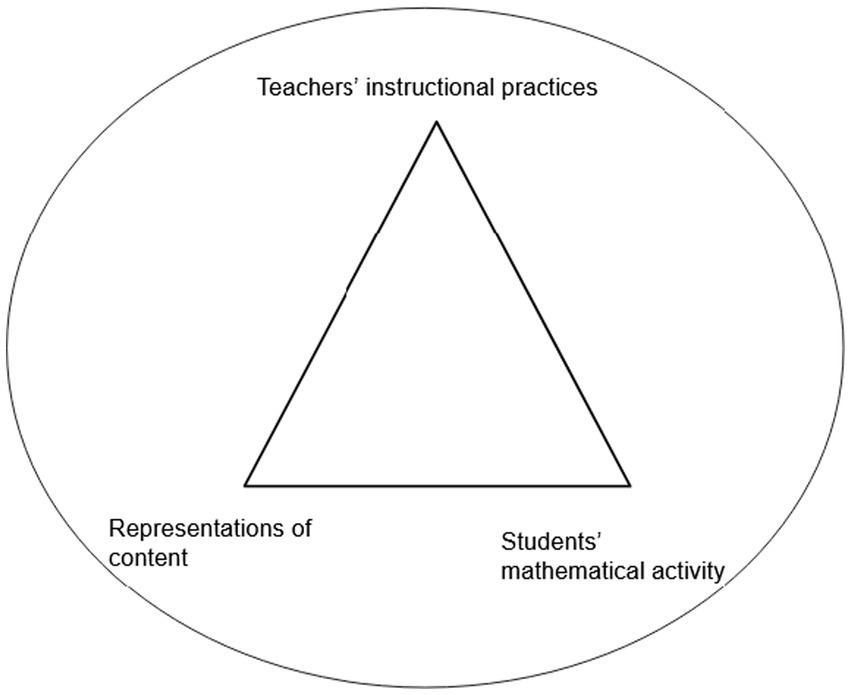
To create our first version of a visual model, we reconceptualized the nodes of the instructional triangle using an activity system perspective. Doing so positions cognition as being collective and distributed (cf. Engeström, 2001; Lankshear and Knobel, 2006) rather than belonging to individuals. Below, we present our initial representation and explain how we reconceptualized each node, see Figure 1.

Figure 1

Figure 1. Version one: the instructional triangle from an activity system perspective.

We describe our conceptualizations of the nodes, beginning with the teacher node, then the student node, and then the content node. We relabeled these nodes as teacher’s instructional practices, students’ mathematical activity, and representations of content, respectively. The circle around the triangle signifies that we constrain ourselves to classroom contexts.

Node 1: Instructional practices

The models of the instructional triangle discussed in the literature focused on the characteristics and actions of individual teachers. In those models, and ours, teachers mediate how students engage with content through their instructional practices. However, our model emphasizes practices rather than the traits of individual teachers as important factors to consider when characterizing classroom practice; doing so allows us to consider the goals of the practices and the systems that support them. Our model explicitly focuses on how teachers’ practices reflect the goals, purposes, and tools of the broader system.

Node 2: Students’ mathematical activity

We start with a similar distinction in this node in that the original or previous iterations of instructional triangle models focus on the characteristics, actions, and cognition of individual students. While there are mediating factors between students and content in the prior models—including the teacher, the environment, and other students—the focus foregrounds what happens inside the heads of individual students in contrast to a view that emphasizes the activities and practices in which the students engage. We define mathematical activity as processes by which students engage with mathematics, including following established processes, recalling terminology, demonstrating flexible use of tools, applying mathematics to contexts, and generating or demonstrating conceptual understanding (Choppin, 2025). This revised conceptualization emphasizes activity and its collective and distributed nature (e.g., Engeström, 2001).

Node 3: Representations of content

Our third distinction focuses on specific representations of content rather than abstracted notions of content. We posit that student engagement is mediated by specific representations of content and tasks in curriculum materials. Knowledge of the concept of linearity, for example, can be said to exist primarily in the contexts in which students encounter it rather than in the abstract, though we recognize that multiple encounters in different contexts may lead to a more abstracted understanding (Vygotsky, 1986). Our model thus relies on situated views of learning in which cognition and actions are strongly linked to the contexts in which they are developed (Brown et al., 1989; Roth and Jornet, 2013). Focusing on representations of content allows us to consider the systems and perspectives that produced those representations.

Process of iterating from version one to version two

We used the model in Figure 1 to inform our data collection and analysis. Our data analysis in turn led to a revised version of the model, Version Two (see Figure 2). This revised version of the model in turn informed our subsequent efforts to revise the model further. Our goal for Version Two was to develop a model that maintained the conceptual underpinnings of Version One while incorporating aspects of the empirical phenomena we observed at the research site. Tensions between the aspirational and empirical foundations of the model were present throughout the revision process. To reinforce the empirical foundations of the model, we used the nodes from Figure 1 to generate the following questions:

1. What instructional practices were emphasized in the mathematics program?

2. What kinds of mathematical activities were emphasized in the mathematics program?

3. What were the characteristics of the curriculum materials?

a. What kinds of activities were emphasized in the materials?

b. What views of mathematics were evident in the materials?

Figure 2

Figure 2. Version two: revision based on analysis of interview data and definition of AMT.

These questions guided our data analysis, which identified essential elements of classroom activity. Simultaneously, we chose to incorporate conceptual elements from the dimensions of AMT, as described in our earlier conceptualization of AMT.

Data sources and analysis that contributed to revisions of the model

We conducted 45 interviews with four teacher leaders, eight other teachers, four administrators, and six external consultations who supported the mathematics program. The interviews involved protocols focused on retrospective accounts of the development of the mathematics program, teachers’ perceptions of the mathematics program, organizational practices pertaining to the mathematics program, and the impact of COVID and the return from COVID. The interviews were conducted between fall of 2020 and spring of 2022.

The research team analyzed the interview data using inductive qualitative approaches described by Saldaña (2013). The lead researcher divided the transcripts into over 900 passages whose lengths varied from 50 to 250 words, and then placed each passage into categories based on topics discussed in the passages (e.g., identification of curriculum programs; instructional philosophy; implementation; resources). Passages were focused on a topic or line of questioning. When the speakers moved to a new topic or line of questioning, we created a passage. We then reduced the data using an inductive process. Three researchers created one or more memos for each passage. For each passage, the researchers then reconciled these memos into a collective memo. For each category, a researcher sorted the collective memos into supermemos, each of which had between 10 and 30 memos associated with it. The memos and supermemos were intended to be low-inference and parsimonious paraphrases of the original passages. The 78 supermemos were then grouped into 24 themes that represented overviews of the findings from the interviews.

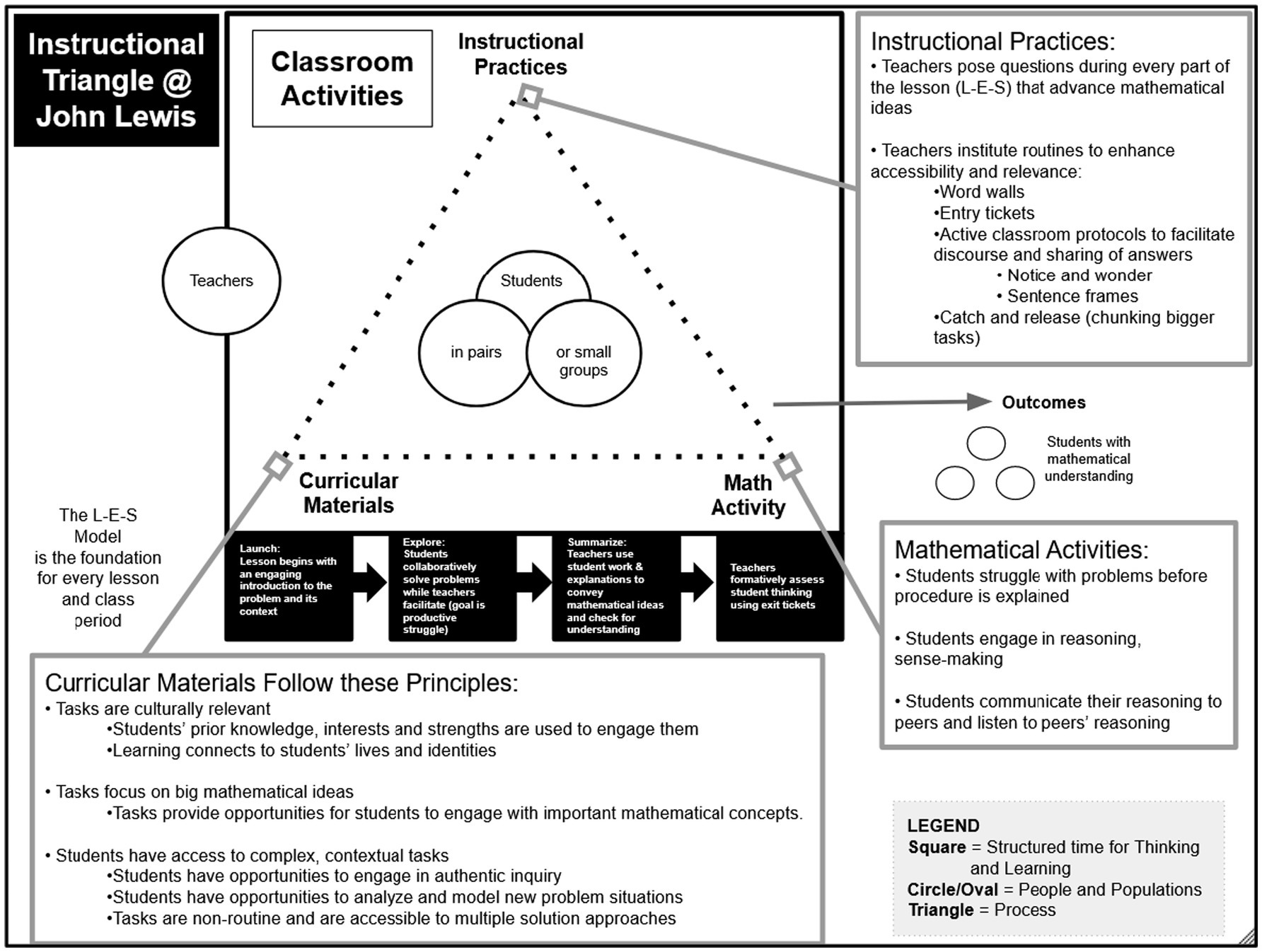
The supermemos and themes helped the research team locate data specific to a node (e.g., instructional practices) in order to generate details for each node. For example, to identify instructional practices, we looked at the following supermemos: using routines in inquiry-based lessons, following the Launch-Explore-Summarize structure, using interaction protocols to promote interaction, and modifying tasks and problems to make them more accessible for all students. We found over 70 quotes that referenced the Launch-Explore-Summarize (LES) sequence. The sheer quantity of the quotes was reinforced by the importance of the LES structure emphasized in the quotes, so we decided to include it in the revised model. Similarly, the analysis identified participation structures (e.g., independent work time, group work, whole class discussions) as central to the vision of instruction, so we added participation structures to the model. We identified other instructional practices as well, as seen in Figure 2. A similar emphasis was noted for modifying tasks and problems to make them more culturally relevant, which we added to the model. An example of revising a task to emphasize cultural relevance came from Owens (all participant names are pseudonyms), a teacher leader at the Upper School, who explained:

Instead of a paragraph explaining what a general store was in the Old West as you were going out to California, it was, “Here’s a black-owned business in Fullerton that sells all different kinds a cereal,” and changed the context of the problem, again, without changing the math involved or the level of thinking, trying to make it something that people can connect with a little more than Old West wagon trips.

In addition, we added elements of AMT according to our conceptions of AMT described above.

Figure 2 shows Version Two. The changes from version one include: changing representations of content to curricular materials; adding the LES lesson structure to the bottom of the triangle; and adding participation structures to the middle of the triangle. The placement of these last two elements signifies our ambivalence about their relationship to the nodes; while we recognized their importance and roles in classroom activity, as reported in the data, they did not neatly align with a particular node. We also included an arrow for student outcomes to acknowledge that the ultimate purpose of classroom activity was student learning of mathematics.

Process of iterating from version two to version three

To add additional conceptual and empirical detail in the next iteration, we sought feedback from three focus groups. Two of the focus groups consisted of educators from the research site and the third was a group of mathematics educators based in the San Diego area. The first focus group, held in November 2022, consisted of mathematics teachers and teacher leaders at the EPO while the second focus group, held in January 2023, consisted of teacher leaders and administrators at the EPO. These groups suggested adding elements such as: a list of essential curriculum outcomes generated via the Understanding by Design (UbD) process (Wiggins and McTighe, 2005); school wide initiatives, such as restorative justice practices, that impacted all aspects of the school; the support systems provided for students; and the role of relationships in supporting student risk taking in mathematics classrooms. The focus groups also mentioned that parts of the model were too specific or were incomplete. For example, they noted that the LES structure was not monolithic. Sometimes the structure spanned multiple class sessions and sometimes there were multiple LES cycles within a lesson. We conducted the third focus group in February 2023 with a group of educators from the San Diego area to understand how the model’s intent and utility was perceived by an outside group of educators. The feedback from this group mentioned missing elements that were similar to those of the EPO focus groups, notably the UbD transfer goals, the role of relationships, and the support provided for students. Our initial response to the focus group feedback was to add an element called Caring Relationships and to clarify aspects of the LES structure. The other suggestions pertained to things that emphasized phenomena that took place outside of the classroom; these suggestions were included in a model we were developing to represent the broader EPO efforts, including those that existed outside of mathematics classrooms. In addition, the conversations in the focus groups provided a broad impetus to continue revising version two.

The focus group feedback spurred the research groups to search for more conceptual and empirical detail. This led to the creation of two groups from the project team. The first group consisted of a postdoctoral fellow, a doctoral research assistant and one of the university-based external consultants who had supported the Upper School mathematics department since the inception of the EPO and was familiar with the design of the mathematics program. This group was charged with providing more conceptual detail to the model by articulating practices aligned with our conceptualization of AMT as well as practices articulated by EPO documents that guided the design of the mathematics program. We called this team the Conceptual Group. The second group, consisting of two doctoral research assistants, comprehensively scoured the data to generate empirical detail for the model and to align that detail with the elements identified by the Conceptual Group. We called this team the Empirical Group. Below, we describe the work from each of the groups.

Adding conceptual detail to the model

The primary work of the Conceptual Group was to provide more conceptual detail about how the EPO aspired to implement AMT and how these efforts related to the dimensions of AMT presented above. The group reviewed documents from the EPO (e.g., A Vision for Culturally Relevant and Responsive Pedagogy (CRRP), the Lesson Quality Checklist, and the Expeditionary Learning Appendix for Protocols and Resources) and explored the results of interviews with mathematics educators and instructional leaders that the Empirical Group provided. They changed the node name of students’ mathematical activity to student engagement because the focus group teachers at John Lewis perceived that the term mathematical activity referred to mathematical tasks rather than to the kinds of mathematical practices in which students engaged. The group created a list of over 60 practices associated with the dimensions of AMT with respect to the instructional triangle nodes (Instructional Practices, Curricular Materials, and Student Engagement). Initially, the Conceptual Group treated equity as a separate dimension of AMT. Through ongoing discussions in which they examined and connected specific practices within particular aspects of the instructional triangle, the group recognized the need to better capture the interwoven relationship between equity and the other AMT dimensions. The group thus incorporated multiple dimensions of equity into all three dimensions of AMT in recognition that equity is essential to all aspects of AMT. In addition, they created seven practices related to LES and 15 related to Caring Relationships. For reasons of space, we focus below on the nodes of the instructional triangle and omit discussion of LES and Caring Relationships.

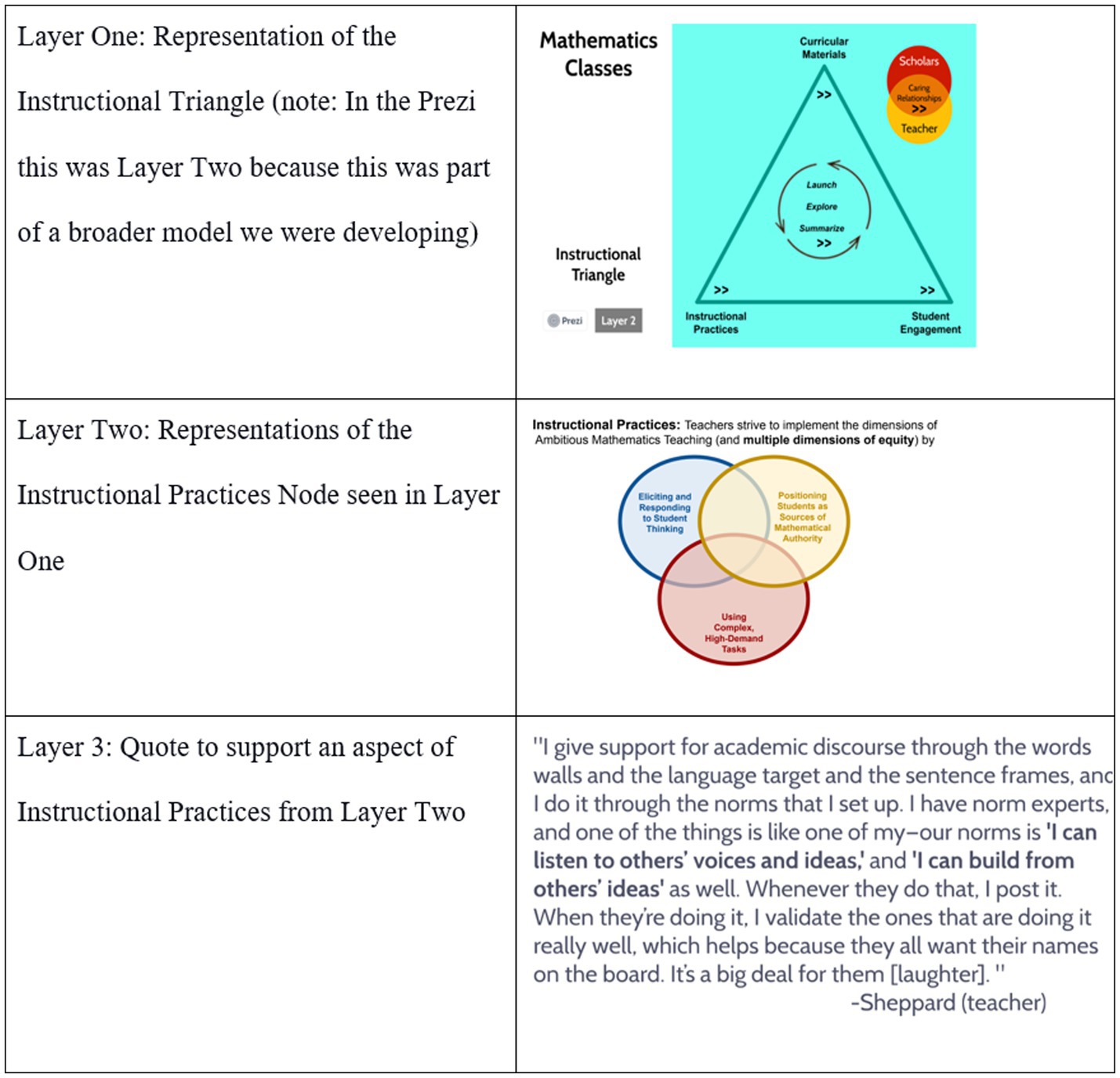
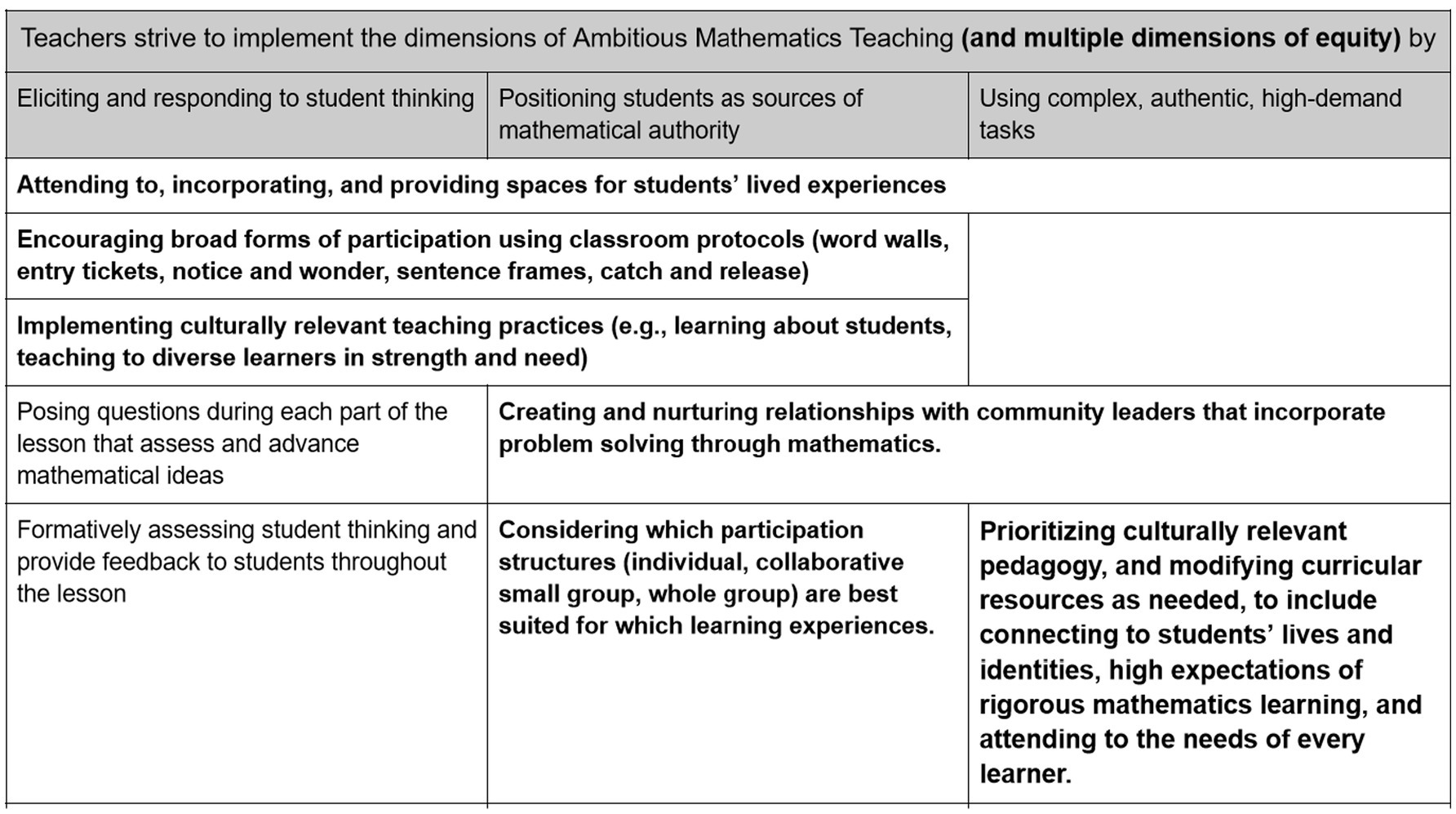
To map the connections between the dimensions of AMT and the nodes of the instructional triangle, the Conceptual Group created a matrix. To capture the interconnectedness of equity to the other three dimensions, they created equity rows that were potentially distributed across the other dimensions of AMT. See Figure 3 for an example related to the Instructional Practice node. In Figure 4, the gray cells list the three dimensions of AMT. The bolded text represents instructional practices specifically related to equity. In some cases, these practices spanned multiple dimensions of AMT, which is why some rows are distributed across multiple columns. The non-bolded text involves practices the Conceptual Group deemed as not explicitly related to equity.

Figure 3

Figure 3. Version three—three layer model.

Figure 4

Figure 4. Instructional practices matrix showing connections to AMT and integration of equity.

The descriptions of the practices included language that incorporated aspirations of the mathematics program rather than strictly actionable language. An example of a Student Engagement practice written in aspirational terms was Students engage in reasoning and sense-making, wrestling with important mathematical ideas, through interaction with high-demand tasks. This practice was associated with the AMT dimension Using complex, authentic, high-demand tasks. This practice aligned with the project definition of AMT, which stated that students should focus on important, broad ideas in mathematics [to provide opportunities] for students to invent strategies and approaches that emerge from their intuitive and everyday ways of thinking and acting in the world. The wording of this practice aligns the dimensions of AMT established by the project, EPO documents, and aspirations identified by the external consultant working with the Conceptual Group. The phrasing engages in reasoning and sense-making and wrestling with important mathematical ideas represents aspirations for student thinking rather than an actionable instructional practice. A second example of an instructional practice generated by this group was Students use a social justice oriented perspective with respect to mathematics that empowers them to critique the status quo and resist oppression and injustice. This practice was developed from the project’s working definition of AMT and the vision expressed in the EPO document A Vision for Culturally Relevant and Responsive Pedagogy. The working definition of AMT described the connection between the development of students’ positive mathematical identity and mathematics instruction that attends to students’ lived experiences, cultural forms of practice, and funds of knowledge. The vision of culturally relevant and responsive pedagogy adopted by John Lewis included the following two dimensions: Complex and Critical Thinking; and Empowerment, Self-Efficacy, and Initiative. These dimensions emphasize empowering students to critique the status quo, and resist oppression and injustice. We considered this practice as aspirational given the challenges of enacting it on a regular basis in mathematics classrooms. Overall, the 60 practices consisted of a mix of actionable and aspirational language that provided additional conceptual detail to what was represented in Version Two.

Adding empirical detail to the model

The empirical group was tasked with finding empirical support for the practices listed in Version Two and the additional practices generated by the conceptual group. We wanted to provide as much empirical detail to the model to inform others endeavoring similar efforts and to ensure that the model accurately represented the practices of the mathematics program at the EPO. The empirical support we sought was from interviews from mathematics educators and instructional leaders at the EPO, rather than from direct observation. Due to the simultaneous onset of COVID with the funding of our study, direct observation of the mathematics program was impacted in ways that made us cautious about using observational data. Much of our data consists of retrospective accounts of the development and implementation of the mathematics program over the first five years of the EPO. Consequently, even our empirical detail had an aspirational element in that participants may have described the goals and intentions of the program and not necessarily what occurred on a daily basis in classrooms.

The Empirical Group created a spreadsheet in which they listed the five elements of the revised model (Instructional Practices, Student Engagement, Curriculum Materials, Caring Relationships, and Launch-Explore-Summary) in the first column, the dimensions of AMT in the second column, and the specific practices in the third column. This allowed the group to map the specific practices to nodes in the instructional triangle and to dimensions of AMT. For each of the practices, the Empirical Group identified quotes from the interviews that related to the practices, with a range of zero to seven quotes for each practice. The research team decided that the proper grain size for which to document empirical support was the combination of instructional triangle node and dimension of AMT rather than specific practices given the sheer number of practices. Table 1 shows the number of practices there were for each of the instructional triangle node-AMT dimension combinations, and how many quotes the Empirical Group identified for each combination.

Table 1

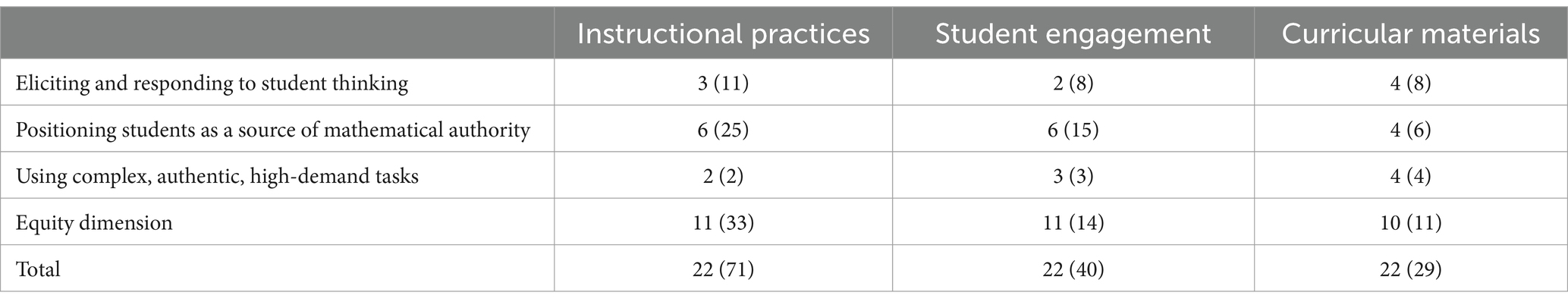
Table 1. Number of practices for each combination of instructional triangle node and dimension of AMT, with the number of quotes for each combination in parentheses.

We provide examples to illustrate how quotes corresponded to the different instructional triangle node-AMT dimension combinations. The first example is the Instructional Practice / Eliciting and Responding to Student Thinking combination, which had three practices associated with it (posing questions during each part of the lesson that assess and advance mathematical ideas; formatively assessing student thinking and provide feedback to students throughout the lesson; and developing students’ capacity to engage in mathematical argumentation and classroom discussions through the intentional use of discursive moves), with a total of 11 quotes identified for those practices. An example of a quote for this combination is:

What types of questions that [I’m] asking them as I'm going around the room? I don't want to stop at a table and they're stuck and I don't want to ask them a bunch of leading questions so that they can get the right answer. Right. I really need to think about how I can ask them questions to push their thinking, but not necessarily lead them to a particular answer that I want. (Bridges, Upper School teacher)

In the quote, Bridges emphasized how she focused on asking questions that pushed students’ thinking without giving away too much information. Two other quotes for this combination mentioned the interaction protocols teachers used to support students to engage in mathematical discourse. Shepherd, a 9th Grade Academy teacher, explained that she gave “support for academic discourse through the words walls and the language target and the sentence frames.” Matthews, an Upper School teacher leader, stated that he wanted “students to be able to explain their thinking, providing evidence at the appropriate sophistication for their grade level.”

A second example of an instructional node /dimension of the AMT combination is Student Engagement / Positioning Students as Sources of Mathematical Authority, which had six associated practices, which we do not list here for reasons of space, with a total of 15 quotes identified for those practices. Tewilliger, an Upper School teacher, described how students reflected on their mathematical understanding in class discussions:

We're putting much more emphasis on not—much more emphasis on explaining your answer. You're finding the solution or you're finding the statistics, but a huge emphasis has been put on explaining what that statistic tells you or what the relative risk tells you and making a claim about that.

Tewilliger described explaining reasoning as a form of student engagement in which students take responsibility for supporting claims in their statistics unit. Another quote for this combination is from Carter, an Upper School teacher, similarly stated, “we are making kids construct their own understanding. We’re making kids explain the process in their own words to each other and in writing. Then we are formalizing at the end.” Jones, an Upper School teacher, similarly reported that he emphasized students “talking to each other and having them struggle, and make meaning together, and writing their ideas down, bringing someone’s partial idea to the class, making it better by themselves and then sharing”.

Franklin, a Lower School teacher, recounted a student conversation, saying “yesterday we had an amazing discussion, we were matching graphs, and they disagreed on which one matched, then they had, like, a 10-min conversation about it”.

A third example of an instructional node /dimension of the AMT combination is Curricular Materials/ Using complex, authentic, high-demand tasks. This combination had four associated practices with a total of four supportive quotes. Matthews, an Upper School teacher leader, explained:

There's a lot of valuable thinking and growth that you go through in these kinds of problems and these kinds of discussions we have surrounding these problems. There’s also lots of times a lot more than one right way to solve a problem as opposed to the rote approach where it’s typically I think, “Here’s the method that works.”

Other teachers expressed similar perspectives of the curriculum, sometimes adding that the complexity of tasks required them to provide additional scaffolding to students.

The Empirical Group also identified quotes associated with the equity dimension of AMT for each of the nodes of the instructional triangle. For the 11 practices associated with the Instructional Practice/Equity combination there were 33 quotes, for the Student Engagement/Equity combination there were 11 practices and 14 quotes, and for the Curriculum Material / Equity combination there were 10 practices and 11 quotes. The equity dimension had considerable variation with respect to which practices had empirical support. The practice of encouraging broad forms of participation using classroom protocols (word walls, entry tickets, notice and wonder, sentence frames, catch and release), for example, had five quotes supporting it, while other practices, such as creating and nurturing relationships with community leaders that incorporate problem-solving through mathematics, had none.

The work of both groups added considerable complexity to the model, creating challenges to visually represent all of its elements. As a result, we made a decision to create layers for Version Three, a process we describe below.

Adding layers to the model

To add the additional empirical and conceptual details, we made a design decision to add layers to the model. We developed a Prezi version of the model that allowed us to place hyperlinks to navigate from one layer to the next. This meant creating a landing page for the instructional triangle that had hyperlinks for each of the elements on the landing page to navigate to the next layer. We called the landing page Layer One, with two subsequent layers. Layer Two for each element of Layer One added additional detail regarding the components of that element, while Layer Three consisted of data from EPO documents and the interviews compiled by the Empirical Group that pertained to the specific elements in Layer Two. See Figure 4 for an example of the three layers. Adding layers allowed us to resolve the granularity issue we encountered in Version Two and helped us to include both aspirational and empirical elements to the model, though it increased the complexity of displaying the model.

To generate feedback for the next iteration of the model, we shared the Prezi version of the model with three focus groups in fall 2023. These focus groups consisted of groups of mathematics educators from a range of mostly western US states. These focus groups consisted of teachers, instructional leaders, independent consultants, university teacher educators, and university researchers. We asked them a series of questions to understand how they interpreted the model and to gather feedback on making it more effective for catalyzing conversations in districts implementing ambitious mathematics programs. We used this feedback to inform the next iteration of the model, discussed below.

Continuing revisions of the model

The original project proposal included funding for a web developer to generate the final version of the model; consequently, we contracted with a web developer who had extensive experience creating websites for innovative educational projects. We used the Prezi as the basis for the design of the web version of the model, using the feedback from the focus groups to revise the content in the Prezi. The web version of the model provided more flexibility for displaying content in each layer of the model and made it easier to navigate within and across layers. In addition, the web version allowed for buttons to display quotes related to specific practices listed in lower layers of the model.

A feature of the web version of the model was the ability to create repositories of materials that complement the conceptual and empirical features of the model. Notably, we archived materials from several hundred mathematics lessons and linked them to assessments and essential goals articulated through the UbD process (Wiggins and McTighe, 2005). This added feature provided resources for educators who wished to study artifacts generated by the ambitious mathematics program. See Figure 5 for an example of a page from the web version of the model. Note that we changed the term student engagement to scholarly engagement to reflect terminology used by the EPO.

Figure 5

Figure 5. Page of the web version of the model.

Discussion

We started from a simple model and progressed to more complex and detailed models based on internal and external reflections on the model and what it represented. Even as the model gained in complexity, we intended for the model to help educators unfamiliar with the study site to understand the range of systems and resources associated with the reform in ways that might support their own efforts. Additionally, we intended for the visual nature of the model to generate insights into the components and relationships between components that would be difficult to generate if we relied solely on written text.

As we iterated the model, we began to conceptualize notions of rigor with respect to constructing visual models. We conceptualized rigor in terms of the conceptual and empirical foundations of the model. For the conceptual aspects, we conceptualized rigor in terms of connecting to external research literature and internal EPO documents that explained the broad principles that informed curriculum and instruction. To connect to external literature, we relied on our previous efforts to define ambitious mathematics instruction that encompassed a year-long review of literature in the first year of the project (Zahner et al., 2021). The Conceptual Group leveraged elements of AMT from our definition to identify practices that were then added to the model. In addition, the Conceptual Group studied the foundational documents from the EPO to identify core principles that informed all aspects of curriculum and instruction at the EPO. For the empirical aspects, we conceptualized rigor in terms of conducting iterative and comprehensive analysis of the 45 interviews of educators at the EPO to identify data that related to the specific practices noted in Version Two and the additional practices provided by the Conceptual Group. To further enhance the rigor of these elements, we conducted two EPO-based focus groups to validate our list of practices and descriptions of those practices. In sum, we conceptualized rigor as engaging in iterative and intensive cycles of exploration and validation to ensure that the elements of the model were warranted, conceptually and empirically.

As we iterated the model, we faced multiple tensions. One tension arose in relation to the balance between the aspirational and empirical aspects of the model. We wanted the model to represent the lived experience at the EPO but recognized that the aspirational aspects informed the lived practices, even if the aspirations were not achieved. Consequently, we recognized that models do not necessarily objectively represent reality but instead they represent, at least partially, idealized visions of a reform. Furthermore, reforms are not strictly about achieving outcomes but rather about engaging in improvement processes. Managing this tension also helped us to understand risks in trying to be too conceptual or too empirical even when the outcomes are uncertain. The danger in only emphasizing idealized outcomes is that most educators will be discouraged from attempting reform if the bar is unrealistically high. Conversely, if the details in the model are too mundane, educators will not be inspired to attempt such efforts. Thus, we were tasked with striking a balance between idealized conceptions of the reform and the lived reality.

A second tension emerged regarding the increasing complexity as we iterated the model. The feedback we received from the focus groups encouraged us to add more detail in order to better represent the vision of the mathematics program and its implementation. However, as we incorporated additional conceptual and empirical elements, it became increasingly challenging to display those details within a single visual. This led to the decision to add layers, which had other benefits in addition to adding detail; we struggled to represent the relationships between components as they increased, so creating layers provided another means of signaling relationships, both horizontally and vertically, between the components. While adding layers allowed us to add detail and communicate relationships between elements of the model, it detracted from the ability to quickly scan the model, which may hamper the intuitive insights the model may generate. The increasingly multidimensional and interactive nature of the model as it progressed from a single visual to a Prezi and then to a website raises questions about what constitutes a model and whether spaces such as a Prezi or a website fit the definition of a model.

Conclusion

Models are not intended to represent reality but instead serve to facilitate thinking about phenomena in new ways or to envision new forms of reality. This struggle to communicate a representation of the lived experiences of a reform effort and simultaneously its aspirational vision means that any model is imperfect. We struggled with finding the right balance between empirical and aspirational elements, ultimately deciding that doing so provided a means of inspiring a direction for reform rather than a prescription for reform.

We strove to visually represent a complex phenomenon. As the amount of detail increased, we had to balance text and visuals, parsimony and complexity, and static and interactive functions. When models are created through an iterative process of creation, feedback, and revision, these balances are difficult to maintain. This raises questions about what constitutes the defining features of a model and the affordances of models relative to more textual descriptions of reforms.

Models should facilitate insights into the relationships between the numerous components of complex educational phenomena. A question that we are left with is how our model does that. A goal of receiving feedback on Version Three from focus groups was to help us understand how others understand the model, the phenomenon it represents, and how it could inform conversations in their contexts. Even after three rounds of focus groups, we still had questions about how best to introduce the model and help others to explore it. These questions, doubts really, have informed the creation of the web-based version to help people make sense of the model.

Ethics statement

The studies involving humans were approved by University of Rochester Office for Human Subjects Protection. The studies were conducted in accordance with the local legislation and institutional requirements. The participants provided their written informed consent to participate in this study. Written informed consent was obtained from the individual(s) for the publication of any potentially identifiable images or data included in this article.

Author contributions

JC: Writing – original draft, Writing – review & editing. SA: Writing – original draft, Writing – review & editing. JL: Writing – original draft, Writing – review & editing. CW: Writing – original draft, Writing – review & editing. EC: Writing – original draft, Writing – review & editing.

Funding

The author(s) declare that financial support was received for the research and/or publication of this article. The work reported in this article was part of a project funded by the US National Science Foundation (Award Number 2010111).

Conflict of interest

The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.

Generative AI statement

The author(s) declare that no Gen AI was used in the creation of this manuscript.

Publisher’s note

All claims expressed in this article are solely those of the authors and do not necessarily represent those of their affiliated organizations, or those of the publisher, the editors and the reviewers. Any product that may be evaluated in this article, or claim that may be made by its manufacturer, is not guaranteed or endorsed by the publisher.

References

Anthony, G., Hunter, J., and Hunter, R. (2015). Supporting prospective teachers to notice students’ mathematical thinking through rehearsal activities. Math. Teach. Educ. Dev. 17, 17–24.

Google Scholar

Ball, D. L., and Forzani, F. M. (2009). The work of teaching and the challenge for teacher education. J. Teach. Educ. 60, 497–511. doi: 10.1177/0022487109348479

Crossref Full Text | Google Scholar

Bieda, K. N., Salloum, S. J., Hu, S., Sweeny, S., Lane, J., and Torphy, K. (2020). Issues with, and insights for, large-scale studies of classroom mathematical instruction. J. Classr. Int. 55, 41–63.

Google Scholar

Boaler, J., and Staples, M. (2008). Creating mathematical futures through an equitable teaching approach: the case of Railside school. Teach. Coll. Rec. 110, 608–645. doi: 10.1177/016146810811000302

Crossref Full Text | Google Scholar

Boston, M. (2012). Assessing instructional quality in mathematics. Elem. Sch. J. 113, 76–104. doi: 10.1086/666387

Crossref Full Text | Google Scholar

Boston, M. D., and Candela, A. G. (2018). The instructional quality assessment as a tool for reflecting on instructional practice. ZDM: the international journal on. Math. Educ. 50, 427–444. doi: 10.1007/s11858-018-0916-6

PubMed Abstract | Crossref Full Text | Google Scholar

Brown, J. S., Collins, A., and Duguid, P. (1989). Situated cognition and the culture of learning. Educ. Res. 18, 32–42. doi: 10.3102/0013189X018001032

Crossref Full Text | Google Scholar

Choppin, J. (2025). “Reconceptualizing analysis of tasks in curriculum materials” in Paper presented at the fifth international conference on mathematics textbook Research and Development (Trondheim, Norway).

Google Scholar

Choppin, J., Green, C., and Zahner, W. (2024). Demands, tensions, and resources when implementing ambitious mathematics. Educ. Policy Anal. Arch. 32. doi: 10.14507/epaa.32.8098

Crossref Full Text | Google Scholar

Cohen, D. K., and Ball, D. L. (1999). Instruction, capacity, and improvement (CPRE Research Report No. RR-43). Philadelphia: University of Pennsylvania, Consortium for Policy Research in Education.

Google Scholar

Cohen, D. K., Raudenbush, S. W., and Ball, D. L. (2003). Resources, instruction, and research. Educ. Eval. Policy Anal. 25, 119–142. doi: 10.3102/01623737025002119

Crossref Full Text | Google Scholar

Engeström, Y. (2001). Expansive learning at work: toward an activity theoretical reconceptualization. J. Educ. Work. 14, 133–156. doi: 10.1080/13639080020028747

Crossref Full Text | Google Scholar

Franke, M. L., Kazemi, E., and Battey, D. (2007). “Mathematics teaching and classroom practice” in Second handbook of research on mathematics teaching and learning. ed. F. K. Lester (Charlotte, NC: Information Age), 225–256.

Google Scholar

Gravemeijer, K., and Doorman, M. (1999). Context problems in realistic mathematics education: a calculus course as an example. Educ. Stud. Math. 39, 111–129. doi: 10.1023/a:1003749919816

Crossref Full Text | Google Scholar

Henderson, S., and Segal, E. H. (2013). Visualizing qualitative data in evaluation research. N. Dir. Eval. 2013, 53–71. doi: 10.1002/ev.20067

PubMed Abstract | Crossref Full Text | Google Scholar

Horn, I. S. (2012). Strength in numbers: Collaborative learning in secondary mathematics. Reston, VA: National Council of Teachers of Mathematics.

Google Scholar

Jacobs, V. R., Lamb, L. L. C., and Philipp, R. (2010). Professional noticing of children’s mathematical thinking. J. Res. Math. Educ. 41, 169–202. doi: 10.5951/jresematheduc.41.2.0169

Crossref Full Text | Google Scholar

Kelley-Peterson, M. (2010). Understanding ambitious mathematics teaching practice through instructional activities. Available online at: http://libproxy.sdsu.edu/login?url=https://www-proquest-com.libproxy.sdsu.edu/docview/881461926?accountid=13758

Google Scholar

Lampert, M., Beasley, H., Ghousseini, H., Kazemi, E., and Franke, M. (2010). “Using designed instructional activities to enable novices to manage ambitious mathematics teaching” in Instructional explanations in the disciplines. eds. M. K. Stein and L. Kucan (New York, NY: Springer), 129–141.

Google Scholar

Lankshear, C., and Knobel, M. (2006). “Blogging as participation: the active sociality of a new literacy” in Paper presented at the American Educational Research Association (San Francisco, CA). Available at: http://www.geocities.com/c.lankshear/bloggingparticipation.pdf

Google Scholar

Larson, J., and Nelms, S. (2021). Collaborating for equity in urban education: comprehensive reform in an innovative university/school partnership. Urban Educ. 58, 2346–2377. doi: 10.1177/00420859211017976

Crossref Full Text | Google Scholar

Lerman, S. (2001). Cultural, discursive psychology: a sociocultural approach to studying the teaching and learning of mathematics. Educ. Stud. Math. 46, 87–113. doi: 10.1023/A:1014031004832

Crossref Full Text | Google Scholar

Milner, R. (2012). But what is urban education? Urban Educ. 47, 556–561. doi: 10.1177/0042085912447516

Crossref Full Text | Google Scholar

Moschkovich, J. (1999). Supporting the participation of English language learners in mathematical discussions. Learn. Math. 19, 11–19.

Google Scholar

Moschkovich, J., and Brenner, M. E. (2002). Preface. In M. E. Brenner & J. Moschkovich (Eds.), Everyday and academic mathematics in the classroom (pp. v-x). NCTM.

Google Scholar

NCTM Research Committee (2018). Asset-based approaches to equitable mathematics education research and practice. J. Res. Math. Educ. 49, 373–389. doi: 10.5951/jresematheduc.49.4.0373

Crossref Full Text | Google Scholar

Radnofsky, M. L. (1996). Qualitative models: visually representing complex data in an image/text balance. Qual. Inq. 2, 385–410. doi: 10.1177/107780049600200402

Crossref Full Text | Google Scholar

Rezat, S., and Sträßer, R. (2012). The didactical tetrahedron as a heuristic for analysing the incorporation of digital technologies into classroom practice in support of investigative approaches to teaching mathematics. ZDM: the international journal on. Math. Educ. 44, 641–651. doi: 10.1007/s11858-012-0448-4

Crossref Full Text | Google Scholar

Roth, W.-M., and Jornet, A. (2013). Situated cognition. WIREs. Cogn. Sci. 4, 463–478. doi: 10.1002/wcs.1242

PubMed Abstract | Crossref Full Text | Google Scholar

Ruthven, K. (2012). The didactical tetrahedron as a heuristic for analysing the incorporation of digital technologies into classroom practice in support of investigative approaches to teaching mathematics. Int. J. Math. Educ. 44, 627–640. doi: 10.1007/s11858-011-0376-8

PubMed Abstract | Crossref Full Text | Google Scholar

Saldaña, J. (2013). Fundamentals of qualitative research. Oxford: Oxford University Press.

Google Scholar

Singer-Gabella, M., Stengel, B., Shahan, E., and Kim, M.-J. (2016). Learning to leverage student thinking: what novice approximations teach us about ambitious practice. Elem. Sch. J. 116, 411–436. doi: 10.1086/684944

Crossref Full Text | Google Scholar

Strauss, A. (1987). Qualitative analysis for social scientists. New York, NY: Cambridge University Press.

Google Scholar

van Es, E. A., and Sherin, M. G. (2008). Mathematics teachers “learning to notice” in the context of a video club. Teach. Teach. Educ. 24, 244–276. doi: 10.1016/j.tate.2006.11.005

PubMed Abstract | Crossref Full Text | Google Scholar

Vygotsky, L. (1986). “The development of scientific concepts in childhood: the design of a working hypothesis” in Thought and language (Cambridge, MA: The MIT Press), 146–209.

Google Scholar

Wertsch, J. V. (1991). Voices of the mind: A sociocultural approach to mediated action. Cambridge, MA: Harvard University Press.

Google Scholar

Wiggins, G., and McTighe, J. (2005). Understanding by design. Alexandria, VA: Association for Supervision and Curriculum Development.

Google Scholar

Zahner, W., Green, C., Tenney, K., Pelaez, K., Choppin, J., and Al, S. (2021). “What is ambitious mathematics teaching? A literature synthesis” in Paper presented at the forty-third annual meeting of the north American chapter of the International Group for the Psychology of mathematics education (Philadelphia, PA).

Google Scholar