- 1Department of Mathematics, Faculty of Science, Davangere University, Davangere, India
- 2Department of Mathematics, Karnatak University, Dharwad, India
- 3Department of Mathematics, JECRC University, Jaipur, India
In this paper, we find the solution for a fractional Richards equation describing the water transport in unsaturated porous media using the q-homotopy analysis transform method (q-HATM). The proposed technique is to use graceful amalgamations of the Laplace transform technique with the q-homotopy analysis scheme as well as the fractional derivative that is defined with the Atangana-Baleanu (AB) operator. The fixed point hypothesis is considered in order to demonstrate the existence and uniqueness of the obtained solution for the proposed fractional order model. In order to validate and illustrate the efficiency of the future technique, we analyze the projected model in terms of fractional order. Meanwhile, the physical behavior of the q-HATM solutions are captured in terms of plots for diverse fractional order and the numerical simulation is also demonstrated. The achieved results illuminate that the future algorithm is easy to implement, highly methodical, effective, and very accurate in its analysis of the behavior of non-linear differential equations of fractional order that arise in the connected areas of science and engineering.
Introduction
Fractional calculus (FC) was originated in Newton's time, but, lately, it has fascinated and captured the attention of many scholars. For the last 30 years, the most intriguing leaps in scientific and engineering applications have been found within the framework of FC. The concept of the fractional derivative has been industrialized due to the complexities associated with a heterogeneous phenomenon. The fractional differential operators are capable of capturing the behavior of multifaceted media as they have diffusion processes. It has been a very essential tool, and many problems can be illustrated more conveniently and more accurately with differential equations having an arbitrary order. Due to the swift development of mathematical techniques that use computer software, many researchers started to work on generalized calculus to present their viewpoints while analyzing many complex phenomena.
Numerous pioneering directions are prescribed for the diverse definitions of fractional calculus by many senior researchers, and these have prearranged the foundation [1–6]. Calculus with fractional order is associated with practical ventures and is extensively employed within nanotechnology [7], optics [8], human diseases [9], chaos theory [10], and other areas [11–39]. The numerical as well as analytical solutions for these equations illustrate that these models have an important role in portraying the nature of non-linear problems within connected areas of science.
In order to illustrate the importance of the novel fractional order derivative and future scheme, we, in the present framework, consider the Richards equation, which plays a vital role in describing the nature of the porous medium as well as the penetration of unsaturated regions in the soil. In 1931, Lorenzo A. Richards was the first person to pioneer work on the unsaturated porous material in order to model water movement. Later, he derived an equation based on continuum mechanics, which govern the water flow in the soil [40]. In the proposed model for the momentum equation, the continuity equation is an amalgam with Darcy's law, and is defined in a one-dimensional form as follows, with soil water diffusivity symbolized by ρ and hydraulic conductivity by σ for unsaturated soil moisture content u
where z designates the elevation above a vertical datum. Recently, many authors employed numerical as well as analytical techniques in order to analyze and predict the suitable models for parameters in the equation and solve the governing equation of unsaturated flow in soils. Meanwhile, three models are generally applied, namely (i) the exponential model, (ii) the van Genuchten model, and (iii) the Brook-Coreysmodel (BCM). Among these models, BCM is extensively applied due to its well-defined configuration and because it is associated with the largest pore size. The following equations describe the complete wet ability of the BC model [41, 42]:
where σ0, k, ρ0, and n are constants denoting particle shape, pore-size distribution and many other soil properties. For n = 0 and k = 2, Equation (2) simplified it to the classic Burgers equation [43, 44], and some particular values signify the generalized Burgers equation, which is essential to describing the important physical phenomena. In the present study, we consider that BCM employed the RC equation. In this case, for the (n, 1) order, the RC equation coincides with the Burgers equation, and this is presented here [45, 46]:
The analytical solution for the above equation is presented:
In the present scenario, many important and non-linear models are methodically and effectively analyzed with the help of fractional calculus. There have been diverse definitions that have been suggested by many senior research scholars like, Riemann, Liouville, Caputo, and Fabrizio. However, these definitions have their own limitations. The Riemann-Liouville derivative is unable to explain the importance of the initial conditions; the Caputo derivative has overcome this shortcoming but cannot explain the singular kernel of the phenomena. In 2015, Caputo and Fabrizio solved the above issues [47], and many researchers consult this derivative in order to analyze and find the solution for diverse classes of non-linear complex problems. Some issues, however, were pointed out in the CF derivative; non-singular kernel and non-local properties are very essential in describing the physical behavior and nature of the non-linear problems. In 2016, Atangana and Baleanu introduced and natured a novel fractional derivative, namely the AB derivative. This novel derivative was defined with the aid of Mittag-Leffler functions [48]. This fractional derivative buried all the above-cited issues and helps us to understand the natural phenomena in the systematic and effective way.
In this framework, we consider the fractional RC equation of the form
where α is fractional order of the system and defined with AB fractional operator, u is the water content with depth x. The fractional order is introduced in order to incorporate the memory effects and hereditary consequence in the system, and these properties aid us in capturing the essential physical properties of the complex problems.
Recently, many mathematicians and physicists have developed very effective and more accurate methods in order to find and analyze solutions for complex and non-linear problems that have arisen in science and technology. In connection with this is the homotopy analysis method (HAM) proposed by Chinese Mathematician Liao Shijun [49, 50]. HAM has been profitably and effectively applied to study the behavior of non-linear problems without perturbation or linearization. But, for computational work, HAM requires significant time and computer memory. To overcome this, there is a possibility of using an amalgamation of the considered method and well-known transformation techniques.
In the present investigation, we analyzed the nature of the q-homotopy analysis transform method (q-HATM) solution for the FCDG equation by applying q-HATM. The future algorithm is the combination of q-HAM with LT [51]. The method of the considered scheme is merging two strong methods to solve linear and non-linear fractional differential equations both analytically as well as numerically. The future technique has many sturdy properties, including a non-local effect, straight forward solution procedure, and a promising large convergence region; moreover, it is free from any assumptions, discretization, and perturbation. Recently, due to its reliability and efficacy, the considered method has been exceptionally applied by many researchers to understand physical behavior in diverse classes of complex problems [52–60]. The novelty of the future method is that it aids a modest algorithm to evaluate the solution, and it is natured by the homotopy and axillary parameters, which provide the rapid convergence of the obtained solution for a non-linear portion of the given problem. Meanwhile, it has prodigious generality because it plausibly contains the results obtained by many algorithms like q-HAM, HPM, ADM and some other traditional techniques. The considered method can preserve great accuracy while decreasing the computational time and work in comparison with other methods.
The considered non-linear model recently caught the attention of researchers from different areas of science. Since RC equation plays a significant role in portraying several complex phenomena, many authors have found and analyzed the solution using analytical as well as numerical schemes; for instance, authors in [61] considered analytical techniques and found solutions for the considered model with arbitrary surface boundary conditions, and authors in [62] presented the compression approximation and infiltration of the RC equation with an analytical solution, authors in [45] applied the Adomian decomposition scheme, and authors in [46] applied HAM in order to find the approximated analytical solution. In this paper, we made an attempt to find the solution for the FRC equation using q-HATM.
Preliminaries
Recently, many authors considered these derivatives to analyze a diverse class of models in comparison with classical order as well as other fractional derivatives, and they prove that the AB derivative is more effective while analyzing the nature and physical behavior of the models [63, 64]. Here, we define the basic notion of Atangana-Baleanu derivatives and integrals [48].
Definition 1. The fractional Atangana-Baleanu-Caputo derivative for a function f ∈ H1 (a, b) (b > a, α ∈ [0, 1]) is presented:
Definition 2. The AB derivative of fractional order for a function f ∈ H1 (a, b), b > a, α ∈ [0, 1] in the Riemann-Liouville sense is presented:
Definition 3. The fractional AB integral related to the non-local kernel is defined by
Definition 4. The Laplace transform (LT) of AB derivative is defined by
Theorem 1. The following Lipschitz conditions, respectively, hold true for both Riemann-Liouville and AB derivatives defined in Equations (6) and (7) [48],
and
Theorem 2. The time-fractional differential equation has a unique solution, which is defined as [48]
Fundamental Idea of the Proposed Scheme
Here, we consider the arbitrary order differential equation in order to demonstrate the basic solution procedure [65, 66]
with the initial condition
where symbolize the AB derivative of v(x, t). On using the LT on Equation (13), we have after simplification
The non-linear operator is presented as
Here, φ(x, t; q) is the real valued function with respect to x, t and . Now, we define a homotopy as follows
where L is signifies LT, is the embedding parameter and ℏ ≠ 0 is an auxiliary parameter. For q = 0 and , the results given below are hold true
Now, by intensifying q from 0 to , then φ(x, t; q) varies from v0(x, t) to v(x, t). By using the Taylor theorem near to q, we define φ(x, t; q) in series form and then we get
where
The series (16) converges at for the proper chaise of v0 (x, t), n and ℏ. Then
On m-times differentiating Equation (17) with q and lately dividing by m! and then substituting q = 0, we get
where the vectors are defined as
On employing the inverse LT on Equation (22), we have
where
and
In Equation (25), signifies a homotopy polynomial and presented as follows
By the aid of Equations (24) and (25), one can get
Then, the terms of vm(x, t) we can obtain using the Equation (28). The q-HATM series solution is presented as
Solution for FRC Equation
In order to present the solution procedure and efficiency of the future scheme, in this segment we consider the DSW equation of fractional order with two distinct cases. Further, by the help of obtained results we made an attempt to capture the behavior of q-HATM solution for different fractional order. By the help of Equation (5) for the function of cubic water content and constant, we have
with initial conditions
Taking LT on Equation (29) and then using Equation (30), we get
The non-linear operator N is presented with the help of future algorithm as below
The deformation equation of m-th order by the help of q-HATM at ℋ(x, t) = 1, is given as follows
where
On applying inverse LT on Equation (34), it reduces to
On simplifying the above equation systematically by using u0 (x, t), we can evaluate the terms of the series solution
Existence of Solutions for the Future Model
Here, we considered the fixed-point theorem in order to demonstrate the existence of the solution for the proposed model. Since the considered model cited in Equation (30) is non-local as well as complex, there are no particular algorithms or methods that exist to evaluate the exact solutions. However, under some particular conditions, the existence of the solution is assured. Now, Equation (30) is considered:
The foregoing system is transformed to the Volterra integral equation using the Theorem 2 as follows
Theorem 3. The kernel g satisfies the Lipschitz condition and contraction if the condition 0 ≤ (δ (a2 + b2 + ab)− δ2) < 1 holds.
Proof. In order to prove the required result, we consider the two functions u and u1, then
where a = ||u|| and b = ||u1|| (since u and u1 are the bounded functions). Putting η = δ (a2 + b2 + ab) − δ2 in the above inequality, then we have
The Lipschitz condition is thus obtained for . Further, we can see that if 0 ≤ (δ (a2 + b2 + ab)− δ2) < 1, then it implies the contraction. The recursive form of Equation (36) is defined as
The associated initial condition is
The successive difference between the terms is presented as
Notice that
By using Equation (39) after applying the norm on the Equation (43), one can get
We prove the following theorem by using the above result.
Theorem 4. The solution for the Equation (30) will exist, and if we have specific t0, then
Proof. Let us consider the bounded function u(x, t) satisfying the Lipschitz condition. Then, by Equation (43), we have
Therefore, the continuity as well as existence of the obtained solution is proved. Subsequently, in order to show the Equation (46) is a solution for the Equation (29), we consider
In order to obtain require a result, we consider
Similarly, at t0 we can obtain
As n approaches to ∞, we can see that form Equation (49), ||n (x, t)|| tends to 0.
Next, it is a necessity to demonstrate uniqueness for the solution of the considered model. Suppose u*(x, t) is the other solution, then we have
On applying norm, the Equation (50) simplifies to
On simplification
From the above condition, it is clear that u(x, t) = u*(x, t), if
Hence, Equation (53) proves our essential result.
Theorem 5. Suppose un (x, t) and u (x, t) are defined in the Banach space (𝔅[0, T], ||·||). The series solution defined in Equation (29) converges to the solution of the Equation (13), if 0 < λ < 1.
Proof: Consider the sequence {n}, which is the partial sum of the Equation (29), and we have to prove {n} is the Cauchy sequence in (𝔅[0, T], ||·||). Now consider
Now, we have for every n, m ∈ N (m ≤ n)
But 0 < λ < 1, therefore ||n − m|| = 0. Hence, {n} is the Cauchy sequence. This proves the required result.
Numerical Results and Discussion
In the present investigation, we have found the solution for equation describing the water transport in unsaturated porous media using q-HATM with the help of Mittag-Leffler law. Here, we consider two distinct cases to present the effectiveness of the proposed method.
Case 1: In this case, we consider the conductivity term as a function of cubic water content and constant and ρ = 1 cm2/h. At and b = −1, Equation (4) becomes
with initial condition
Case 2: In this segment, we consider the conductivity term as a function of quadric water content and constant and ρ = 1 cm2/h. At and b = −1, the Equation (4) becomes
with initial condition
Here, we demonstrate the numerical simulation for the considered non-linear. In Tables 1 and 2, the error analysis has been validated. From the tables we can see that the proposed scheme is more accurate, and we confirm that the iterations increase the q-HATM solutions so that they get closer to the analytical solution.
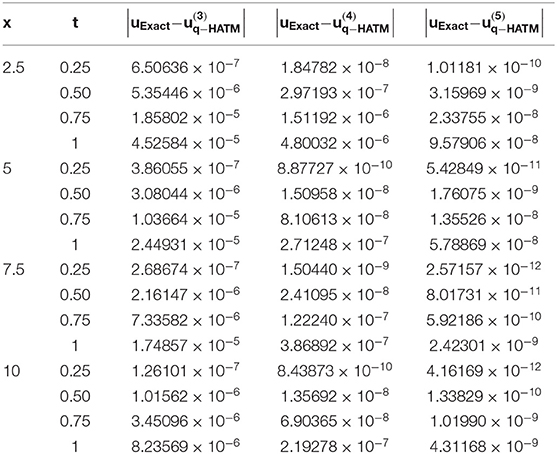
Table 1. Numerical simulation presented for u(x, t) of FR equation consider in Case 1 at n = 1, ℏ = −1 and α = 1.

Table 2. Numerical simulation presented for u(x, t) of FR equation consider in Case 2 at n = 1, ℏ = −1 and α = 1.
The surfaces of the obtained solution and the exact solution in comparison with absolute error have been captured, respectively, in Figures 1 and 2 for Case 1 and Case 2. The behavior of the obtained solution for different orders is presented in Figure 3 for both the cases in terms of 2D plots. In order to analyze the variations of the obtained solution for the FRC equation cited in Case 1 and Case 2 with respect to the homotopy parameter (ℏ), and the (ℏ) curves are drawn for diverse μ and presented in Figure 4 with distinct n. In the plots, the horizontal line signifies the convergence region of the q-HATM solution and these curves aid us to adjust and handle the convergence province of the solution. For an appropriate value of ℏ, the achieved solution quickly tends to the exact solution. The small deviation in the physical behavior of the complex models stimulates the enormous new results to analyze and understand the nature in a better and systematic manner. Moreover, from all the plots we can see that the proposed method is more accurate and very effective in its analysis of the considered non-linear fractional order equations.
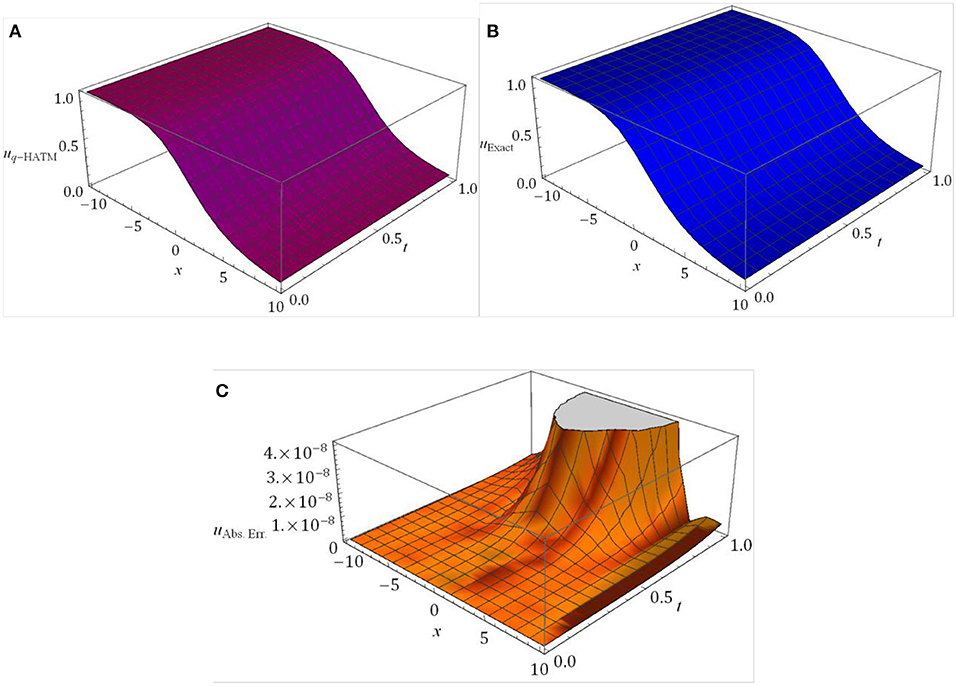
Figure 1. Surfaces of (A) uq−HATM, (B) uExact (C) uAbs. Err. = |uExact − uq−HATM| for FR equation considered in Case 1 at ℏ = −1, n = 1 and α = 1.
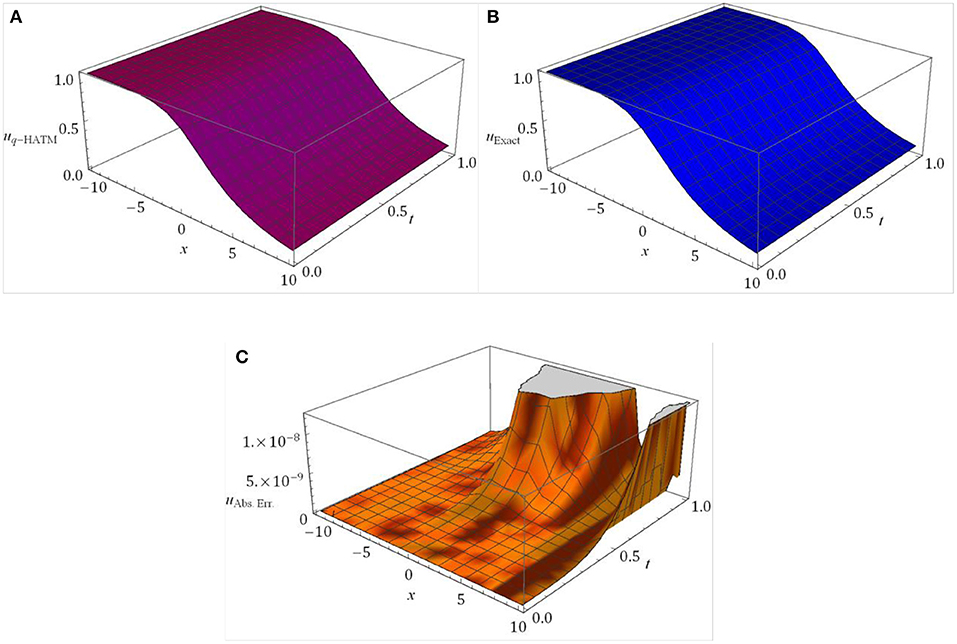
Figure 2. Surfaces of (A) uq−HATM, (B) uExact (C) uAbs. Err. = |uExact − uq−HATM| for FR equation considered in Case 2 at ℏ = −1, n = 1 and α = 1.
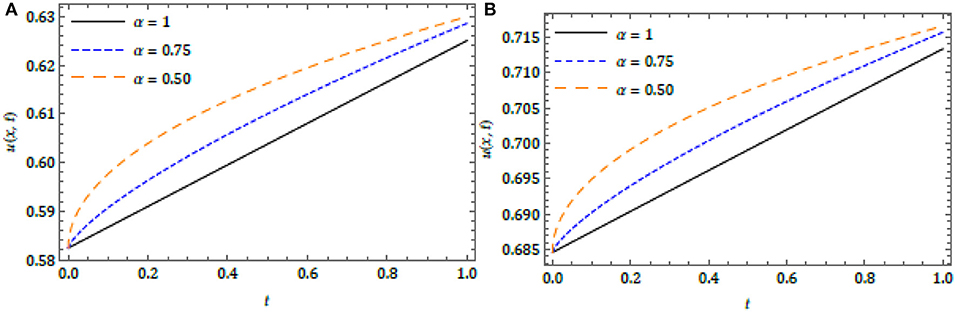
Figure 3. Nature of theq-HATM solution for (A) Case 1 and (B) Case 2 with distinct α at ℏ = −1, n = 1 and x = 1.
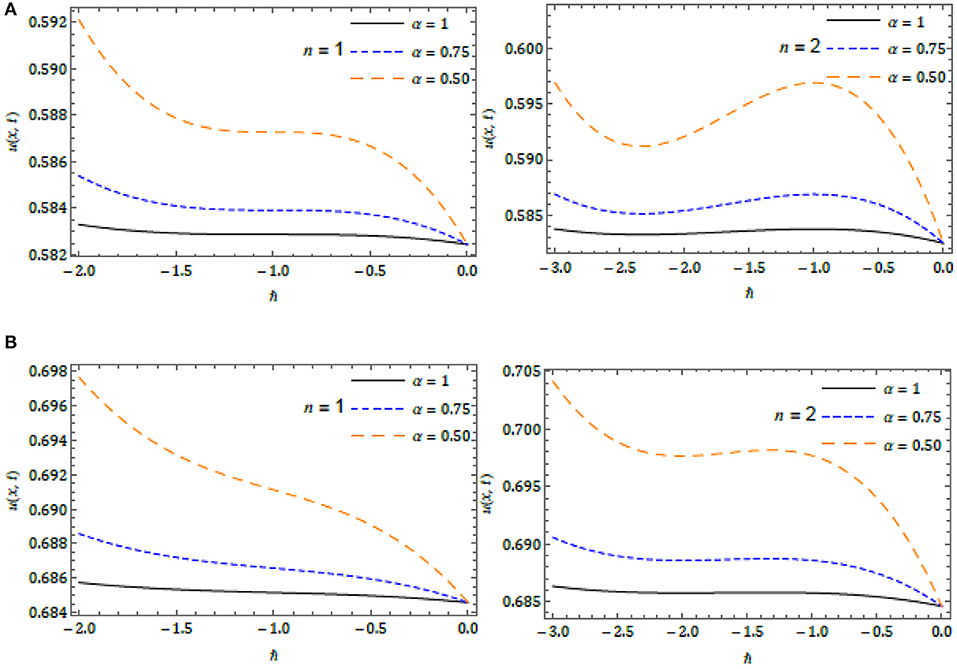
Figure 4. ℏ-curves for (A) Case 1 (B) Case 2 with distinct α at x = 1 and t = 0.01 with n = 1 and 2.
Since every non-linear differential equation does not have an exact solution we look for an approximated analytical solution thorugh which we can prove the exactness or accuracy of the proposed scheme, as opposed to an exact solution. As we mentioned earlier, the q-HATM is a modified algorithm of HAM, and it thus does not require perturbation, dissertation, linearization, or any assumptions. More importantly, the future method generalizes many traditional techniques, such as HAM, HPM, FRDTM, and others, because these are a special case of q-HATM (n = 1, ℏ = 1). In connection with this, we capture the physical behavior of q-HATM solution to illustrate the accuracy. Further, we noticed that the considered non-linear phenomenon is highly dependent on a fractional operator. In order to illustrate the computational level and computational cost, the numerical simulation has been presented. From the table, it shows that as a number of series terms increases the solution converges to an analytical solution.
Conclusion
In this paper, the q-HATM is applied profitably to find the solution for an arbitrary order RC equation describing the water transport in the unsaturated porous media. Since AB derivatives and integrals having fractional order are defined with the help of generalized Mittag-Leffler function as the non-local kernel and non-singular, the present investigation illuminates the effectiveness of the considered derivative. The existence and uniqueness of the obtained solution is demonstrated by the fixed point hypothesis. The results obtained by the future scheme are more stimulating as compared to results available in the literature. Further, the proposed algorithm finds the solution of the non-linear problem without considering any discretization, perturbation or transformations.
The behavior of the obtained series solution has been captured in terms of 2D and 3D plots for distinct fractional order. These plots show that the q-HATM solution is more accurate and also conformed with the help of numerical simulation, and this is cited in the tables. Further, we confirm that, as the order of the solution increases, the obtained solutions converge to the exact solution. The present investigation illuminates how the considered complex non-linear phenomena noticeably depend on the time history and the time instant, which can be proficiently analyzed by applying the concept of calculus to fractional order. The present investigation helps the researchers to study the behavior of non-linear problems, and this gives very interesting and useful consequences. The proposed derivative provides non-singular kernel and non-local properties; these properties are very essential in describing the physical behavior and nature of the non-linear problems, and hence researchers can consider the AB derivative to solve many non-linear complex problems. Lastly, we can conclude the projected method is extremely methodical, effective and very accurate, and that it can be applied to the analysis of the diverse classes of non-linear problems that exist in science and technology.
Data Availability Statement
All datasets generated for this study are included in the article/supplementary material.
Author Contributions
All the authors have worked equally in this manuscript. All the authors have read and approved the final manuscript.
Conflict of Interest
The authors declare that the research was conducted in the absence of any commercial or financial relationships that could be construed as a potential conflict of interest.
References
1. Liouville J. Memoire surquelques questions de geometrieet de mecanique, etsur un nouveau genre de calcul pour resoudreces questions. JEcolePolytech. (1832) 13:1–69.
2. Riemann GFB. Versuch Einer Allgemeinen Auffassung der Integration und Differentiation. Leipzig: Gesammelte Mathematische Werke (1896).
4. Miller KS, Ross B. An Introduction to Fractional Calculus and Fractional Differential Equations. New York, NY: Wiley (1993).
6. Kilbas AA, Srivastava HM, Trujillo JJ. Theory and Applications of Fractional Differential Equations. Amsterdam: Elsevier (2006).
7. Baleanu D, Guvenc ZB, Tenreiro Machado JA. New Trends in Nanotechnology and Fractional Calculus Applications. London; New York, NY: Springer Dordrecht Heidelberg (2010). doi: 10.1007/978-90-481-3293-5
8. Esen S, Sulaiman TA, Bulut H, Baskonus HM. Optical solitons and other solutions to the conformable space-time fractional Fokas-Lenells equation. Optik. (2018) 167:150–6. doi: 10.1016/j.ijleo.2018.04.015
9. Veeresha P, Prakasha DG, Baskonus HM. Solving smoking epidemic model of fractional order using a modified homotopy analysis transform method. Math Sci. (2019) 13:115–28. doi: 10.1007/s40096-019-0284-6
10. Baleanu D, Wu GC, Zeng SD. Chaos analysis and asymptotic stability of generalized Caputo fractional differential equations. Chaos Solitons Fractals. (2017) 102:99–105. doi: 10.1016/j.chaos.2017.02.007
11. Veeresha P, Prakasha DG, Baskonus HM. New numerical surfaces to the mathematical model of cancer chemotherapy effect in Caputo fractional derivatives Chaos. (2019) 29:013119. doi: 10.1063/1.5074099
12. Baskonus HM, Sulaiman TA, Bulut H. On the new wave behavior to the Klein-Gordon-Zakharov equations in plasma physics. Indian J Phys. (2019) 93:393–9. doi: 10.1007/s12648-018-1262-9
13. Prakasha DG, Veeresha P, Baskonus HM. Analysis of the dynamics of hepatitis E virus using the Atangana-Baleanu fractional derivative, Eur Phys J Plus. (2019) 134:1–11. doi: 10.1140/epjp/i2019-12590-5
14. Veeresha P, Prakasha DG, Baleanu D. An efficient numerical technique for the nonlinear fractional Kolmogorov-Petrovskii-Piskunov equation. Mathematics. (2019) 7:1–18. doi: 10.3390/math7030265
15. Yokuş A, Gülbahar S. Numerical solutions with linearization techniques of the fractional Harry Dym equation. Appl Math Nonlinear Sci. (2019) 4:35–42. doi: 10.2478/AMNS.2019.1.00004
16. Cattani C. Haar wavelet-based technique for sharp jumps classification. Math Comp Model. (2004) 39:255–78. doi: 10.1016/S0895-7177(04)90010-6
17. Yang A-M, Zhang Y-Z, Cattani C, Xie G-N, Rashidi MM, Zhou Y-J, et al. Application of local fractional series expansion method to solve Klein-Gordon equations on Cantor sets. Abstr Appl Anal. (2014) 2014:372741. doi: 10.1155/2014/372741
18. Zhang Y, Cattani C, Yang X-J. Local fractional homotopy perturbation method for solving non-homogeneous heat conduction equations in fractal domains. Entropy. (2015) 17:6753–64. doi: 10.3390/e17106753
19. Prakasha DG, Veeresha P, Baskonus HM. Residual power series method for fractional Swift–Hohenberg equation. Fractal Fract. (2019) 3:1–9. doi: 10.3390/fractalfract3010009
20. Singh J, Kumar D, Hammouch Z, Atangana A. A fractional epidemiological model for computer viruses pertaining to a new fractional derivative. Appl Math Comput. (2018) 316:504–15. doi: 10.1016/j.amc.2017.08.048
21. Asif NA, Hammouch Z, Riaz MB, Bulut H. Analytical solution of a Maxwell fluid with slip effects in view of the Caputo-Fabrizio derivative. Eur Phys J Plus. (2018) 133:272. doi: 10.1140/epjp/i2018-12098-6
22. Veeresha P, Prakasha DG, Magesh N, Nandeppanavar MM, Christopher AJ. Numerical simulation for fractional Jaulent–Miodek equation associated with energy-dependent Schrödinger potential using two novel techniques. Waves Random Complex Media. (2019). doi: 10.1080/17455030.2019.1651461. [Epub ahead of print].
23. Baskonus HM, Mekkaoui T, Hammouch Z, Bulut H. Active control of a chaotic fractional order economic system. Entropy. (2015) 17:5771–83. doi: 10.3390/e17085771
24. Ravichandran C, Jothimani K, Baskonus HM, Valliammal N. New results on nondensely characterized integrodifferential equations with fractional order, EurPhys J Plus. (2018) 133:1–10. doi: 10.1140/epjp/i2018-11966-3
25. Gao W, Ghanbari B, Baskonus HM. New numerical simulations for some real world problems with Atangana-Baleanu fractional derivative. Chaos Solitons Fractals. (2019) 128:34–43. doi: 10.1016/j.chaos.2019.07.037
26. Atangana A, Koca I. Chaos in a simple nonlinear system with Atangana–Baleanu derivatives with fractional order. Chaos Solitons Fractals. (2016) 89:447–54. doi: 10.1016/j.chaos.2016.02.012
27. Abro KA, Mirbhar MN, Gómez-Aguilar JF. Functional application of Fourier sine transform in radiating gas flow with non-singular and non-local kernel. J Braz Soc Mech Sci Eng. (2019) 41:400. doi: 10.1007/s40430-019-1899-0
28. Abro KA, Khan I, Gómez-Aguilar JF. Thermal effects of magnetohydrodynamic micropolar fluid embedded in porous medium with Fourier sine transform technique. J Braz Soc Mech Sci Eng. (2019) 41:174. doi: 10.1007/s40430-019-1671-5
29. Abro KA, Gómez-Aguilar JF. A comparison of heat and mass transfer on a Walter's B fluid via Caputo-Fabrizio versus Atangana-Baleanu fractional derivatives using the Fox-H function. Eur Phys J Plus. (2019) 134:101. doi: 10.1140/epjp/i2019-12507-4
30. Gómez-Aguilar1 JF, Yépez-Martínez H, Torres-Jiménez J, Córdova-Fraga T, Escobar-Jiméne RF, Olivares-Peregrino VH. Homotopy perturbation transform method for nonlinear differential equations involving to fractional operator with exponential kernel. Adv Diff Equ. (2017) 2017:68. doi: 10.1186/s13662-017-1120-7
31. Yepez-Martineza H, Gomez-Aguilar JF, Sosaa IO, Reyesa JM, Torres-Jimenez J. The Feng's first integral method applied to the nonlinear mKdV space-time fractional partial differential equation. Rev Mex Fís. (2016) 62:310–6. doi: 10.1155/2016/7047126
32. Gómez-Aguilar JF, Torres L, Yépez-Martínez H, Baleanu D, Reyes JM, Sosa IO. Fractional Liénard type model of a pipeline within the fractional derivative without singular kernel. Adv Diff Equ. (2016) 2016:173. doi: 10.1186/s13662-016-0908-1
33. Morales-Delgado VF, Taneco-Hernández MA, Gómez-Aguilar JF. On the solutions of fractional order of evolution equations. Eur Phys J Plus. (2017) 132:47. doi: 10.1140/epjp/i2017-11341-0
34. Singh J, Kumar D, Baleanu D. New aspects of fractional Biswas-Milovic model with Mittag-Leffler law. Math Model Nat Phenom. (2019) 14:1–23. doi: 10.1051/mmnp/2018068
35. Bhatter S, Mathur A, Kumar D, Singh J. A new analysis of fractional Drinfeld–Sokolov–Wilson model with exponential memory. Phys A. (2020) 537:122578. doi: 10.1016/j.physa.2019.122578
36. Kumar D, Singh J, Baleanu D. On the analysis of vibration equation involving a fractional derivative with Mittag-Leffler law. Math Methods Appl Sci. (2019). doi: 10.1002/mma.5903. [Epub ahead of print].
37. Goswami A, Singh J, Kumar D, Sushila. An efficient analytical approach for fractional equal width equations describing hydro-magnetic waves in cold plasma. Phys A. (2020) 524:563–75. doi: 10.1016/j.physa.2019.04.058
38. Kumar D, Singh J, Qurashi MA, Baleanu D. A new fractional SIRS-SI malaria disease model with application of vaccines, anti-malarial drugs, and spraying. Adv Diff Equ. (2019) 2019:278. doi: 10.1186/s13662-019-2199-9
39. Veeresha P, Prakasha DG, Kumar D. An efficient technique for nonlinear time-fractional Klein–Fock–Gordon equation. Appl Math Comput. (2020) 364:124637. doi: 10.1016/j.amc.2019.124637
40. Richards LA. Capillary conduction of liquids through porous medium. Physics. (1931) 1:318–33. doi: 10.1063/1.1745010
41. Brooks RH, Corey AT. Hydraulic Properties of Porous Media. Hydrol Paper 3. Fort Collins, CO: Colorado State University (1964).
42. Corey AT. Mechanics of Immiscible Fluids in Porous Media. Highlands Ranch, CO: Water Resource Publications (1994).
44. Basha HA. Burgers' equation: a general nonlinear solution of infiltration and redistribution. Water Resour Res. (2002) 38:1–9. doi: 10.1029/2001WR000954
45. Nasseri M, Shaghaghian MR, Daneshbod Y, Seyyedian H. An analytic solution of water transport in unsaturated porous media. J Porous Media. (2008) 11:591–601. doi: 10.1615/JPorMedia.v11.i6.60
46. Jafari H, Firoozjaee MA. Application of homotopy analysis method for water transport in unsaturated porous media. Stud Nonlinear Sci. (2010) 1:8–13.
47. Caputo M. Fabrizio M. A new definition of fractional derivative without singular kernel. Prog Fract Diff Appl. (2015) 1:73–85. doi: 10.12785/pfda/010201
48. Atangana A, Baleanu D. New fractional derivatives with non-local and non-singular kernel theory and application to heat transfer model. Therm Sci. (2016) 20:763–9. doi: 10.2298/TSCI160111018A
49. Liao SJ. Homotopy analysis method and its applications in mathematics. J Basic Sci Eng. (1997) 5:111–25.
50. Liao SJ. Homotopy analysis method: a new analytic method for nonlinear problems. Appl Math Mech. (1998) 19:957–62. doi: 10.1007/BF02457955
51. Singh J, Kumar D, Swroop R. Numerical solution of time- and space-fractional coupled Burgers' equations via homotopy algorithm. Alexandria Eng J. (2016) 55:1753–63. doi: 10.1016/j.aej.2016.03.028
52. Srivastava HM, Kumar D, Singh J. An efficient analytical technique for fractional model of vibration equation. Appl Math Model. (2017) 45:192–204. doi: 10.1016/j.apm.2016.12.008
53. Prakasha DG, Veeresha P, Baskonus HM. Two novel computational techniques for fractional Gardner and Cahn-Hilliard equations, Comp Math Methods. (2019) 1:1–19. doi: 10.1002/cmm4.1021
54. Bulut H, Kumar D, Singh J, Swroop R, Baskonus HM. Analytic study for a fractional model of HIV infection of CD4+T lymphocyte cells. Math Nat Sci. (2018) 2:33–43. doi: 10.22436/mns.02.01.04
55. Veeresha P, Prakasha DG. Solution for fractional Zakharov-Kuznetsov equations by using two reliable techniques. Chinese J Phys. (2019) 60:313–30. doi: 10.1016/j.cjph.2019.05.009
56. Kumar D, Agarwal RP, Singh J. A modified numerical scheme and convergence analysis for fractional model of Lienard's equation. J Comput Appl Math. (2018) 399:405–13. doi: 10.1016/j.cam.2017.03.011
57. Prakash A, Prakasha DG, Veeresha P. A reliable algorithm for time-fractional Navier-Stokes equations via Laplace transform. Nonlinear Eng. (2019). 8:695–701. doi: 10.1515/nleng-2018-0080
58. Veeresha P, Prakasha DG, Baskonus HM. Novel simulations to the time-fractional Fisher's equation. Math Sci. (2019) 13:33–42. doi: 10.1007/s40096-019-0276-6
59. Prakash A, Veeresha P, Prakasha DG, Goyal M. A homotopy technique for fractional order multi-dimensional telegraph equation via Laplace transform. Eur Phys J Plus. (2019) 134:19. doi: 10.1140/epjp/i2019-12411-y
60. Singh J, Kumar D, Baleanu D, Rathore S. An efficient numerical algorithm for the fractional Drinfeld–Sokolov–Wilson equation. Appl Math Comput. (2018) 335:12–24. doi: 10.1016/j.amc.2018.04.025
61. Parlang J-Y, Barry DA, Parlange MB, Hogarth WL, Haverkamp R, Ross PJ, et al. New approximate analytical technique to solve Richards equation for arbitrary surface boundary conditions. Water Resour Res. (1997) 33:903–6. doi: 10.1029/96WR03846
62. Parlang J-Y, Hogarth WL, Barry DA, Parlange MB, Haverkamp R, Ross PJ, et al. Analytical approximation to the solutions of Richards' equation with applications to infiltration, ponding, and time compression approximation. Adv Water Resour. (1999) 23:189–94. doi: 10.1016/S0309-1708(99)00022-6
63. Atangana A, Alkahtani BT. Analysis of the Keller-Segel model with a fractional derivative without singular kernel. Entropy. (2015) 17:4439–53. doi: 10.3390/e17064439
64. Alkahtani BT, Atangana A. Analysis of non-homogenous heat model with new trend of derivative with fractional order. Chaos Solitons Fractals. (2016) 89:566–71. doi: 10.1016/j.chaos.2016.03.027
65. Singh J, Kumar D, Swroop R, Kumar S. An Efficient computational approach for time-fractional Rosenau-Hyman equation. Neural Comput Appl. (2018) 30:3063–70. doi: 10.1007/s00521-017-2909-8
Keywords: Laplace transform, Atangana-Baleanu derivative, Richards equation, q-homotopy analysis method, fixed point theorem
Citation: Prakasha DG, Veeresha P and Singh J (2019) Fractional Approach for Equation Describing the Water Transport in Unsaturated Porous Media With Mittag-Leffler Kernel. Front. Phys. 7:193. doi: 10.3389/fphy.2019.00193
Received: 18 October 2019; Accepted: 05 November 2019;
Published: 04 December 2019.
Edited by:
Devendra Kumar, University of Rajasthan, IndiaReviewed by:
Haci Mehmet Baskonus, Harran University, TurkeyFrancisco Gomez, Centro Nacional de Investigación y Desarrollo Tecnológico, Mexico
Copyright © 2019 Prakasha, Veeresha and Singh. This is an open-access article distributed under the terms of the Creative Commons Attribution License (CC BY). The use, distribution or reproduction in other forums is permitted, provided the original author(s) and the copyright owner(s) are credited and that the original publication in this journal is cited, in accordance with accepted academic practice. No use, distribution or reproduction is permitted which does not comply with these terms.
*Correspondence: Jagdev Singh, amFnZGV2c2luZ2hyYXRob3JlQGdtYWlsLmNvbQ==
 D. G. Prakasha1
D. G. Prakasha1 P. Veeresha
P. Veeresha Jagdev Singh
Jagdev Singh